Linked: strength in weakness?
“How many handshakes?” Do we all know about networking? And when weak links are strong?
And really, what do we know about networks? Many people already know, although with a close look at this subject, not everything is so simple. Almost everyone knows about the strange fact of the "six handshakes." Despite the fact that this property of networks can be proved with mathematical (statistical) accuracy, it can be difficult to understand and there will always be a lot of doubters. See, for example, the interesting discussions of this subject under the question mark “myth or scientific truth?” Or in the other “Six handshakes are replaced by three”.
 What is now known in the science of the real structure of networks does not fit in with the usual ideas about networks. And therefore come across funny delusions, from time to time occurring in forums and blogs. For example, many believe that the essence of the law lies in the magic number, which is not exactly known (about 6 or 7 handshakes), but this number exists and even seems to be (almost) fixed for any networks. Of course, the number itself has nothing to do with, it can vary for different real networks and is quite noticeable. Moreover, there are examples of networks where the rule of six handshakes does not work at all and a similar network model will be presented here below. In fact, all that the rule of six handshakes says is that the world is small and its size in handshakes is almost always negligible compared to the size of the network, calculated from the number of nodes or links. As a rule, all spontaneously formed social networks nevertheless fulfill the rule of six (three, ten, etc. in different networks in different ways) handshakes. But in the field of transport networks it is easy to find well-known examples of the network where this rule is not satisfied. This is a common road network. It is clear that we consider not handshakes, but crossroads, turns or settlements traveled on the way from point A to point B. I will not prove it in detail here, it seems that it is not difficult to understand simply by opening and analyzing any map. By the way, in the air traffic network the rule of “six handshakes” (transfers or transits) is fulfilled. In general, the number of “handshakes” for each network may depend on the size of the network, the statistical distribution of connections between nodes, the degree of clustering, and some other not so noticeable factors.
What is now known in the science of the real structure of networks does not fit in with the usual ideas about networks. And therefore come across funny delusions, from time to time occurring in forums and blogs. For example, many believe that the essence of the law lies in the magic number, which is not exactly known (about 6 or 7 handshakes), but this number exists and even seems to be (almost) fixed for any networks. Of course, the number itself has nothing to do with, it can vary for different real networks and is quite noticeable. Moreover, there are examples of networks where the rule of six handshakes does not work at all and a similar network model will be presented here below. In fact, all that the rule of six handshakes says is that the world is small and its size in handshakes is almost always negligible compared to the size of the network, calculated from the number of nodes or links. As a rule, all spontaneously formed social networks nevertheless fulfill the rule of six (three, ten, etc. in different networks in different ways) handshakes. But in the field of transport networks it is easy to find well-known examples of the network where this rule is not satisfied. This is a common road network. It is clear that we consider not handshakes, but crossroads, turns or settlements traveled on the way from point A to point B. I will not prove it in detail here, it seems that it is not difficult to understand simply by opening and analyzing any map. By the way, in the air traffic network the rule of “six handshakes” (transfers or transits) is fulfilled. In general, the number of “handshakes” for each network may depend on the size of the network, the statistical distribution of connections between nodes, the degree of clustering, and some other not so noticeable factors.
I understand that in a small article it is difficult to present something in a fully demonstrative way, and any comment that refutes it all may look no less convincing. Nevertheless, everything I write about here is based on scientific sources. Check it for yourself - I will give a link to one book that is written by a mathematician specializing in this particular field of study: Barabasi, Albert-Laszlo (2003), Linked on - Life , Plume, 2003. I strongly advise you to read to those who are interested (unfortunately, not many serious books on the topic have already been translated into Russian). Actually, some of the considerations presented here about the networks are taken (among others) from this book.
')
You can prove the fact of the “six handshakes” just mathematically, but there is another way to explain the effect of a tight world, as a result of which one more interesting property of networks becomes clear, called the strength of weak connections. First, what are weak links in themselves, and then why they are both so strong at the same time (the famous question is “what is the strength, brother?” :))
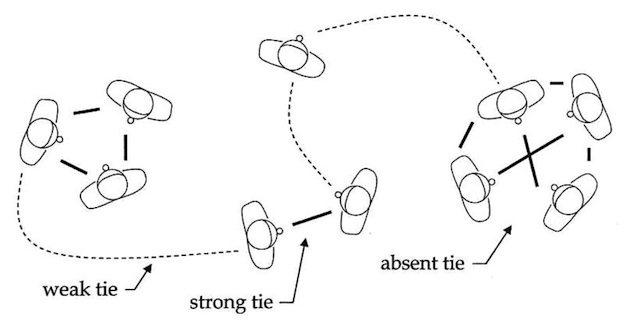
The easiest way to understand what weak links are is by a simple example of a personal contact sheet. Let's try to divide all your contacts into close friends and acquaintances on the one hand and all the others on the other. It is intuitive to everyone what is the difference between the two resulting contact lists. But we are only interested in one of these differences, which is directly related not to the fact of personal closeness, but to the structure of the network itself. Let's analyze the connections of friends both with me and with each other. As a rule, all my friends are acquainted not only with me, but also among themselves as well. And indeed, due to frequent contacts, we usually manage to introduce our friends to each other, even if initially some of them were not familiar with each other. Usually also people working together (in the same department) are strongly connected. Sociologists have called such connections strong since the 1970s. But all the links from the second part of the list are called weak. If I rarely communicate with a person, then most likely, if he knows someone from my friends (or, in general, all my acquaintances), then by no means all. If we take and analyze all contacts from the second list, then the density of connections among such people (the ratio [number of connections] / [number of nodes] in this group) will be an order of magnitude less than in the first list. The calculation of such relations and their distribution gives us one way to measure clustering of a network of graphs. And from the point of view of cluster analysis, it turns out that strong links are those that are located inside one of the clusters of our network, and weak links are those that link different clusters to each other.
Another characteristic by which we can distinguish two types of bonds is the frequency of contacts, which is an order of magnitude greater in the case of strong bonds. Of course, we communicate with friends, relatives and co-workers much more often than with former classmates or with acquaintances of a scientific or business conference (there are quite a few business cards of such acquaintances in our business card holders). Well, what is so surprising here and is there at least some benefit from such differences? Yes, in general, nothing surprising, if you do not go further into the study of the device networks and do not deal with them further. Let's still try to move on.
First, it is weak links, unlike strong ones, that are the thin strings that make our world so close. They bring us together to a few handshakes. Why is this so consider a simple network model (see figure)

In the extreme left figure, only strong bonds are present. And in this model it is easy to see that the rule of 6 handshakes is not fulfilled. If we take it exactly, between the opposite nodes exactly (for the meticulous ones, - up to the divisor) N / 4 handshakes. And with the growth of N (the total number of nodes of this model), the average number of handshakes increases in proportion to N. In the second figure, you can see how even a small number of weak links drastically reduces the distance of the nodes from each other. Only one such connection can reduce the distance between the most distant nodes by 2 times. For large N, the number of weak links (we denote it by m), which is enough to reduce the entire network to six handshakes, is surprisingly small. Those. as N → ∞, m / N → 0, - which is the rule of six handshakes or a tight world. Of course, this fact about the role of weak ties for our close world is so intuitive. How many conversations about adventurers, loners and travelers, who like strings connect us with the wild tribes of Africa or Amazonia. Here I tried to imagine how this fact can be calculated mathematically on a model. Construct the appropriate formulas for the model in the figure any high school graduate can do himself, although more serious models and even more so real networks require more serious mathematics.
The second surprising (weird) property of weak links is connected with the conditions of information distribution in the network. Obviously, through strong connections (i.e. within individual clusters), information spreads very quickly. And this is wonderful; the actual exchange of information is what social connections exist for. But there are also cons, imperceptible without additional analysis. The effect of such an intensity of data exchange leads to such a state of affairs that any information known to one of the participants in a particular cluster is likely to be known to the rest of its participants. For example, information about a vacancy in a department becomes known to all employees of a department, and outside the department, and especially firms, not many of those familiar with these departments have such information. Now it is clear that this fact leads to problems when it is necessary to search for absolutely new information. Imagine that we are looking for work through friends. It is almost useless to ask my close friends, as well as colleagues, since their knowledge is hardly different from what I know. Thus, if we really need new information, we should use our "weak" contacts (that is, those from the second part of our contact list). Of course, we cannot talk about strict patterns, but only about trends and greater likelihood. This is only a relative effect. After all, we cannot say that all people from our inner circle have a completely identical set of knowledge. For those interested, I will give a link to a real sociological study of this fact: The strength of weak ties .
What other benefits can be gained in everyday life or in work from this knowledge of network structure? Let's just say, to promote a site on the network, it would be nice to sort out and separate two types of links and focus on the part that belongs to the category of the weak. Due to these connections, we can use their potential to more widely dissolve our tentacles. There is another interesting topic. Marketers regularly talk about the benefits of segmentation and the choice of target segments. I read marketing books where it is stated and tried to understand the logic presented there, but I never found it convincing. Although from the experience of my work, I saw that correct segmentation in reality operates here and there. I am a techie and I love clear logic and consistent evidence of any known fact and so far I don’t have a clear and complete understanding of what exactly happens when we purposefully narrow down the target market and how it increases marketing effectiveness. But I still have some thoughts. My hypothesis is such that in a correctly chosen target segment there is an increased degree of clusterization and density of bonds and due to this, the viral effect is the most efficient. I will be happy to hear if someone presents their ideas why target segmentation is more efficient than marketing in the entire market (preferably in the network sense, it is clear that there are simply economic considerations of the type — limited resources are used more efficiently, etc.)
And really, what do we know about networks? Many people already know, although with a close look at this subject, not everything is so simple. Almost everyone knows about the strange fact of the "six handshakes." Despite the fact that this property of networks can be proved with mathematical (statistical) accuracy, it can be difficult to understand and there will always be a lot of doubters. See, for example, the interesting discussions of this subject under the question mark “myth or scientific truth?” Or in the other “Six handshakes are replaced by three”.
 What is now known in the science of the real structure of networks does not fit in with the usual ideas about networks. And therefore come across funny delusions, from time to time occurring in forums and blogs. For example, many believe that the essence of the law lies in the magic number, which is not exactly known (about 6 or 7 handshakes), but this number exists and even seems to be (almost) fixed for any networks. Of course, the number itself has nothing to do with, it can vary for different real networks and is quite noticeable. Moreover, there are examples of networks where the rule of six handshakes does not work at all and a similar network model will be presented here below. In fact, all that the rule of six handshakes says is that the world is small and its size in handshakes is almost always negligible compared to the size of the network, calculated from the number of nodes or links. As a rule, all spontaneously formed social networks nevertheless fulfill the rule of six (three, ten, etc. in different networks in different ways) handshakes. But in the field of transport networks it is easy to find well-known examples of the network where this rule is not satisfied. This is a common road network. It is clear that we consider not handshakes, but crossroads, turns or settlements traveled on the way from point A to point B. I will not prove it in detail here, it seems that it is not difficult to understand simply by opening and analyzing any map. By the way, in the air traffic network the rule of “six handshakes” (transfers or transits) is fulfilled. In general, the number of “handshakes” for each network may depend on the size of the network, the statistical distribution of connections between nodes, the degree of clustering, and some other not so noticeable factors.
What is now known in the science of the real structure of networks does not fit in with the usual ideas about networks. And therefore come across funny delusions, from time to time occurring in forums and blogs. For example, many believe that the essence of the law lies in the magic number, which is not exactly known (about 6 or 7 handshakes), but this number exists and even seems to be (almost) fixed for any networks. Of course, the number itself has nothing to do with, it can vary for different real networks and is quite noticeable. Moreover, there are examples of networks where the rule of six handshakes does not work at all and a similar network model will be presented here below. In fact, all that the rule of six handshakes says is that the world is small and its size in handshakes is almost always negligible compared to the size of the network, calculated from the number of nodes or links. As a rule, all spontaneously formed social networks nevertheless fulfill the rule of six (three, ten, etc. in different networks in different ways) handshakes. But in the field of transport networks it is easy to find well-known examples of the network where this rule is not satisfied. This is a common road network. It is clear that we consider not handshakes, but crossroads, turns or settlements traveled on the way from point A to point B. I will not prove it in detail here, it seems that it is not difficult to understand simply by opening and analyzing any map. By the way, in the air traffic network the rule of “six handshakes” (transfers or transits) is fulfilled. In general, the number of “handshakes” for each network may depend on the size of the network, the statistical distribution of connections between nodes, the degree of clustering, and some other not so noticeable factors.I understand that in a small article it is difficult to present something in a fully demonstrative way, and any comment that refutes it all may look no less convincing. Nevertheless, everything I write about here is based on scientific sources. Check it for yourself - I will give a link to one book that is written by a mathematician specializing in this particular field of study: Barabasi, Albert-Laszlo (2003), Linked on - Life , Plume, 2003. I strongly advise you to read to those who are interested (unfortunately, not many serious books on the topic have already been translated into Russian). Actually, some of the considerations presented here about the networks are taken (among others) from this book.
')
You can prove the fact of the “six handshakes” just mathematically, but there is another way to explain the effect of a tight world, as a result of which one more interesting property of networks becomes clear, called the strength of weak connections. First, what are weak links in themselves, and then why they are both so strong at the same time (the famous question is “what is the strength, brother?” :))

The easiest way to understand what weak links are is by a simple example of a personal contact sheet. Let's try to divide all your contacts into close friends and acquaintances on the one hand and all the others on the other. It is intuitive to everyone what is the difference between the two resulting contact lists. But we are only interested in one of these differences, which is directly related not to the fact of personal closeness, but to the structure of the network itself. Let's analyze the connections of friends both with me and with each other. As a rule, all my friends are acquainted not only with me, but also among themselves as well. And indeed, due to frequent contacts, we usually manage to introduce our friends to each other, even if initially some of them were not familiar with each other. Usually also people working together (in the same department) are strongly connected. Sociologists have called such connections strong since the 1970s. But all the links from the second part of the list are called weak. If I rarely communicate with a person, then most likely, if he knows someone from my friends (or, in general, all my acquaintances), then by no means all. If we take and analyze all contacts from the second list, then the density of connections among such people (the ratio [number of connections] / [number of nodes] in this group) will be an order of magnitude less than in the first list. The calculation of such relations and their distribution gives us one way to measure clustering of a network of graphs. And from the point of view of cluster analysis, it turns out that strong links are those that are located inside one of the clusters of our network, and weak links are those that link different clusters to each other.
Another characteristic by which we can distinguish two types of bonds is the frequency of contacts, which is an order of magnitude greater in the case of strong bonds. Of course, we communicate with friends, relatives and co-workers much more often than with former classmates or with acquaintances of a scientific or business conference (there are quite a few business cards of such acquaintances in our business card holders). Well, what is so surprising here and is there at least some benefit from such differences? Yes, in general, nothing surprising, if you do not go further into the study of the device networks and do not deal with them further. Let's still try to move on.
First, it is weak links, unlike strong ones, that are the thin strings that make our world so close. They bring us together to a few handshakes. Why is this so consider a simple network model (see figure)

In the extreme left figure, only strong bonds are present. And in this model it is easy to see that the rule of 6 handshakes is not fulfilled. If we take it exactly, between the opposite nodes exactly (for the meticulous ones, - up to the divisor) N / 4 handshakes. And with the growth of N (the total number of nodes of this model), the average number of handshakes increases in proportion to N. In the second figure, you can see how even a small number of weak links drastically reduces the distance of the nodes from each other. Only one such connection can reduce the distance between the most distant nodes by 2 times. For large N, the number of weak links (we denote it by m), which is enough to reduce the entire network to six handshakes, is surprisingly small. Those. as N → ∞, m / N → 0, - which is the rule of six handshakes or a tight world. Of course, this fact about the role of weak ties for our close world is so intuitive. How many conversations about adventurers, loners and travelers, who like strings connect us with the wild tribes of Africa or Amazonia. Here I tried to imagine how this fact can be calculated mathematically on a model. Construct the appropriate formulas for the model in the figure any high school graduate can do himself, although more serious models and even more so real networks require more serious mathematics.
The second surprising (weird) property of weak links is connected with the conditions of information distribution in the network. Obviously, through strong connections (i.e. within individual clusters), information spreads very quickly. And this is wonderful; the actual exchange of information is what social connections exist for. But there are also cons, imperceptible without additional analysis. The effect of such an intensity of data exchange leads to such a state of affairs that any information known to one of the participants in a particular cluster is likely to be known to the rest of its participants. For example, information about a vacancy in a department becomes known to all employees of a department, and outside the department, and especially firms, not many of those familiar with these departments have such information. Now it is clear that this fact leads to problems when it is necessary to search for absolutely new information. Imagine that we are looking for work through friends. It is almost useless to ask my close friends, as well as colleagues, since their knowledge is hardly different from what I know. Thus, if we really need new information, we should use our "weak" contacts (that is, those from the second part of our contact list). Of course, we cannot talk about strict patterns, but only about trends and greater likelihood. This is only a relative effect. After all, we cannot say that all people from our inner circle have a completely identical set of knowledge. For those interested, I will give a link to a real sociological study of this fact: The strength of weak ties .
What other benefits can be gained in everyday life or in work from this knowledge of network structure? Let's just say, to promote a site on the network, it would be nice to sort out and separate two types of links and focus on the part that belongs to the category of the weak. Due to these connections, we can use their potential to more widely dissolve our tentacles. There is another interesting topic. Marketers regularly talk about the benefits of segmentation and the choice of target segments. I read marketing books where it is stated and tried to understand the logic presented there, but I never found it convincing. Although from the experience of my work, I saw that correct segmentation in reality operates here and there. I am a techie and I love clear logic and consistent evidence of any known fact and so far I don’t have a clear and complete understanding of what exactly happens when we purposefully narrow down the target market and how it increases marketing effectiveness. But I still have some thoughts. My hypothesis is such that in a correctly chosen target segment there is an increased degree of clusterization and density of bonds and due to this, the viral effect is the most efficient. I will be happy to hear if someone presents their ideas why target segmentation is more efficient than marketing in the entire market (preferably in the network sense, it is clear that there are simply economic considerations of the type — limited resources are used more efficiently, etc.)
Source: https://habr.com/ru/post/95278/
All Articles