Maths. Symmetry of "pseudo-simple twins"
At leisure, he studied the properties of prime numbers and revealed one possibly interesting pattern. Looked on the web, in my opinion, this question has not been raised before.
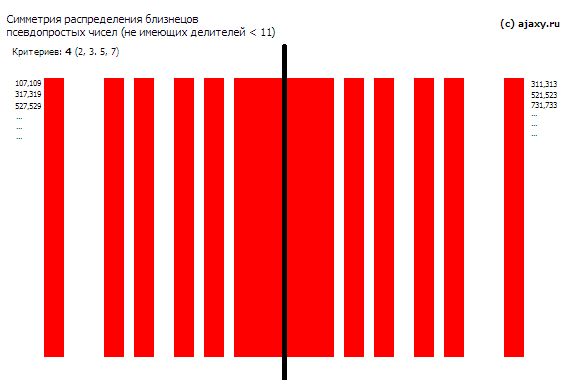
It is known that primes in nature are distributed in an unknown way, and there are no functions that reflect the pattern of sequence. In the theory, there are also “ simple twins ” - pairs of prime numbers that differ by 2 (for example - 7, 11, 13 , 17, 23, 29, 31 ).
The effect that is visible in the image above is observed when creating a series of pseudo-simple numbers. Psevdoprosnymi we will call such numbers that do not have divisors from a limited set of possible. For example, if you take several numbers in a row without divisors from the first 4 prime numbers (2, 3, 5, 7) and select “twins” from them, an interesting picture is observed: the order of twins repeats cyclically, each cycle consisting of two mirror parts. Here is a numerical example .
')
When adding the following simple divider as a criterion - 11 , the picture changes greatly, the cycle length increases from 313 to 2309, but the symmetry remains:

Example with numbers .
Despite the fact that at the addition of 13 to the filter dividers, the cycle length increased to ~ 30,000 , I managed to find its boundary and display symmetry. However, gd2 refuses to correctly draw such a long picture, use the zoom out in the browser to better consider the numeric example number 3 (the axis of symmetry passes between 15013 and 15017 ). And you have to wait a bit while the script checks 60000 numbers.
In addition to graphical symmetry, all textual examples clearly show the symmetry of numbers in numbers, which theoretically makes it easy to build such tables mechanically, without factorizing numbers.
All this suggests the idea that, if we push the number of criteria (divisors) to ∞, then similar effects can theoretically be found for genuinely prime numbers, and this could be the discovery of the millennium :-p
In any case, it is interesting to hear your opinion on the possible explanation of such behavior of numbers and, perhaps, some thoughts for the further development of the idea on this matter.
Have a nice day, everyone! :)

It is known that primes in nature are distributed in an unknown way, and there are no functions that reflect the pattern of sequence. In the theory, there are also “ simple twins ” - pairs of prime numbers that differ by 2 (for example - 7, 11, 13 , 17, 23, 29, 31 ).
The effect that is visible in the image above is observed when creating a series of pseudo-simple numbers. Psevdoprosnymi we will call such numbers that do not have divisors from a limited set of possible. For example, if you take several numbers in a row without divisors from the first 4 prime numbers (2, 3, 5, 7) and select “twins” from them, an interesting picture is observed: the order of twins repeats cyclically, each cycle consisting of two mirror parts. Here is a numerical example .
')
When adding the following simple divider as a criterion - 11 , the picture changes greatly, the cycle length increases from 313 to 2309, but the symmetry remains:

Example with numbers .
Despite the fact that at the addition of 13 to the filter dividers, the cycle length increased to ~ 30,000 , I managed to find its boundary and display symmetry. However, gd2 refuses to correctly draw such a long picture, use the zoom out in the browser to better consider the numeric example number 3 (the axis of symmetry passes between 15013 and 15017 ). And you have to wait a bit while the script checks 60000 numbers.
In addition to graphical symmetry, all textual examples clearly show the symmetry of numbers in numbers, which theoretically makes it easy to build such tables mechanically, without factorizing numbers.
All this suggests the idea that, if we push the number of criteria (divisors) to ∞, then similar effects can theoretically be found for genuinely prime numbers, and this could be the discovery of the millennium :-p
In any case, it is interesting to hear your opinion on the possible explanation of such behavior of numbers and, perhaps, some thoughts for the further development of the idea on this matter.
Have a nice day, everyone! :)
Source: https://habr.com/ru/post/88524/
All Articles