Model of the natural series of numbers and its elements. Diamonds

In this work, the basic G 2 ± model is preserved, but another organization of its cells is adopted (another figure). On top of the primary lattice with cells of size 1 × 1 , a larger grid is depicted - a grid of rhombuses, and a grid of centers of rhombuses (SCR) is also considered. The last grid is not depicted, so as not to overload the lines with diamonds. We will not repeat the definitions and concepts that have been set forth in detail in previous works , but we will give references pointing to these works.
Constructive description of the model
Through cells G 2 ± - models contained in even long D i and short K i diagonals with numbers terminating in zeros within G 2 - - submodels, lines are drawn that form a large grid of rhombuses in the plane. The areas of the rhombus for the cells together cover the entire plane without breaks. Each rhombus contains 41 cells, of which only 16 are of interest, and only 4 cells with fixed flexion are used for sounding the rhombus.
The characteristics of the rhombus will include:
')
- the number of cells in a diamond;
- the value of the number in the central cell;
- numbers of its horizontal ( H i ) and vertical ( V i );
- identifying cells for numbers with flakes 1, 3, 7, 9;
- coordinates of these cells in the coordinate system of a rhombus with the beginning in the central cell of the rhombus.
Through the cells of the centers of rhombuses, a grid of centers of rhombuses is also constructed, the nodes of which are located in the cells at the intersection of long and short diagonals with multiples of 5.
From the picture with rhombuses it is clear which grid we are talking about. To illustrate the features of rhombuses, images of a pair of rhombuses in each half-plane are provided. These rhombuses are provided with markup identifying numbers of cells in the bottom and another pair of such rhombus in the upper half-planes. The marking of rhombuses in the half-plane below the diagonal D 0 differs from the marking of rhombuses in the half-plane above it, but within one half-plane the marking of all rhombuses is identical for both the lower and upper half-planes. The essence of the markup is the localization of cells with numbers that have equal flexions (marked by filling the cells with the same color), setting their coordinates x 1 , x 0 . The designated rhombuses will be called fundamental, from them other rhombuses can be formed with changing the image scale.
The centers of the lower half-rhombus are cells with numbers ending in two digits, either 25 with a horizontal number with flexion 5 and with a vertical number with flexion 0, or 75 with a horizontal number with flexion 0 and with vertical number with flexion 5. In the upper half-plane G 2 + - the submodels all numbers in the central cells of all rhombuses end in two figures 25. Further, we restrict consideration to the half-plane G 2 - .
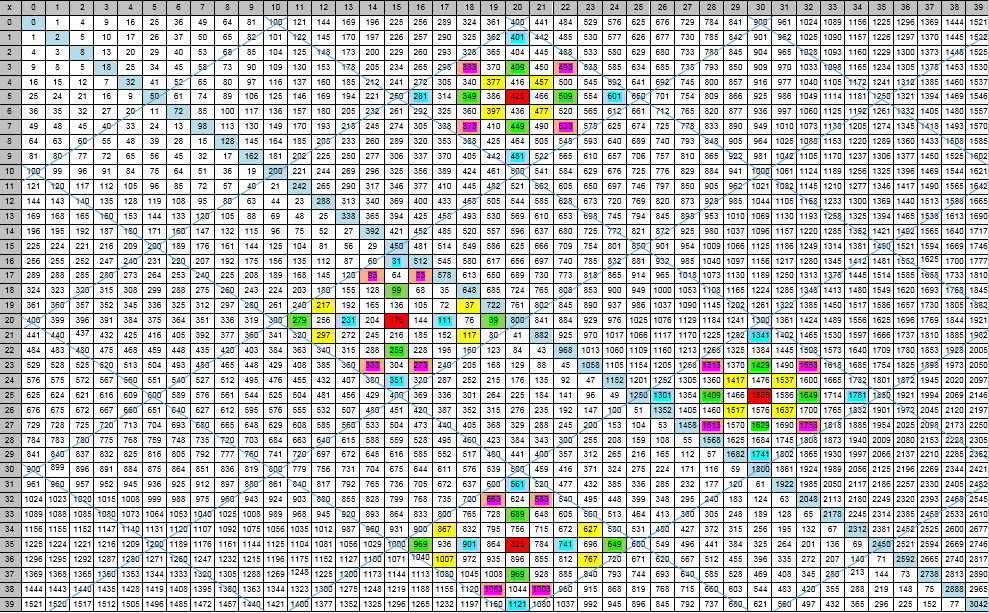
Figure 1— Visual representation of the diamond model
Definition 1. A fundamental rhombus is the structure of Γ 2 ± - a model bounded by two short and two long diagonals of this plane with numbers that are multiples of 10. The main element characterizing a rhombus is the cell ( x 1c , x 0c ) of its center. The center contains a numerical value of N, a multiple of 5.
Definition 2. The set of centers of fundamental rhombuses is the nodes (cells) of the rhombus grid (CCR) of intersecting short and long diagonals, with numbers multiple of 5. The rhombi themselves completely cover the plane G 2 ± - models (parquet principle).
All diamonds are arranged in the same type, and the numbers in their cells with fixed endings are placed in fixed positions (cells). This makes it easy to factor this number when solving the problem of localizing the number N in a certain diamond. The ten horizontals whose cells form a rhombus and the rhombus adjacent to it (with coordinates different from it) are called the rhombus strip. Horizontal bands are considered: West-East (ZV); vertical: North-South (SY), along short diagonals: Northeast (NE) and along long diagonals: North-West (NW). The displacement from one rhombus to another can, in addition to specifying a band, be supplemented by an indication (up-down) along the bands mentioned.
Since definition 2 implies that the totality of cells of all rhombuses are all G 2 ± - model cells, one of the cells (x 1p , x 0p ) belonging to a certain rhombus necessarily contains a given composite odd natural number (SNF) N ( x 1p , x 0p ) = N (x 1 , x 0 ) . At the same time, we believe that it is possible to indicate such a rhombus (determining the coordinates of its central cell) (x 1c , x 0c ) , rather than probing all the cells, even limiting ourselves to probing only odd diagonals.
The indication of such a rhombus and the desired cell within it is a solution to the localization problem for a given number N (x 1 , x 0 ) . This task and its solution precede the receipt of the SFChB solution. The meaning and ultimate goal of the localization problem is to indicate in a certain diamond for a given SNF N (x 1 , x 0 ) the coordinates of the cell (x 1p , x 0p ) in G 2 ± - the model in which there is a number that coincides with N (x 1 , x 0 ) .
In this article, we use the mechanism of establishing the belonging of a given number N to a particular rhombus and a cell in it. This mechanism is far from the best, but in the proposed examples copes with the task. Readers are invited to either offer their original, or improve this mechanism.
Our mechanism is based on the remarkable regularity of the G 2 ± open model — the presence of horizontals with multiples of five (and some others), cells with squares of Pythagorean triplets (PFT) < g, k 1 , k 2 > = <hypotenuse , leg 1 , leg 2 >. About the CFT it will be discussed in another work.
To simplify the conclusions and calculations, we need three coordinate systems: a planar one that has already been entered , a network with different diagonal numbers for the SCR (Fig. 2) and a rhombic one (Table 1), in which the beginning is associated with the rhomb center cell.

Figure 2. Numbering (double) short diagonals and
distribution of diamond centers on short diagonals
Table 1. Determination of the coordinates of the search point within a fixed rhombus

In the system of SCR, the following numbers are indicated: the number of the short diagonal n , is the ordinal number of the center on it, as well as the network-wide C number of the center, the own numbering of short diagonals is performed, starting from n = 1 , then the number n p = 2 (this is the number increased by 10, i.e. the 15th planar K i ) and then, in increments of 10, all the rest. The position of all cells of the centers of rhombuses in each K i CCR is also numbered from q = 1 to q = 2n p of the doubled network number of the short diagonal.
Example 1 Suppose you want to find the network-wide number C of the center of one of the rhombuses and the number N in this cell for a given short diagonal passing through the centers of the rhombus, its network number n p = 5 , and the sequence number of the center u = 3 of one of the rhombuses on it. The network coordinates of the cell of the center of this rhombus are represented simply in the form (n p , c) = (5, 3) .
- Find the plane x 1 coordinate of the cell of the beginning of a given diagonal (nd):
x 1 = x nd = 10n p - 5 = 50 - 5 = 45.
For our case, we got x 1 = x nd = 45 . - Now we can go straight to the search for the plane coordinates of the cell ( x 1c , x ots ) of the required center: x 1 = x nd - 5 (c - 1) = 45 - 5 (3 - 1) = 35, x 0 = 0 + 5 ( q - 1) = 2 ∙ 5 = 10.
- Find the network number of the center of the rhombus ( C ).
Comment. It is known that for a number x the formula 2C x + 1 2 = x (x + 1) is the double number of combinations of x + 1 , two by two.
The number of centers preceding the short diagonal n p = 5 is 2n p (n p - 1) . Then the sequence number of the network center is given by the formula
C = n p (n p -1) + c = 2C n p 2 + 3 = 5 ∙ 4 + 3 = 23 . - Find the value of the number N (x 1c , x ots ) in the center cell of the rhombus N = x 1 2 - x 0 2 - the sign in the formula is taken depending on the center position relative to the main diagonal.
N = 35 2 - 10 2 = 1125 - for our case.
Thus, having only the network number n p of the short diagonal passing through the center cells and the current center number of the diamond on this diagonal, all other information about the center of the diamond can be obtained.
All odd positive integers of interest N belong to the cells of the rhombus. The concept of flexion - the last digit of a number - allows localizing their position within a diamond. For factorization, those N numbers that end in the numbers 1, 3, 7, 9 are of interest.
Even numbers as N are not considered, since they have a simple divisor 2. Numbers ending with a five, have a simple divisor 5, which is also unacceptable for N. Localization of a specific N along the flexion inside a rhombus is advisable to be carried out relative to the center of the rhombus, in the context that the center is the most important characteristic of the rhombus. Based on the fact that all rhombuses have the same structure, there is a clear relationship between the number N given for factorization and c numbers in rhombus cells with certain flexions and in the rhombus center cell. Data on such interrelations of numbers are given in table. one.
However, sorting all the rhombuses on the plane to find the desired rhombus is unacceptable neither in time nor in computing costs. Thus, the problem arose of localizing the regions of the G 2 - - submodel (half-plane), including such rhombuses, which would contain the initial number N to be factorized.
Pythagorean triples . To solve the formulated problem are used
Pythagorean triples are triples of numbers satisfying the Pythagorean theorem: Namely, Pythagorean triples that satisfy the rule of the so-called Egyptian triangle, i.e., a triangle with sides that are multiple to 3, 4, 5.
In each horizontal x 1 containing the centers of rhombuses, there is one or more such Pythagorean triples.
The first rhombus in the localization problem is indicated approximately and, with a “miss,” the following rhombuses should be chosen. To do this, it is necessary to determine the direction of movement along the CCR, so as to gradually approach the final goal. For example, if the smaller of the 4 numbers in the current rhombus is less than the given N, then the Northeastern and Eastern diamonds from it contain even smaller 4 numbers of numbers, i.e., such rhombuses should not be probed. Moving to the West rhombus leads to such an increase in values in all 4 of its cells, that even a smaller number of the West rhombus turns out to be more than the previous rhombus and, therefore, more N. Hence the solution: to move from rhombus to rhomb upwards in the NW direction.
If a rhombus containing a cell with a number equal to N (x 1p , x 0p ) = N is found and the coordinates of the cell (x 1p , x 0p ) are determined, then the decision of the RSPCS is determined by the basic relation G 2 ± - models
N = x 2 1 - x 2 0 = (x 1 - x 0 ) (x 1 + x 0 ) = p ∙ q
Another subtask is the selection and implementation of a sequence of cell traversal of a diamond selected for sensing. Here, the counterclockwise order is taken, starting with the left upper cell containing the number with the desired flexion. In the situation of coincidence of values in the rhombus cell N (x 1p , x 0p ) and a given number N (x 1 , x 0 ), the difference between them turns out to be zero.
Algorithm solution ZFBCH using fundamental diamonds and CFT
- Extract the root from the number N. Round down.
- Check if √N is divisible by 3. If divisible, then assign this value to the first leg k1, otherwise, to ensure that the divisibility property is 3, subtract from result 1 or 2, and store it as k1. The result of dividing an entirely chosen value into three M = √N / 3 is the scaling factor of the CFT.
- We obtain the value for the second leg k 2 , according to the rule of the Egyptian triangle, k 2 = 4 ∙ M.
- Find the value of the hypotenuse g = 5 ∙ M , and the value x 1 = g should be divided by the number five. As you can see, the value of the hypotenuse is always equal to the horizontal number of the CFT.
- Find the coordinate x 1 = g .
- After that, we determine the inflection (last digit) of the number N , f = N (mod10) .
- We find the center of the rhombus closest to k1 and then examine the adjacent rhombuses in one of the rhombus lanes (there are 4 directions) to find a solution.
- Depending on which inflection we get in point 6, we use the required column (mask) from those presented in table. 1 to determine the coordinates of the search point ( x 1p , x 0p ) and find the value of the number in this cell N p . In each rhombus, only 4 cells from 41 cells are checked.
- After establishing the affiliation of the number N to a certain rhombus and a cell in it, based on the table. 1, we obtain the plane coordinates N: (x 1p , x 0p ) .
- Using the properties of the selected mathematical model
N = x 2 1 - x 2 0 = (x 1 - x 0 ) (x 1 + x 0 ) = p ∙ q
we obtain the multiplicative representation of N from the additive one. - Thus, at the output of the algorithm, we have: N = p ∙ q . Depending on the value of the flexion f according to the formulas in the table. 1, the coordinates of the point (x 1p , x 0p ) are determined and the value of the difference ∆ = N (x 1p , x 0p ) - N (x 1 , x 0 ) is calculated. If ∆ ≠ 0 , then the transition to another cell, if all the cells of the diamond are checked, then to another diamond.
If ∆ = 0 , then x 1 = x 1p , x 0 = x 0p and p = (x 1 - x 0 ) , q = N / p = (x 1 + x 0 ) .
Example 2. Given: N = 1037 , a number with a digit capacity 4. It is required to factor it. We act according to the above algorithm.
- Extract the root from N : √N = 32.202 . Round down: √N = 32 .
- Check whether 32 is divided into 3. Since 32 is not divided by 3, then we subtract 2. So, we assume that the first leg is equal to k 1 = 3 ∙ 10 = 30 , here M = 10 = 30/3 is the scaling factor of the TFT .
- Get the value for the second leg k 2 = 4 ∙ 10 = 40 .
- We find the value of the hypotenuse g = (k 2 1 + k 2 2 ) 0.5 , provided that it is divided by 5, (30 2 +40 2 ) 0.5 = 50.
- Thus, x1 = k1 = 50 and the TFT transforms to the form g = 50 , k 1 = 30, k 2 = 40 .
- Find the inflection of the number N : f (1037) = 1037 (mod10) = 7 .
- Find the center of the rhombus nearest to N = 1037 .
It will have the coordinates of the central rhombus cell: x 1 = 50, x 0 = 35 . The first coordinate is the number of the line containing the CFT. The square of the smaller leg is 900, contained in the vertical with the number 40. The cell with the number 957 ending in seven, closest to 900, lies in the preceding horizontal with number 49 and in the vertical with number 38. This is the smallest number of 4 in the diamond and flexion 7. The data in Table 1 is used here. The nearest center of the rhombus should be left three spaces, i.e., belongs to the vertical 38 - 3 = 35, this is the second coordinate of the rhombus center. The value of the number in the center cell of the rhombus is N (50, 35) = 1275
This is a rhombus, having squares of legs k 1 and k 2 on its borders. Within this rhombus, the min number ending in a seven 957 in a cell ( x 1 = 49, x 0 = 38 ), and another number in this vertical ending in 7th 1157 , large numbers 1377 and 1577 lie to the left of the central cell, matches the number N = 1037 is not, therefore, it is necessary to climb to the rhombus to the left and above with the value in the central cell 1125 and with the coordinates of the central cell ( x 1 = 50 - 5 = 45, x 0 = 35 - 5 = 30 ). We check four numbers for flexion 7 These are 847, 1027, 1207 and 1387 and there is no coincidence in this diamond with N = 1037 ), we will rise even higher in the same direction along the NW strip of rhombuses. The center cell of the new rhombus has a value of 975 and coordinates ( x 1 = 45 -5 = 40, x 0 = 35 - 5 = 25 ). We check in this rhombus four numbers per flexion 7. It is 737, 897, 1197 and finally we get 1037 in the cell ( x 1p = 39, x 0r = 22 ) received a full match with the given N.
These actions are presented in detail by the following calculations. In accordance with Table 1, we calculate the coordinates of the cells and the values of the numbers in them. After that, we find the differences between the calculated and the given values of N. In the first rhombus, all 4 cells are calculated.
Δ = N (x 1 c -1, x 0 c -3) - N (x 1 , x 0 ) = N (49,32) - 1037 = 1377 - 1037 = 340 ≠ 0,
∆ = N (x 1 c +1, x 0 c -3) - N (x 1 , x 0 ) = N (51.32) - 1037 = 1577 - 1037 = 540 ≠ 0,
∆ = N (x 1 c +1, x 0 c +3) - N (x 1 , x 0 ) = N (51.38) - 1037 = 1157 - 1037 = 120 0,
∆ = N (x 1 c -1, x 0 c +3) - N (x 1 , x 0 ) = N (49.38) - 1037 = 957 - 1037 = - 80 ≠ 0.
In this rhombus there is no coincidence of the number N with the numbers in the cells.
Go to the next rhombus with the center in the cell (x 1ts -5, x 0ts -5) = (45, 30) and the value in it is N (x 1ts - 5, x 0ts - 5) = N (45, 30) = 1125 .
Δ = N (x 1 c -1, x 0 c -3) - N (x 1 , x 0 ) = N (44.27) - 1037 = 1207 - 1037 = 170 0,
∆ = N (x 1 c +1, x 0 c -3) - N (x 1 , x 0 ) = N (46.27) - 1037 = 1387 - 1037 = 350 0,
∆ = N (x 1 c +1, x 0 c +3) - N (x 1 , x 0 ) = N (46,33) - 1037 = 1027 - 1037 = - 10 0,
∆ = N (x 1 c -1, x 0 c +3) - N (x 1 , x 0 ) = N (44.33) - 1037 = 847 - 1037 = - 190 0.
In this rhombus, there is also no coincidence of the number N with the numbers in the cells.
Go to the next rhombus with the center in the cell (x 1ts -5, x 0ts -5) = (40, 25) and the value in it is N (x 1ts - 5, x 0ts - 5) = N (40, 25) = 975
∆ = N (x 1 c -1, x 0 c -3) - N (x 1 , x 0 ) = N (39.22) - 1037 = 1037 - 1037 = 0.
Received a zero difference of values. There is a complete coincidence. From this it follows that the given number N (x 1 , x 0 ) = 1037 is contained in a cell with coordinates (x 1 , x 0 ) = (39, 22). Finally, the decision of the RQFN is determined by the basic relation G 2 ± - models
N = x 2 1 - x 2 0 = (x 1 - x 0 ) (x 1 + x 0 ) = (39 - 22) (39 + 22) = p ∙ q = 17 61 . - You can act differently. Starting with the rhombus indicated in paragraph 6, using the table. 1, we find out whether the number N belongs to one or another rhombus, moving between the centers of the rhombus, first horizontally, towards the main diagonal, then moving down to the next lane of the rhombus and repeating everything again.
- After establishing the belonging of the number N to a certain rhombus (in our case, the rhombus will have coordinates ( x 1 = 40, x 0 = 25 )) based on the same table. 1 we obtain the coordinates N : x 1p = 39 , x 2p = 22 (9 diamonds viewed).
- Using properties of the chosen mathematical model of number
N = x 2 1 - x 2 0 = (x 1 - x 0 ) (x 1 + x 0 ) = p ∙ q
we obtain the multiplicative representation of N from the additive one:
N = (39 - 22) (39 + 22) = 17 ∙ 61 = 1037 .
Thus, at the output, we have N = p · q = 17 · 61 = 1037 , i.e., the solution of the problem is obtained successfully.
We also obtain the result of the software solution of the problem in Example 3.
Example 3 Given: N = 3808572773, a number with a digit capacity of 10.
- Extract the root from N: √N = 61713 , 64 = 61713 .
- Check whether 61713 is divided by 3. Since 61713 is divisible by 3,
6 + 1 + 7 + 1 + 3 = 18 is divided by 3, then the first leg of k 1 is equal to k 1 = 61713 . - We get the second leg k 2 = 4k 1/3 => 4k 2/3 = 82284 .
- Find the hypotenuse g = √k 12 + k 22 , provided that it is divided into
5 · g = √ 617132 + 822842 = 102855 . - Thus x 1 = k 1 = 61713 , and the Pythagorean triple is transformed accordingly to the form k 1 = 61713, k 2 = 82284, g = 102855 .
- Find the inflection of the number N: f (3808572773) = 3808572773 (mod10) = 3 .
- Find the nearest center of the diamond. It will have coordinates x 1 = 61715; x 9 = 0 .
- Starting with the rhombus indicated in paragraph 7, using the data table. 1, we find out the belonging of the number N to one or another rhombus, moving between the centers of the rhombus, first horizontally, towards the main diagonal, then moving to the next lane of the rhombus and repeating everything again.
- After establishing the belonging of the number N to a certain rhombus (in our case, the center of the rhombus will have the coordinates x 1 = 62015 , x 0 = 6085 ) we get N :
x 1p = 62013; x 0p = 6086 ; (60 diamonds examined). - Using the properties of the selected mathematical model
N = x 2 1 - x 2 0 = (x 1 - x 0 ) (x 1 + x 0 ) = p · q we get a multiplicative representation of N from the additive:
N = (62013 - 6086) · (62013 + 62086) = 55927 · 68099 = 3808572773 ; - Thus, at the output we have N = p · q = 55927 · 68099 = 3808572773 , i.e., the solution of the problem is obtained successfully.
Source: https://habr.com/ru/post/459520/
All Articles