The most beautiful theorem of mathematics: Euler's identity
After seeing Professor Robin Wilson’s lecture on Euler's identity, I was finally able to understand why Euler's identity is the most beautiful equation. To share my admiration for this topic and to strengthen my own knowledge, I will outline the notes made during the lecture. And here you can buy his beautiful book.
What could be more mysterious than the interaction of imaginary numbers with real numbers, resulting in nothing? Such a question was asked by the reader of the journal Physics World in 2004 to emphasize the beauty of the Euler equation "e to the power of i multiplied by pi equals minus one" .
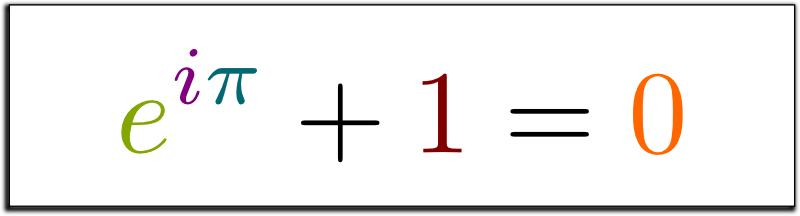
Figure 1.0 : Euler's identity - e to degree i multiplied by pi, plus one equals zero.
')
Even earlier, in 1988, the mathematician David Wells, who wrote articles for The Mathematical Intelligencer , the American mathematical journal, compiled a list of 24 theorems of mathematics and conducted a survey asking the readers of his article to choose the most beautiful theorem. And after winning the Euler equation with a large margin, it received the title of "the most beautiful equation in mathematics."

Figure 2.0 : The Mathematical Intelligencer Magazine Cover

Figure 3.0 : David Wells survey from the magazine
Leonard Euler is called the most productive mathematician ever. Other outstanding mathematicians were inspired by his work. One of the best physicists in the world, Richard Feynman, in his famous lectures on physics, called the Euler equation "the most remarkable formula in mathematics" . Another terrific mathematician, Michael Atiya, called this formula "... the mathematical analogue of Hamlet's phrase“ to be or not to be ”- very short, very compressed, and at the same time very deep” .
There are many interesting facts about the Euler equation. For example, it was found in some episodes of The Simpsons.

Figure 4.0 : in this scene, the Euler equation can be seen in the second book in the rightmost stack.

Figure 5.0 : In this scene, the Euler equation is written on a minor character T-shirt.
Also, the Euler equation became a key point in the criminal case . In 2003, a graduate student at the California Institute of Technology, Billy Cottrell, painted Euler's equation on other people's sports cars with paint on other people's sports cars. At trial, he said: " I have known Euler's theorem since I was five, and everyone must know it ."

Figure 6.0 : The bill issued in 1983 in Germany in memory of the bicentennial anniversary of the death of Euler.

Figure 7.0 : a stamp issued by Switzerland in 1957 in honor of the 250th anniversary of Euler.
You have every right to ask: why did Billy Cottrell think that everyone should know about the Euler equation? And I was so sure that I started writing it on other people's machines? The answer is simple: Euler used three fundamental constants of mathematics and applied mathematical operations of multiplication and exponentiation to write a beautiful formula, resulting in zero or minus one.
The Euler identity first appeared in 1748 in his book, Introductio in analysin infinitorum . Later, other people saw that this formula is connected with the trigonometric functions of sine and cosine, and this connection is amazing, because the power function tends to infinity, and the trigonometric functions vary in the range from - 1 to -1.
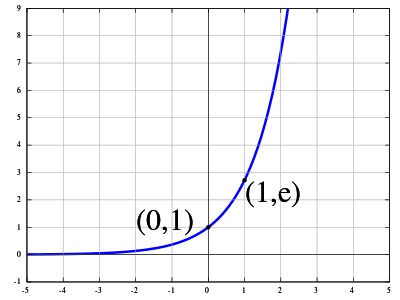
Figure 8.0 : The exponential function y = ex.
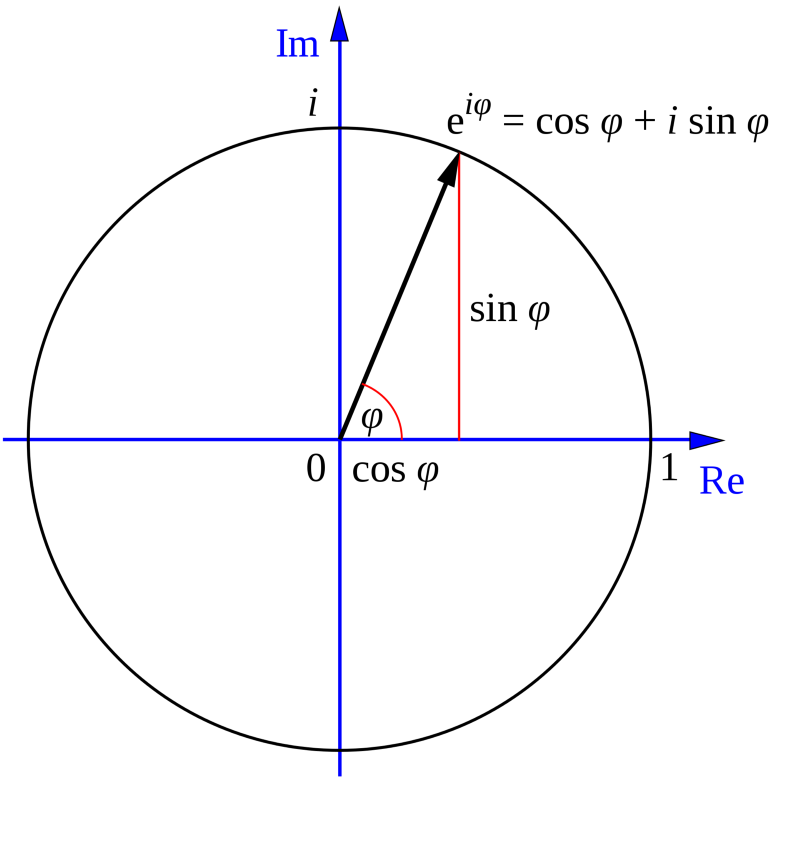
Figure 8.1 : Euler identity graph.
Figure 8.2 : Frequencies emitted by the LC circuit.
The equations and graphs shown above may seem abstract, but they are important for quantum physics and image processing calculations, and depend on Euler's identity.
The number 1 (unit) is the basis of our counting system. With it, we start the account. But how do we think? To calculate, we use digits 0–9 and a system of digits that determines the value of the digit.
For example, the number 323 means 3 hundred, 2 ten and 3 units. Here the number 3 plays two different roles that depend on its location.
323 = (3 * 100) + (2 * 10) + (3 * 1)
There is another system of calculus, called binary. In this system, instead of 1, the base 2 is used. It is widely used in computers and programming. For example, in the binary system:
1001 = (2 3 ) + (0 2 ) + (0 1 ) + (2 0 ) = [9 in the system with base 10]
Who created the number systems? How did the first people count objects or animals?
How did our calculus system come about? How did the first civilizations count? We know for sure that they did not use our bit system. For example, 4000 years ago, the ancient Egyptians used the system of calculus with different symbols. However, they combined the symbols, creating a new symbol denoting numbers.
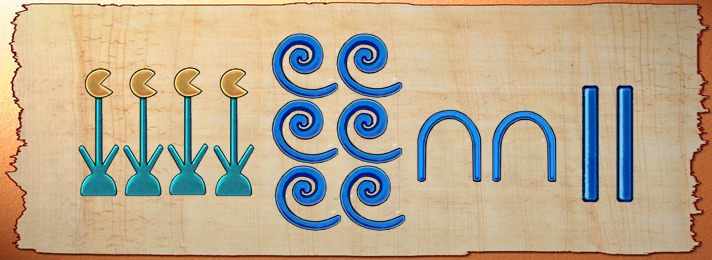
Figure 11 : the hieroglyphs shown here form the number 4622; this is one of the numbers carved on the wall in the temple of Karnak (Egypt).
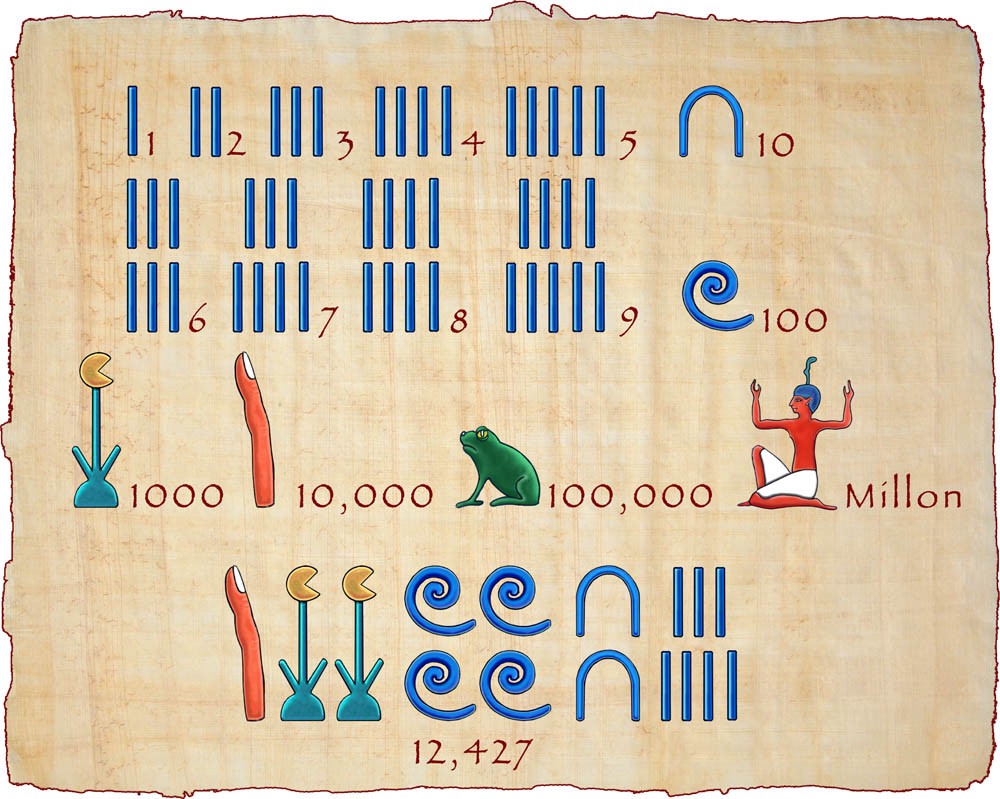
Figure 12 : Hieroglyphs are images that represent words, and in this case, numbers.
At the same time, but in another place, another society found a method of calculation, but symbols were also used in it. In addition, the basis of their counting system was 60, not 10. We use their counting method to determine the time; therefore in a minute of 60 seconds, and in an hour of 60 minutes.

Figure 13 : The Babylonian numbers from the hexadecimal number system (with a base of 60).
A thousand years later, the ancient Romans invented Roman numbers. They used letters to denote numbers. Roman notation is not considered a discharge system, because for many of the values of our number system it uses different letters. It is for this reason that they used an abacus for the account.
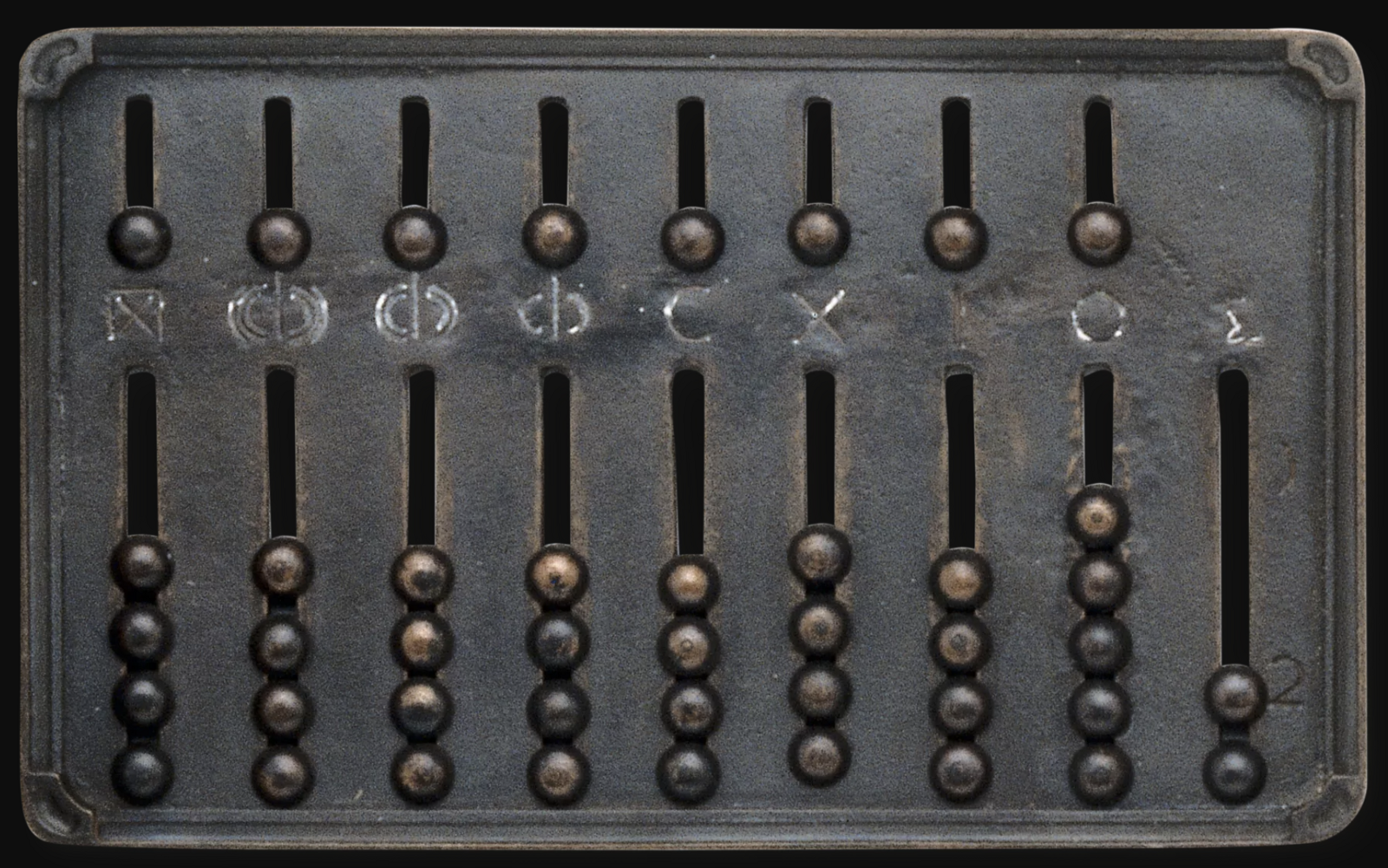
Figure 14 : Roman Abacus in hexadecimal (with base 16) number system

Figure 15 : Conversion Table from Arabic to Roman Numbers
The ancient Greeks also did not use the bit number system. Greek mathematicians denoted numbers by letters. They had special letters for numbers from 100 to 900. Many people at that time considered Greek numbers confusing.
Figure 15 : the table of letters of the ancient Greeks.
At the same time, Chinese mathematicians began to use small bamboo sticks for calculations. This Chinese counting method is called the first decimal bit system.
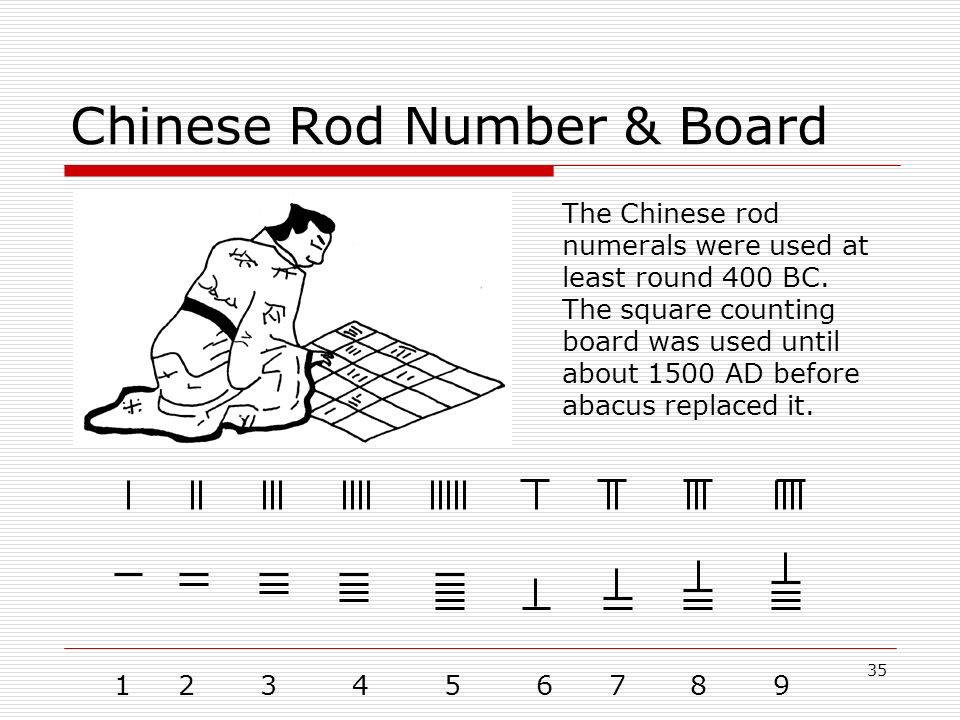
Figure 16 : The Chinese way of counting with chopsticks. It has been used since at least 400 BC. The square counting board was used until about the year 1500, when the abacus replaced it.
However, the most unique counting system was used by the Maya Indians. Their number system had a base of 20. They used points and lines to denote numbers from 1 to 19. What was different about their number system? For each number, they used heads and a single zero character 0.
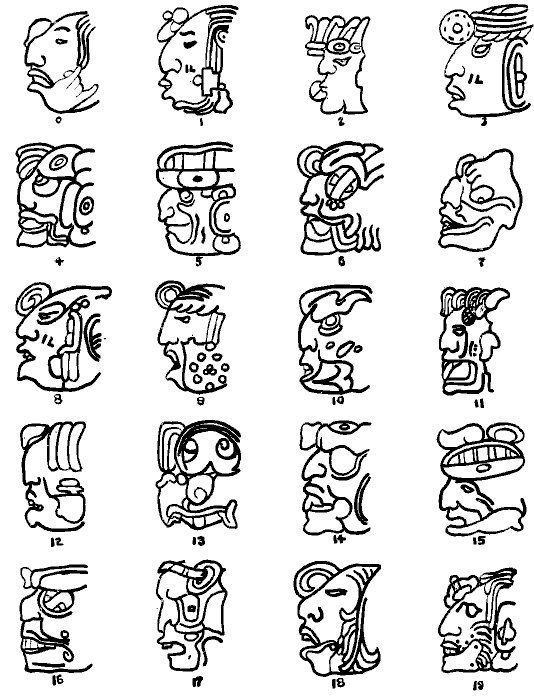
Figure 17: Maya number system with a base of 20, in which the numbers were designated by heads

Figure 18 : Another way to write Maya numbers.
Some civilizations used spaces to, for example, distinguish the number 101 from 11. After some time, a special number began to appear - zero. For example, in a cave in the Indian city of Gwalior, archaeologists discovered on the wall the number 270, in which there was zero. The very first recorded use of zero can be seen in the Bodleyan library.

Figure 19 : The circle carved on the wall of the Gwalior temple denotes zero. He is about 1500 years old.

Figure 20 : The black dots in the Bakhshali manuscript denote zeros; This is the oldest written example of using numbers, it is about 1800 years old.
About 1400 years ago, the rules for computing with zero were written. For example, adding a negative number and a zero results in the same negative number. Division by zero is not allowed, because if divided by zero, we will get a number that can be equal to any number we need, which should be prohibited.
Shortly thereafter, many people published books on arithmetic, spreading the use of Indo-Arabic writing of numbers. The following shows the evolution of Indo-Arabic numbers. Most countries use the Indo-Arabic number system, but Arab countries still use Arabic numbers.

Figure 21 : this diagram shows the evolution of numbers derived from Brahmi numbers and ending with the numbers we use today.

Figure 22 : The classic engraving “Arithmetic” from Margarita Philosophica by Gregor Reisch, which depicts a competition between Boetzia, smiling after discovering Indo-Arabic numbers and written calculations, and a frowning Pythagoras, who are still trying to use a calculating board.
Pi is the most popular irrational number known to us. Pi can be found in two ways: by calculating the ratio of the circumference of a circle to its diameter, or the ratio of the area of a circle to the square of its radius. Euclid proved that these relations are constant for all circles, even for the moon, penny, tires, etc.
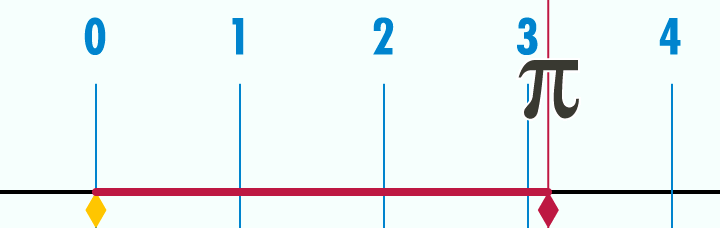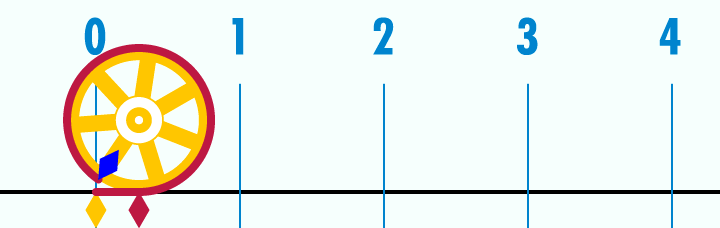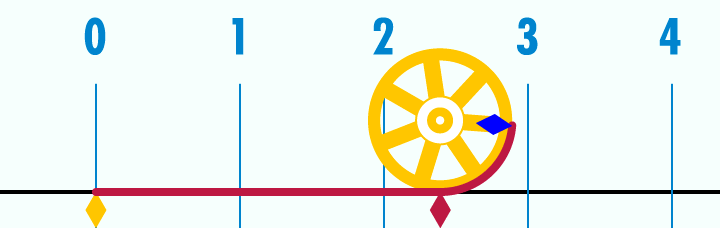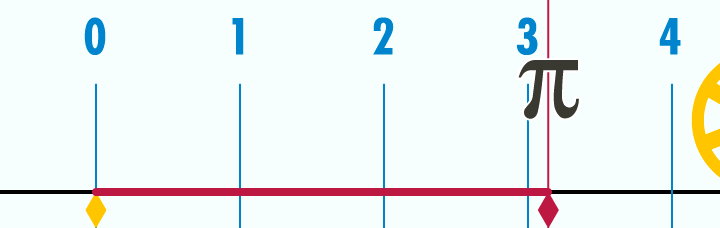
Figure 22 : An animated connection between a circle and a diameter in relation to pi.
Since irrational numbers like pi are infinite and have no repetitions, we will never finish writing pi. It goes on forever. There are people who remember many decimal places pi (the current record is 70,000 digits! Source: "Guinness Book of Records" ).
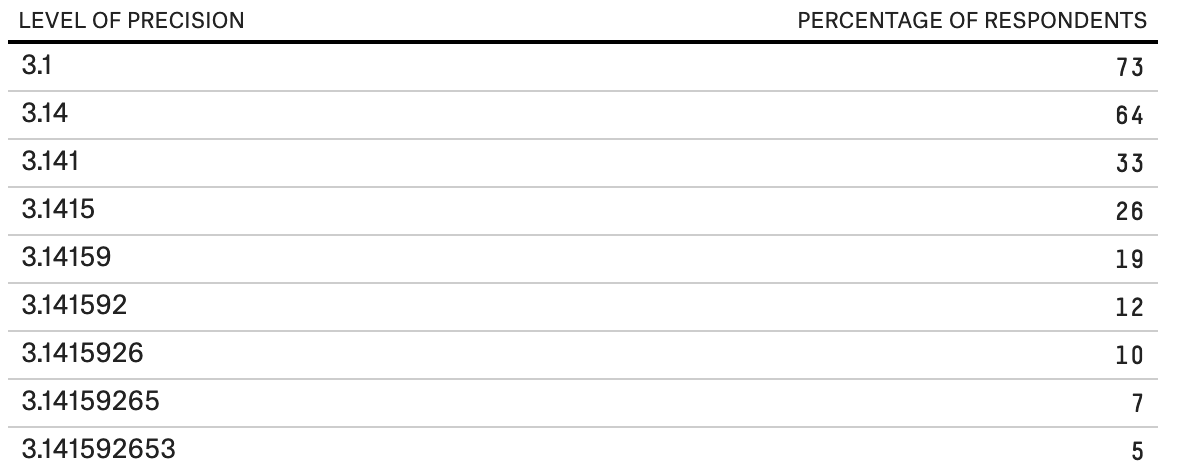
Figure 23 : data from a survey of 941 respondents to determine the percentage of people who can memorize pi signs after the comma.

Figure 24 : On the wall of the Karlsplatz underground station in Vienna, hundreds of pi discharges are recorded.
At the moment, computers were able to calculate only 2.7 trillion digits of pi. It may seem like a lot, but in fact this path is endless.
As I said above, pi found Euclid. But what did people do before Euclid when they needed to find the area of a circle? Historians have discovered a Babylonian clay tablet in which the ratio of the perimeter of a hexagon to the circumference described around it was recorded. After calculations, the resulting number turned out to be 3.125. It is very close to pi.

Figure 24 : Babylonian clay tablet with a hexagonal perimeter ratio to the circumference of the circumscribed circle.

Figure 25 : Numberwarrior
The ancient Egyptians, too, came close to the meaning of pi. Historians have discovered a document showing how the ancient Egyptians found pi. When historians translated the document, they found the following problem:


Figure 26 : Ahmes Mathematical Papyrus .
However, Greek mathematicians found a better way to calculate pi. For example, Archimedes preferred to work with perimeters. He began to draw circles describing polygons of various sizes. When he drew a hexagon, he drew a circle with a diameter of 1. Then he saw that each side of the hexagon was 1/2 and the perimeter of the hexagon was 1/2 x 6 = 3. Then he increased the number of sides of the polygon until it looked like a circle . Working with a 96-sided polygon and applying the same method, he received 2 decimal places pi after the comma: 3 and 10/71 = 3.14084. Many years later, the Chinese mathematician Liu Hu used a 3072-sided polygon and obtained the number 3.14159 (5 correct decimal digits pi after the comma). After that, another Chinese mathematician Zu Chunzhi did an even more impressive job. He worked with a 24,000-sided polygon and got 3.1415926 - seven correct decimal digits of pi after the comma.
After a thousand years, the German mathematician Ludolf Zeylen worked with a 2,622-sided polygon and received 35 decimal digits of pi. This number, called Ludolfov, was carved on his gravestone.



In 1706, Englishman John Machin, a long time professor of astronomy, used the addition formula to prove that pi is equal to
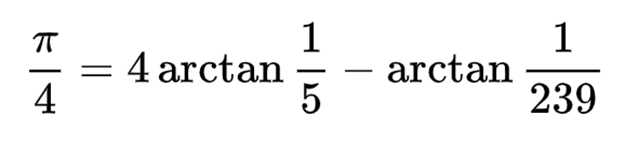
Without worrying about how this formula came from, Macin began to use it constantly, and then wrote down the series shown below. It was the largest step at that time in the number of pi discharges.

Figure 29 : Machin's formula for pi
However, the first mention of pi appeared in 1706. Mathematics teacher William Jones wrote a book and first proposed pi to measure circles. So pi first appeared in the books!



Figure 30 : Juliabloggers
In 1873, William Shanks took advantage of John Machin's formula and got 707 decimal pi. These figures are written in the room pi of the Paris Palace of Discovery. However, later mathematicians found out that only 527 digits are correct.

Figure 31 : room pi
On the other hand, a more interesting way of finding pi discovered Buffon. His experiment was based on the random scattering of needles for evaluating pi. He drew several parallel lines on the board at a distance D and took needles of length L. Then he randomly began to throw needles on the board and wrote down the proportion of needles crossing the line.

Figure 32 : Science Friday
After that, another mathematician named Lazarini threw a needle 3408 times and received six decimal places pi with a ratio of 355/113. However, if one needle had not crossed the line, he would have received only 2 digits of pi.
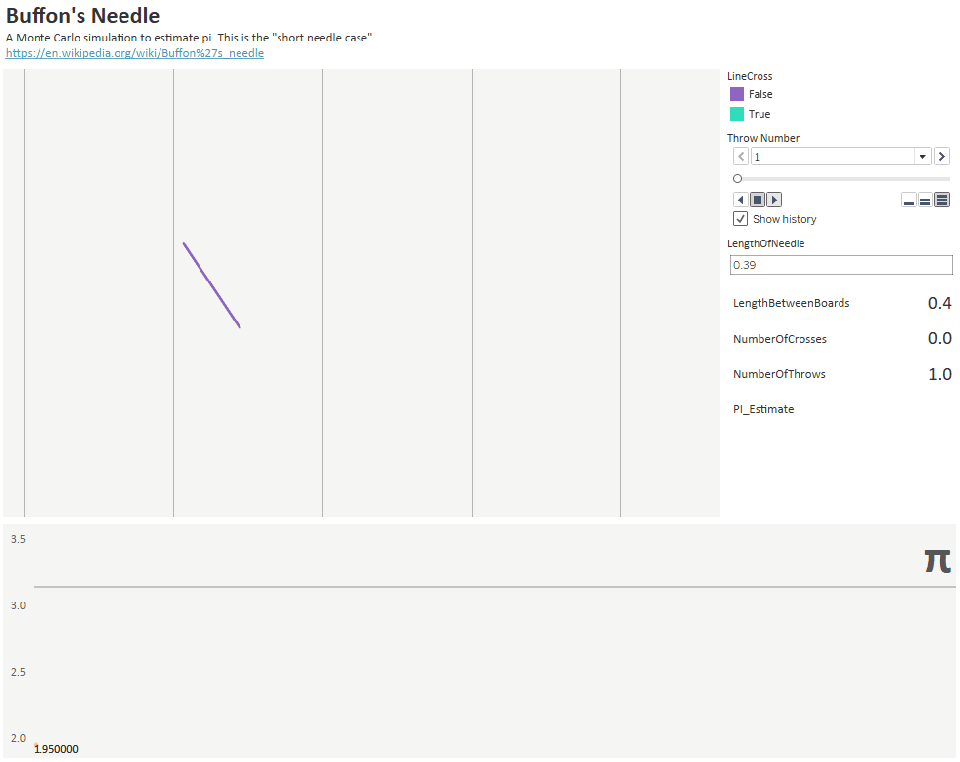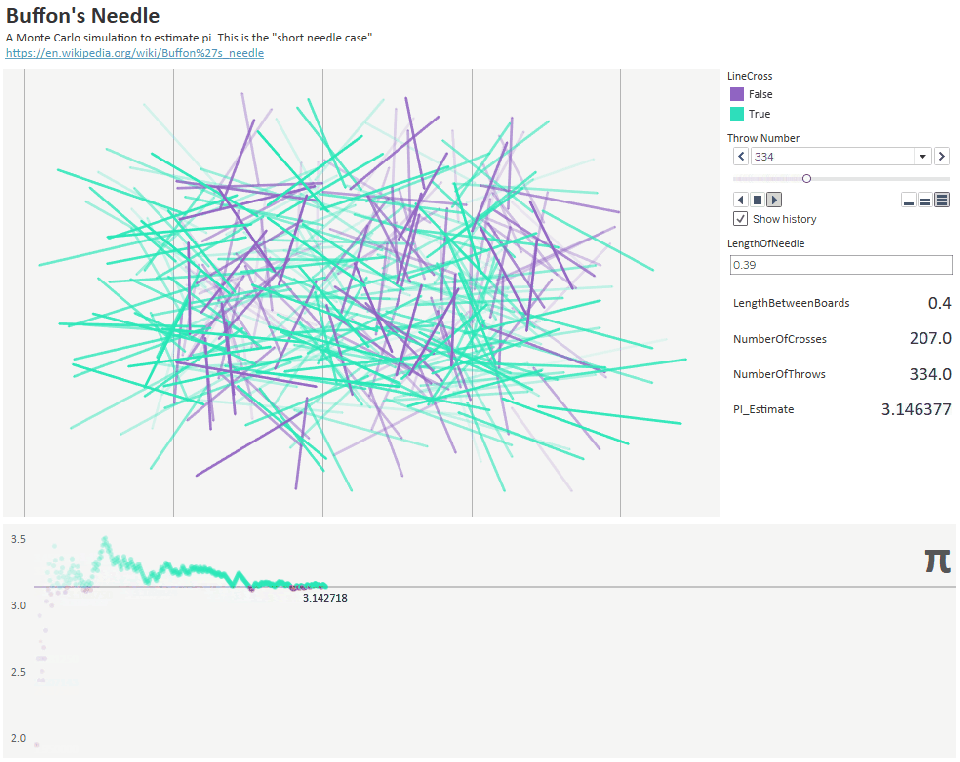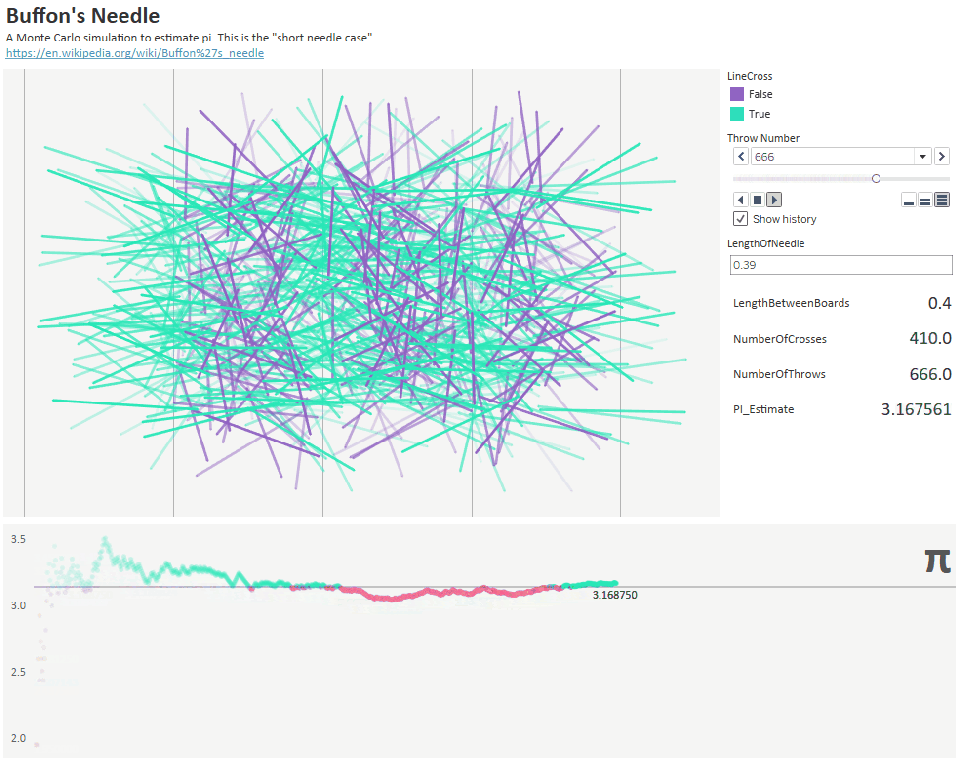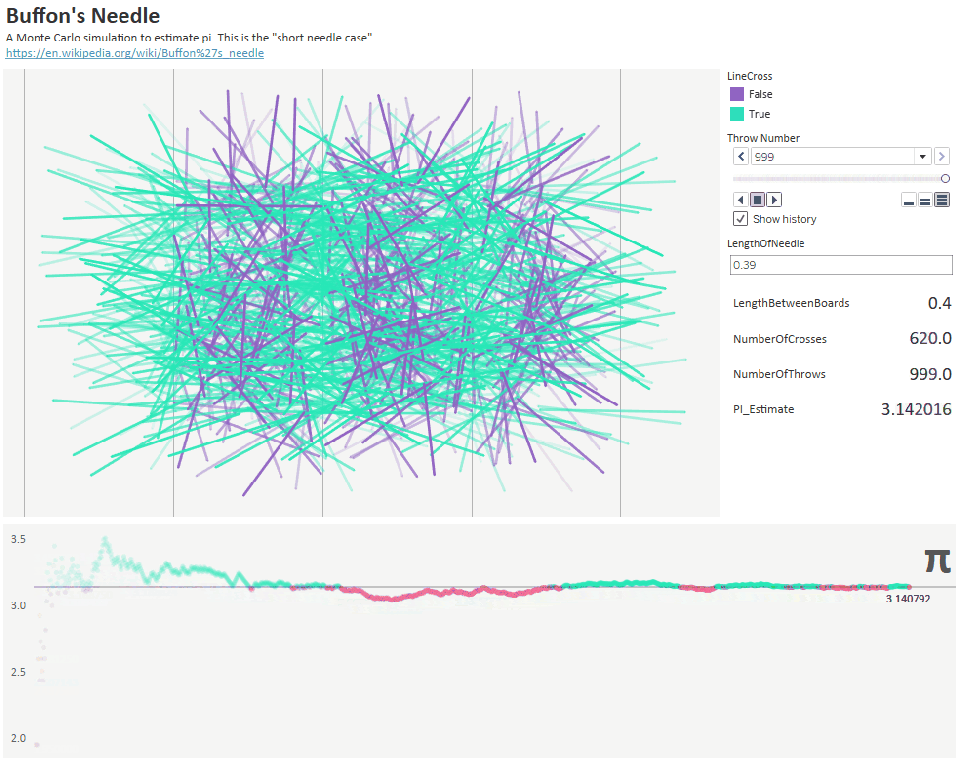
Figure 32 : throwing 1000 needles to estimate the approximate value of pi
e is another famous irrational number. The fractional part of e is also infinite, as in pi. We use the number e to calculate the power (exponential) growth. In other words, we use e when we see very rapid growth or decrease.
One of the greatest, and possibly the best mathematician Leonard Euler discovered the number e in 1736 and first mentioned this particular number in his book Mechanica .


Figure 33 : Source
To understand the exponential growth, we can use the story of the inventor of chess. When he invented this game, he showed it to the ruler of the North. The king liked the game and he promised that he would give the author any reward. Then the inventor asked for something very simple: 2 0 grains to the first cell of the chessboard, 2 1 grains to the second cell of the board, 2 2 grains to the third, and so on. Each time the amount of grain doubled. The King of the North thought that the request would be easy to fulfill, but he was wrong, because then 2 63 grains would have to be put on the last cell, which is equal to 9,223,372,036,854,775,808 . This is an exponential growth. It began with 1, constantly doubled, and after 64 steps it grew into a huge number!
If the chess inventor chose a linear equation, for example 2n, he would get 2, 4, 6, 8, ... 128 ... Therefore, in the long run, the exponential growth often exceeds the polynomial growth.

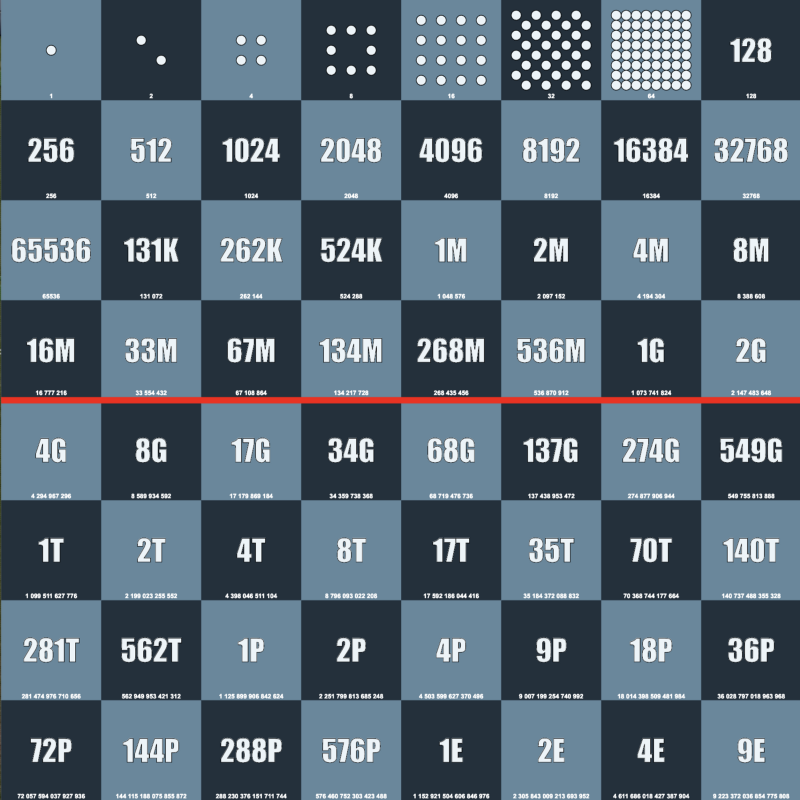
Figure 34 : Source: Wikipedia
The number e was discovered by Euler. However, Jacob Bernoulli also worked with the number e , when he calculated compound interest to earn more money. If you invest 100 dollars at 10% of income, how will this amount grow? First, it depends on how often the bank calculates interest. For example, if he calculates once, then we will receive $ 110 at the end of the year. If we change our mind and take interest every 6 months, in this case we will get more than 110 dollars. The point is that the interest received in the first 6 months will also receive its interest. The total amount will be equal to 110.25 dollars. One can guess that we can get more money if we take the money every quarter of the year. And if we make the time interval shorter, the final amounts will continue to grow. Such an infinite compound interest will make us rich! However, our total revenue tends to a limited value associated with e .
Bernoulli did not name the number 2,71828 name e . When Euler worked from 2.71828, he raised the exponential function e to the power x . He outlined his discoveries in the book The Analysis of Infinite .
In 1798, Thomas Malthus used the exponential function in his essay on the food deficit of the future. He created a line graph showing food production and an exponential graph showing the world's population. Malthus concluded that exponential growth would win in the long run, and the world would face a serious food shortage. This phenomenon was called the "Malthusian catastrophe." Newton also used this model to show how a cup of tea cools.

Figure 35 : Newton-Richman's Law
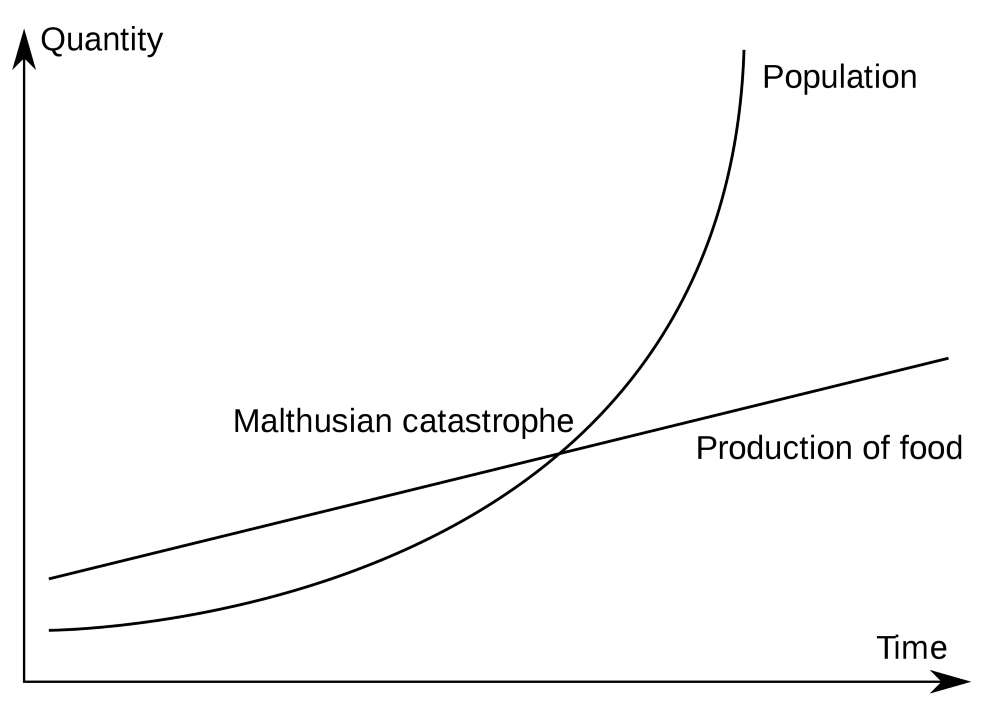
Figure 36 : Malthusian Catastrophe
For a long time, mathematics were enough ordinary numbers to solve their problems. However, at some point for further development they needed to discover something new and mysterious.For example, the Italian mathematician Cardano tried to divide the number 10 into 2 parts, the product of which would be equal to 40. To solve this problem, he wrote the equation: x (10-x) = 40. When he solved this quadratic equation, he got two solutions: 5 plus √-15 and 5 minus √-15, which at that time did not make any sense. This result was meaningless, because by definition of the square root he needed to find a number whose square would be negative. However, both positive and negative numbers in a square have a positive value. Anyway, he found his unique number. However, the first mathematician who called √-1 (the square root of minus ones) the imaginary number i was Euler.
Leibniz made the following comment about the imaginary number √-1:
We can add, subtract, multiply and divide imaginary numbers. Addition, subtraction and multiplication are simple, and the division is a bit more complicated. Real and imaginary parts are added separately. In the case of multiplication, i 2 will be -1.
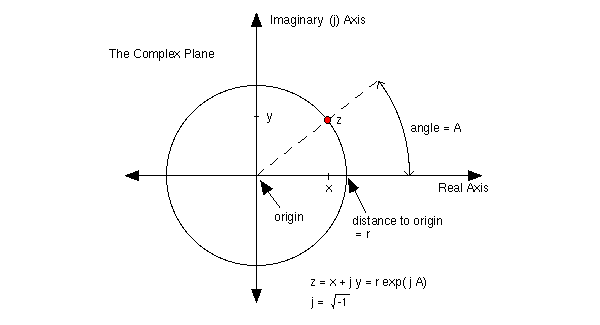
After Euler, mathematician Caspar Wessel presented imaginary numbers geometrically with having created a complex plane. Today we represent each complex number a + bi as a point with coordinates (a, b).
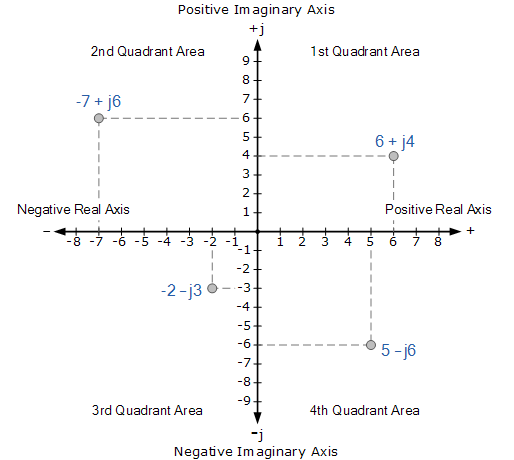
Figures 37 and 38 : complex numbers
In the Victorian era, many people regarded imaginary numbers with suspicion. However, the Irish mathematician and astronomer William Rowan Hamilton did away with these doubts by defining complex numbers as applied to quaternions .
Euler's identity connects the exponential function with sine and cosine functions, the values of which range from minus one to one. To find a bond with trigonometric functions, we can represent them in the form of an infinite series, true for all values
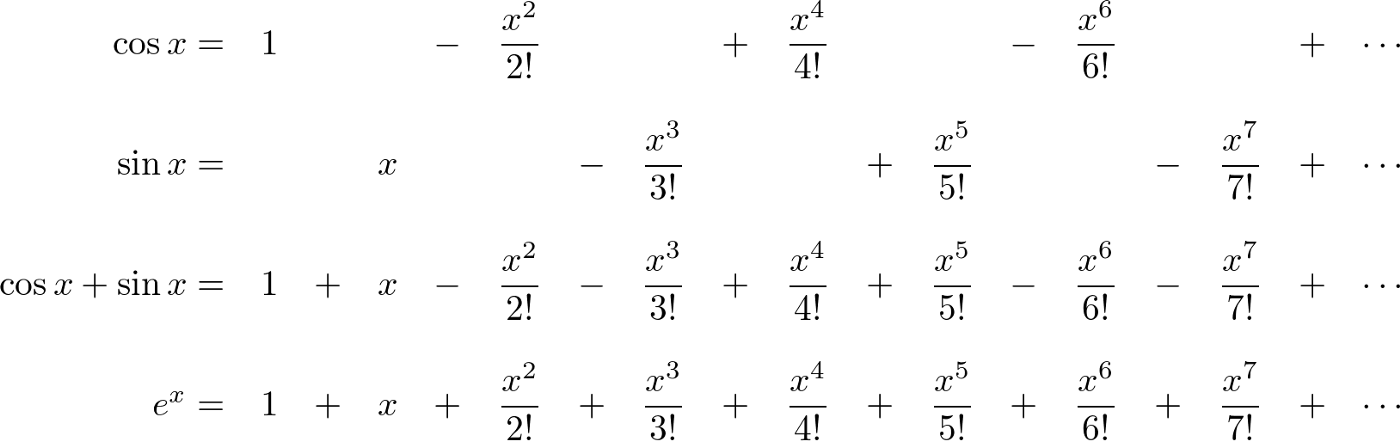
Figure 39 : Discovery of the Euler Identity
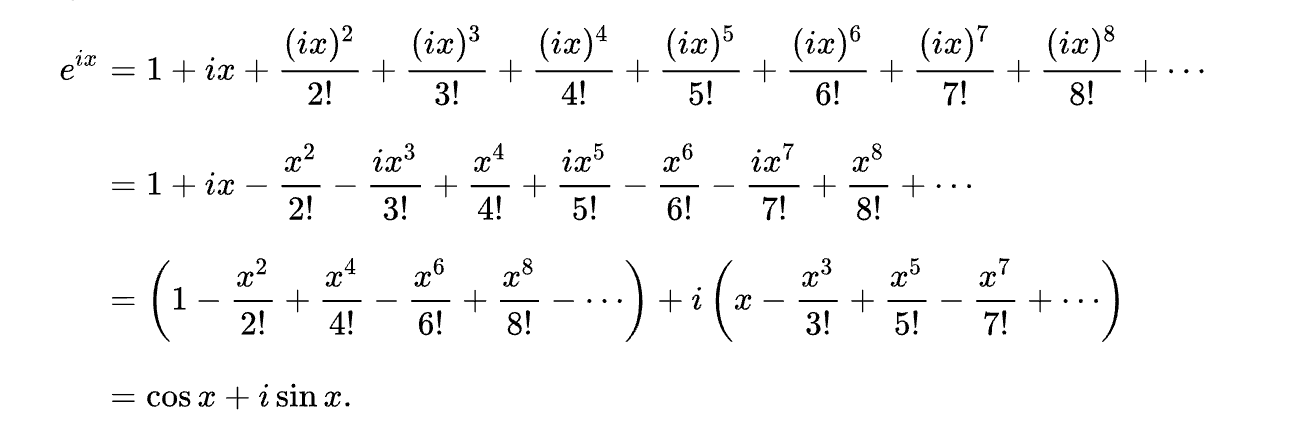
Figure 40 : Euler's identity
Euler never recorded this identity explicitly, and we do not know who first wrote it down. Nevertheless, we associate him with the name of Euler in deference to this great pioneer of mathematics.
What could be more mysterious than the interaction of imaginary numbers with real numbers, resulting in nothing? Such a question was asked by the reader of the journal Physics World in 2004 to emphasize the beauty of the Euler equation "e to the power of i multiplied by pi equals minus one" .
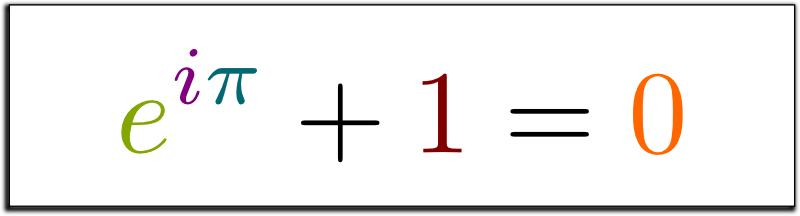
Figure 1.0 : Euler's identity - e to degree i multiplied by pi, plus one equals zero.
')
Even earlier, in 1988, the mathematician David Wells, who wrote articles for The Mathematical Intelligencer , the American mathematical journal, compiled a list of 24 theorems of mathematics and conducted a survey asking the readers of his article to choose the most beautiful theorem. And after winning the Euler equation with a large margin, it received the title of "the most beautiful equation in mathematics."

Figure 2.0 : The Mathematical Intelligencer Magazine Cover

Figure 3.0 : David Wells survey from the magazine
Leonard Euler is called the most productive mathematician ever. Other outstanding mathematicians were inspired by his work. One of the best physicists in the world, Richard Feynman, in his famous lectures on physics, called the Euler equation "the most remarkable formula in mathematics" . Another terrific mathematician, Michael Atiya, called this formula "... the mathematical analogue of Hamlet's phrase“ to be or not to be ”- very short, very compressed, and at the same time very deep” .
There are many interesting facts about the Euler equation. For example, it was found in some episodes of The Simpsons.

Figure 4.0 : in this scene, the Euler equation can be seen in the second book in the rightmost stack.

Figure 5.0 : In this scene, the Euler equation is written on a minor character T-shirt.
Also, the Euler equation became a key point in the criminal case . In 2003, a graduate student at the California Institute of Technology, Billy Cottrell, painted Euler's equation on other people's sports cars with paint on other people's sports cars. At trial, he said: " I have known Euler's theorem since I was five, and everyone must know it ."

Figure 6.0 : The bill issued in 1983 in Germany in memory of the bicentennial anniversary of the death of Euler.

Figure 7.0 : a stamp issued by Switzerland in 1957 in honor of the 250th anniversary of Euler.
Why is the Euler equation so important?
You have every right to ask: why did Billy Cottrell think that everyone should know about the Euler equation? And I was so sure that I started writing it on other people's machines? The answer is simple: Euler used three fundamental constants of mathematics and applied mathematical operations of multiplication and exponentiation to write a beautiful formula, resulting in zero or minus one.
- The constant e is associated with power functions.
- The constant i is not real, but an imaginary number equal to the square root of minus ones.
- The famous constant π (pi) is associated with circles.
The Euler identity first appeared in 1748 in his book, Introductio in analysin infinitorum . Later, other people saw that this formula is connected with the trigonometric functions of sine and cosine, and this connection is amazing, because the power function tends to infinity, and the trigonometric functions vary in the range from - 1 to -1.
e to the power of i multiplied by ϕ (phi) = cos ϕ (phi) + sin ϕ (phi)

Figure 8.0 : The exponential function y = ex.

Figure 8.1 : Euler identity graph.

Figure 8.2 : Frequencies emitted by the LC circuit.
The equations and graphs shown above may seem abstract, but they are important for quantum physics and image processing calculations, and depend on Euler's identity.
1: number for the account
The number 1 (unit) is the basis of our counting system. With it, we start the account. But how do we think? To calculate, we use digits 0–9 and a system of digits that determines the value of the digit.
For example, the number 323 means 3 hundred, 2 ten and 3 units. Here the number 3 plays two different roles that depend on its location.
323 = (3 * 100) + (2 * 10) + (3 * 1)
There is another system of calculus, called binary. In this system, instead of 1, the base 2 is used. It is widely used in computers and programming. For example, in the binary system:
1001 = (2 3 ) + (0 2 ) + (0 1 ) + (2 0 ) = [9 in the system with base 10]
Who created the number systems? How did the first people count objects or animals?
How did our calculus system come about? How did the first civilizations count? We know for sure that they did not use our bit system. For example, 4000 years ago, the ancient Egyptians used the system of calculus with different symbols. However, they combined the symbols, creating a new symbol denoting numbers.

Figure 11 : the hieroglyphs shown here form the number 4622; this is one of the numbers carved on the wall in the temple of Karnak (Egypt).

Figure 12 : Hieroglyphs are images that represent words, and in this case, numbers.
At the same time, but in another place, another society found a method of calculation, but symbols were also used in it. In addition, the basis of their counting system was 60, not 10. We use their counting method to determine the time; therefore in a minute of 60 seconds, and in an hour of 60 minutes.

Figure 13 : The Babylonian numbers from the hexadecimal number system (with a base of 60).
A thousand years later, the ancient Romans invented Roman numbers. They used letters to denote numbers. Roman notation is not considered a discharge system, because for many of the values of our number system it uses different letters. It is for this reason that they used an abacus for the account.

Figure 14 : Roman Abacus in hexadecimal (with base 16) number system

Figure 15 : Conversion Table from Arabic to Roman Numbers
The ancient Greeks also did not use the bit number system. Greek mathematicians denoted numbers by letters. They had special letters for numbers from 100 to 900. Many people at that time considered Greek numbers confusing.

Figure 15 : the table of letters of the ancient Greeks.
At the same time, Chinese mathematicians began to use small bamboo sticks for calculations. This Chinese counting method is called the first decimal bit system.

Figure 16 : The Chinese way of counting with chopsticks. It has been used since at least 400 BC. The square counting board was used until about the year 1500, when the abacus replaced it.
However, the most unique counting system was used by the Maya Indians. Their number system had a base of 20. They used points and lines to denote numbers from 1 to 19. What was different about their number system? For each number, they used heads and a single zero character 0.
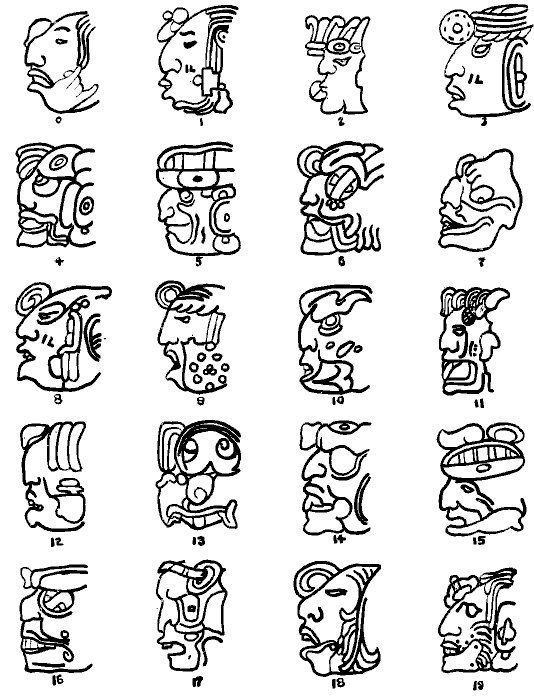
Figure 17: Maya number system with a base of 20, in which the numbers were designated by heads

Figure 18 : Another way to write Maya numbers.
0: a number to indicate nothing
Some civilizations used spaces to, for example, distinguish the number 101 from 11. After some time, a special number began to appear - zero. For example, in a cave in the Indian city of Gwalior, archaeologists discovered on the wall the number 270, in which there was zero. The very first recorded use of zero can be seen in the Bodleyan library.

Figure 19 : The circle carved on the wall of the Gwalior temple denotes zero. He is about 1500 years old.

Figure 20 : The black dots in the Bakhshali manuscript denote zeros; This is the oldest written example of using numbers, it is about 1800 years old.
About 1400 years ago, the rules for computing with zero were written. For example, adding a negative number and a zero results in the same negative number. Division by zero is not allowed, because if divided by zero, we will get a number that can be equal to any number we need, which should be prohibited.
Shortly thereafter, many people published books on arithmetic, spreading the use of Indo-Arabic writing of numbers. The following shows the evolution of Indo-Arabic numbers. Most countries use the Indo-Arabic number system, but Arab countries still use Arabic numbers.

Figure 21 : this diagram shows the evolution of numbers derived from Brahmi numbers and ending with the numbers we use today.

Figure 22 : The classic engraving “Arithmetic” from Margarita Philosophica by Gregor Reisch, which depicts a competition between Boetzia, smiling after discovering Indo-Arabic numbers and written calculations, and a frowning Pythagoras, who are still trying to use a calculating board.
Pi (π): the most famous irrational number
Pi is the most popular irrational number known to us. Pi can be found in two ways: by calculating the ratio of the circumference of a circle to its diameter, or the ratio of the area of a circle to the square of its radius. Euclid proved that these relations are constant for all circles, even for the moon, penny, tires, etc.
π = circumference / diameter OR π = area of a circle / radius²

Figure 22 : An animated connection between a circle and a diameter in relation to pi.
Since irrational numbers like pi are infinite and have no repetitions, we will never finish writing pi. It goes on forever. There are people who remember many decimal places pi (the current record is 70,000 digits! Source: "Guinness Book of Records" ).

Figure 23 : data from a survey of 941 respondents to determine the percentage of people who can memorize pi signs after the comma.

Figure 24 : On the wall of the Karlsplatz underground station in Vienna, hundreds of pi discharges are recorded.
At the moment, computers were able to calculate only 2.7 trillion digits of pi. It may seem like a lot, but in fact this path is endless.
As I said above, pi found Euclid. But what did people do before Euclid when they needed to find the area of a circle? Historians have discovered a Babylonian clay tablet in which the ratio of the perimeter of a hexagon to the circumference described around it was recorded. After calculations, the resulting number turned out to be 3.125. It is very close to pi.

Figure 24 : Babylonian clay tablet with a hexagonal perimeter ratio to the circumference of the circumscribed circle.

Figure 25 : Numberwarrior
The ancient Egyptians, too, came close to the meaning of pi. Historians have discovered a document showing how the ancient Egyptians found pi. When historians translated the document, they found the following problem:
For example, to find the area of a field with a diameter of 9 het (1 hat = 52.35 meters), you need to perform the following calculation:
Subtract 1/9 of the diameter, namely 1. The remainder is 8. Multiply it by 8, which gives us 64. Therefore, the area will be 64 setjat (unit of area).
In other words, the diameter is 2r, and 1/9 of the radius is (1/9 • 2r). Then if we subtract it from the original diameter, we get 2r - (1/9 • 2r) = 8/9 (2r). Then the area of the circle is 256/81 r². That is, pi is almost 3.16. They discovered this pi value about 4,000 years ago.


Figure 26 : Ahmes Mathematical Papyrus .
However, Greek mathematicians found a better way to calculate pi. For example, Archimedes preferred to work with perimeters. He began to draw circles describing polygons of various sizes. When he drew a hexagon, he drew a circle with a diameter of 1. Then he saw that each side of the hexagon was 1/2 and the perimeter of the hexagon was 1/2 x 6 = 3. Then he increased the number of sides of the polygon until it looked like a circle . Working with a 96-sided polygon and applying the same method, he received 2 decimal places pi after the comma: 3 and 10/71 = 3.14084. Many years later, the Chinese mathematician Liu Hu used a 3072-sided polygon and obtained the number 3.14159 (5 correct decimal digits pi after the comma). After that, another Chinese mathematician Zu Chunzhi did an even more impressive job. He worked with a 24,000-sided polygon and got 3.1415926 - seven correct decimal digits of pi after the comma.
After a thousand years, the German mathematician Ludolf Zeylen worked with a 2,622-sided polygon and received 35 decimal digits of pi. This number, called Ludolfov, was carved on his gravestone.



In 1706, Englishman John Machin, a long time professor of astronomy, used the addition formula to prove that pi is equal to
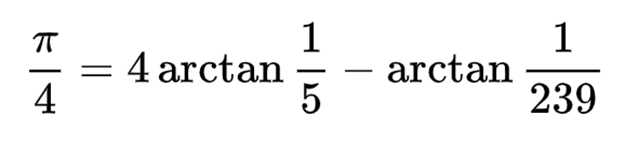
Without worrying about how this formula came from, Macin began to use it constantly, and then wrote down the series shown below. It was the largest step at that time in the number of pi discharges.

Figure 29 : Machin's formula for pi
However, the first mention of pi appeared in 1706. Mathematics teacher William Jones wrote a book and first proposed pi to measure circles. So pi first appeared in the books!



Figure 30 : Juliabloggers
In 1873, William Shanks took advantage of John Machin's formula and got 707 decimal pi. These figures are written in the room pi of the Paris Palace of Discovery. However, later mathematicians found out that only 527 digits are correct.

Figure 31 : room pi
On the other hand, a more interesting way of finding pi discovered Buffon. His experiment was based on the random scattering of needles for evaluating pi. He drew several parallel lines on the board at a distance D and took needles of length L. Then he randomly began to throw needles on the board and wrote down the proportion of needles crossing the line.

Figure 32 : Science Friday
After that, another mathematician named Lazarini threw a needle 3408 times and received six decimal places pi with a ratio of 355/113. However, if one needle had not crossed the line, he would have received only 2 digits of pi.

Figure 32 : throwing 1000 needles to estimate the approximate value of pi
e: history of exponential growth
e is another famous irrational number. The fractional part of e is also infinite, as in pi. We use the number e to calculate the power (exponential) growth. In other words, we use e when we see very rapid growth or decrease.
One of the greatest, and possibly the best mathematician Leonard Euler discovered the number e in 1736 and first mentioned this particular number in his book Mechanica .


Figure 33 : Source
To understand the exponential growth, we can use the story of the inventor of chess. When he invented this game, he showed it to the ruler of the North. The king liked the game and he promised that he would give the author any reward. Then the inventor asked for something very simple: 2 0 grains to the first cell of the chessboard, 2 1 grains to the second cell of the board, 2 2 grains to the third, and so on. Each time the amount of grain doubled. The King of the North thought that the request would be easy to fulfill, but he was wrong, because then 2 63 grains would have to be put on the last cell, which is equal to 9,223,372,036,854,775,808 . This is an exponential growth. It began with 1, constantly doubled, and after 64 steps it grew into a huge number!
If the chess inventor chose a linear equation, for example 2n, he would get 2, 4, 6, 8, ... 128 ... Therefore, in the long run, the exponential growth often exceeds the polynomial growth.
By the way, 9 223 372 036 854 775 808-1 is the maximum value of a 64-bit signed integer .


Figure 34 : Source: Wikipedia
The number e was discovered by Euler. However, Jacob Bernoulli also worked with the number e , when he calculated compound interest to earn more money. If you invest 100 dollars at 10% of income, how will this amount grow? First, it depends on how often the bank calculates interest. For example, if he calculates once, then we will receive $ 110 at the end of the year. If we change our mind and take interest every 6 months, in this case we will get more than 110 dollars. The point is that the interest received in the first 6 months will also receive its interest. The total amount will be equal to 110.25 dollars. One can guess that we can get more money if we take the money every quarter of the year. And if we make the time interval shorter, the final amounts will continue to grow. Such an infinite compound interest will make us rich! However, our total revenue tends to a limited value associated with e .
Bernoulli did not name the number 2,71828 name e . When Euler worked from 2.71828, he raised the exponential function e to the power x . He outlined his discoveries in the book The Analysis of Infinite .
In 1798, Thomas Malthus used the exponential function in his essay on the food deficit of the future. He created a line graph showing food production and an exponential graph showing the world's population. Malthus concluded that exponential growth would win in the long run, and the world would face a serious food shortage. This phenomenon was called the "Malthusian catastrophe." Newton also used this model to show how a cup of tea cools.

Figure 35 : Newton-Richman's Law

Figure 36 : Malthusian Catastrophe
Filthy number: i, square root -1
For a long time, mathematics were enough ordinary numbers to solve their problems. However, at some point for further development they needed to discover something new and mysterious.For example, the Italian mathematician Cardano tried to divide the number 10 into 2 parts, the product of which would be equal to 40. To solve this problem, he wrote the equation: x (10-x) = 40. When he solved this quadratic equation, he got two solutions: 5 plus √-15 and 5 minus √-15, which at that time did not make any sense. This result was meaningless, because by definition of the square root he needed to find a number whose square would be negative. However, both positive and negative numbers in a square have a positive value. Anyway, he found his unique number. However, the first mathematician who called √-1 (the square root of minus ones) the imaginary number i was Euler.
Leibniz made the following comment about the imaginary number √-1:
Complex numbers are a beautiful and wonderful refuge of the divine spirit, almost like an amphibian of being with non-being.
We can add, subtract, multiply and divide imaginary numbers. Addition, subtraction and multiplication are simple, and the division is a bit more complicated. Real and imaginary parts are added separately. In the case of multiplication, i 2 will be -1.
After Euler, mathematician Caspar Wessel presented imaginary numbers geometrically with having created a complex plane. Today we represent each complex number a + bi as a point with coordinates (a, b).

Figures 37 and 38 : complex numbers
In the Victorian era, many people regarded imaginary numbers with suspicion. However, the Irish mathematician and astronomer William Rowan Hamilton did away with these doubts by defining complex numbers as applied to quaternions .
The most beautiful equation: Euler's identity
Euler's identity connects the exponential function with sine and cosine functions, the values of which range from minus one to one. To find a bond with trigonometric functions, we can represent them in the form of an infinite series, true for all values
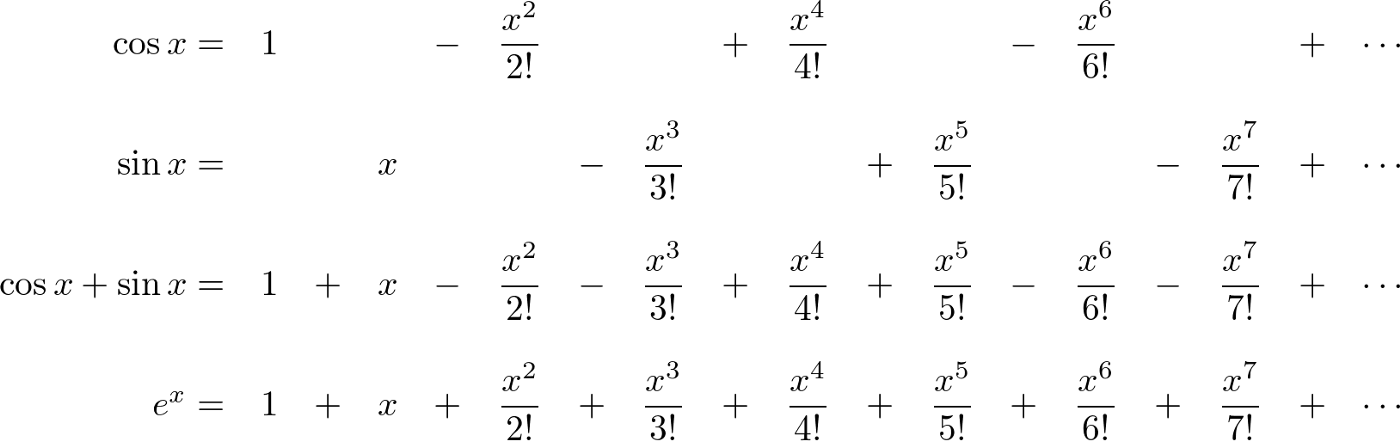
Figure 39 : Discovery of the Euler Identity
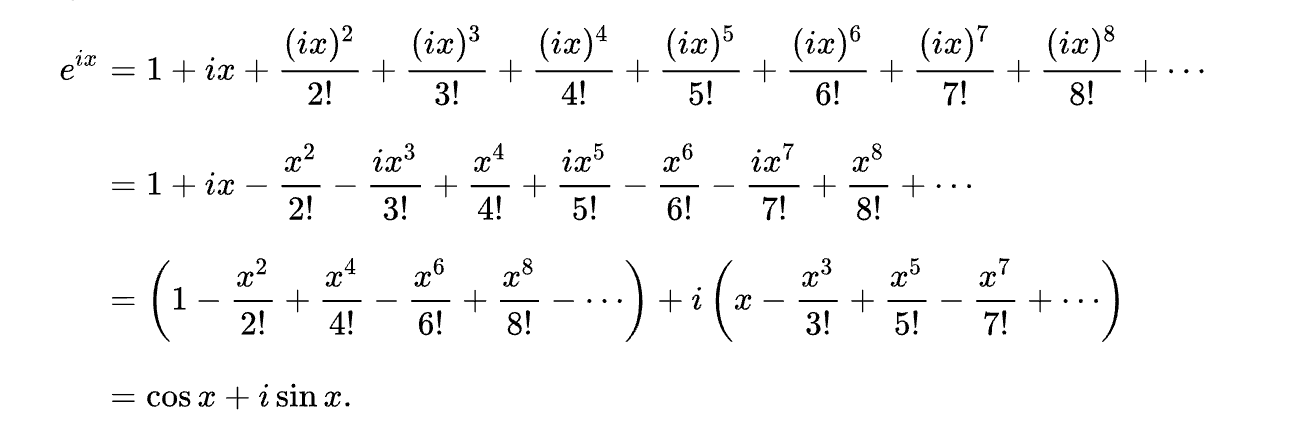
Figure 40 : Euler's identity
Euler never recorded this identity explicitly, and we do not know who first wrote it down. Nevertheless, we associate him with the name of Euler in deference to this great pioneer of mathematics.
Source: https://habr.com/ru/post/454136/
All Articles