Thermodynamics of black holes

Happy Cosmonautics Day! We handed over to the printing house "A little book about black holes . " It is these days that astrophysicists showed the whole world how black holes look. Coincidence? We don’t think;) So wait, there will soon be an amazing book written by Steven Habser and Frans Pretorius, translated by the wonderful Pulkovo astronomer aka Astroed Cyril Maslennikov, made the science editor the legendary Vladimir Surdin and supported its publication Trajectory Foundation.



The passage "Thermodynamics of black holes" under the cut.



Until now, we have considered black holes as astrophysical objects that were formed during supernova explosions or lie in the centers of galaxies. We observe them indirectly by measuring the acceleration of stars close to them. The famous registration of gravitational waves receiver LIGO September 14, 2015 was an example of more direct observations of the collision of black holes. The mathematical tools we use to achieve a better understanding of the nature of black holes are: differential geometry, Einstein's equations, and powerful analytical and numerical methods used to solve Einstein's equations and when describing the space-time geometry that black holes generate. And as soon as we can give a complete quantitative description of the space-time generated by a black hole, from an astrophysical point of view, the topic of black holes can be considered closed. In a broader theoretical perspective, there are still many opportunities for research. The purpose of this chapter is to tell about some theoretical achievements of modern black hole physics, in which the ideas of thermodynamics and quantum theory are combined with the general theory of relativity, giving rise to unexpected new concepts. The basic idea is that black holes are not just geometric objects. They have a temperature, they have enormous entropy and can demonstrate manifestations of quantum entanglement. Our reasoning about the thermodynamic and quantum aspects of black hole physics will be more fragmentary and superficial than the analysis of purely geometric features of space-time in black holes presented in previous chapters. But both these and especially quantum aspects are an essential and vital part of the ongoing theoretical studies of black holes, and we will try very hard to convey, if not complex details, then at least the spirit of these works.



In the classical general theory of relativity - if we talk about the differential geometry of solutions of the Einstein equations - black holes are truly black in the sense that nothing can get out of them. Stephen Hawking showed that this situation changes completely when we take into account the quantum effects: black holes, it turns out, emit radiation of a certain temperature, known as Hawking temperature. For astrophysical black holes (that is, from black holes of stellar masses to supermassive), Hawking temperature is negligible compared to the temperature of the cosmic microwave background — radiation that fills the entire Universe, which, by the way, can itself be considered a Hawking radiation variant. Hawking's calculations for determining the temperature of black holes are part of a more extensive research program in the field called black hole thermodynamics. Another large part of this program is the study of the entropy of black holes, which characterizes the amount of information lost inside a black hole. Ordinary objects (such as a cup of water, a bar of pure magnesium or a star) also have entropy, and one of the central statements of black hole thermodynamics is that a black hole of this size has more entropy than any other form of matter that can be accommodated an area of ​​the same size, but without the formation of a black hole.

')

But before we dive deep into an analysis of the problems associated with Hawking radiation and the entropy of black holes, let us take a quick look at quantum mechanics, thermodynamics, and entanglement. Quantum mechanics was developed mainly in the 1920s, and its main purpose was to describe very small particles of matter, such as atoms. The development of quantum mechanics led to the erosion of such basic concepts of physics as the exact position of an individual particle: it turned out, for example, that the position of an electron as it moves around the atomic nucleus cannot be accurately determined. Instead, electrons were assigned so-called orbits, in which their real positions can only be determined in a probabilistic sense. For our purposes, however, it is important not to move to this - probabilistic - side of the matter too quickly. Take the simplest example: a hydrogen atom. It can be in a certain quantum state. The simplest state of the hydrogen atom, called the ground state, is the one with the lowest energy, and this energy is precisely known. In a more general sense, quantum mechanics allows us (in principle) to know the state of any quantum system absolutely exactly.



Probabilities come to the scene when we ask a certain kind of questions about a quantum mechanical system. For example, if it is definitely known that the hydrogen atom is in the ground state, we can ask: “Where is the electron?” And according to the laws of quantum

mechanics we get to this question only a certain estimate of probability, approximately something like: “the electron is probably at a distance of up to half an angstrom from the nucleus of the hydrogen atom” (one angstrom is equal to 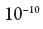meters). But we have the possibility, through a certain physical process, to find the position of an electron much more accurately than before one angstrom. This rather common process in physics consists in launching a photon with a very short wavelength into an electron (or, as physicists say, scattering a photon on an electron) - after that we will be able to reconstruct the electron's position at the moment of scattering with an accuracy approximately equal to photon. But this process will change the state of the electron, so that after that it will no longer be in the ground state of the hydrogen atom and will not have exactly certain energy. But for some time its position will be almost exactly determined (up to the wavelength of the photon used for this). A preliminary assessment of the position of an electron can only be carried out in a probabilistic sense with an accuracy of about one angstrom, but as soon as we measure it, we know exactly what it was equal to. In short, if we measure a quantum-mechanical system in some way, then, at least in the generally accepted sense, we “forcibly” give it a state with a certain value of the quantity that we measure.



Quantum mechanics is applicable not only to small, but also (as we believe) to all systems, but for large systems, quantum-mechanical rules quickly become very complex. The key concept is quantum entanglement, a simple example of which is the concept of spin (rotation). Individual electrons have spin, so in practice a single electron can have a spin directed upwards or downwards with respect to the chosen spatial axis. The spin of an electron is an observable quantity because the electron generates a weak magnetic field, similar to the field of a magnetic bar. Then the upward spin means that the north pole of the electron points down, and the downward spin means that the north pole "looks" up. Two electrons can be put in a conjugate quantum state, in which one of them has a spin upwards and the other downwards, but it is impossible to say which of the electrons has a spin. In essence, in the ground state of the helium atom, the two electrons are precisely in this state, called the spin-singlet state, since the total spin of both electrons is zero. If we separate these two electrons without changing their spins, we can continue to assert that they are spin-singlet together, but still cannot say what the spin of each of them will be separately. But if we measure one of their spins and establish that it is pointing up, then we will be completely sure that the second is pointing down. In this situation, we say that the backs are tangled — none by itself has a definite meaning, while together they are in a certain quantum state.



Einstein was very worried about the phenomenon of entanglement: it seemed to threaten the basic principles of the theory of relativity. Consider the case of two electrons in a spin-singlet state, when they are far apart in space. For definiteness, let one of them take Alice and the other Bob. Suppose that Alice measured the spin of her electron and found that it is pointing up, and Bob did not measure anything. Until Alice completed her measurement, it was impossible to tell what the spin of his electron was. But as soon as she completed her measurement, she absolutely knew that the electron spin of Bob is directed downward (in the opposite direction to the spin of her own electron). Does this mean that its measurement instantly transferred the electron to the state of Bob in a state where his spin is directed downwards? How could this happen if electrons are spatially separated? Einstein and his collaborators Natan Rosen and Boris Podolsky felt that the story of measuring entangled systems is so serious that it threatens the very existence of quantum mechanics. The Einstein – Podolsky – Rosen paradox (EPR) that they formulated uses a mental experiment similar to the one we have just described to conclude: quantum mechanics cannot be a complete description of reality. Now, on the basis of the theoretical studies that followed and a multitude of measurements, a general opinion has been established that the EPR paradox contains an error, and quantum theory is correct. Quantum-mechanical entanglement is real: measurements of entangled systems will correlate, even if these systems are far apart in space-time.



Let us return to the situation where we put two electrons in a spin-singlet state and distributed them to Alice and Bob. What can we say about electrons before measurements are made? That both together they are in a certain quantum state (spin-singlet). The spin of the Alicin electron with the same probability is directed up or down. More precisely, the quantum state of its electron with the same probability can be one (spin up) or another (spin down). Now for us, the concept of probability takes on a deeper meaning than before. Before, we considered a certain quantum state (the ground state of the hydrogen atom) and saw that there were some “inconvenient” questions, such as “Where is the electron?”, Questions that are answered only in a probabilistic sense. If we asked "good" questions, for example: "What is the energy of this electron?", We would get certain answers to them. Now there are no "good" questions that we could ask about Alicin's electron, the answers to which would not depend on the electron of Bob. (We are not talking about silly questions like “Does the Alicean electron have any spin at all?” - questions for which there is only one answer.) Thus, to determine the parameters of one of the halves of the entangled system, we will have to use a probabilistic language. Certainty arises only when we consider the relationship between questions that Alice and Bob can ask about their electrons.



We purposely began with one of the simplest quantum-mechanical systems that we know: the spin systems of individual electrons. It is hoped that quantum computers will be built on the basis of such simple systems. The system of spins of individual electrons or other equivalent quantum systems are now called qubits (short for “quantum bits”), which emphasizes their role in quantum computers, similar to the role played by ordinary bits in digital computers.



Let us imagine now that we replaced each electron with a much more complex quantum system with many, and not just two, quantum states. For example, they gave Alice and Bob pure magnesium bars. Before Alice and Bob go about their business in different directions, their bars can interact, and we agree that in doing so they acquire a certain common quantum state. As soon as Alice and Bob disperse, their magnesium bars no longer interact. As in the case of electrons, each bar is in an indefinite quantum state, although together, we believe, they form a state quite definite. (In this discussion, we assume that Alice and Bob are able to move their magnesium bars without disturbing their internal state, just as we assumed earlier that Alice and Bob could separate their entangled electrons without changing their spins.) But the difference between this mental experiment and the experiment with electrons is that the uncertainty of the quantum state of each bar is huge. The bar may well acquire more quantum states than the number of atoms in the Universe. This is where thermodynamics comes on the scene. Very imprecisely defined systems may, however, have some well-defined macroscopic characteristics. Such a characteristic is, for example, temperature. Temperature is a measure of the probability with which any part of the system has a certain average energy, with a higher temperature corresponding to a higher probability of having greater energy. Another thermodynamic parameter is entropy, in essence, equal to the logarithm of the number of states that the system can accept. Another thermodynamic characteristic that would be essential for a magnesium bar is its total magnetization, that is, in essence, a parameter that shows how much more electrons in a bar can have a spin upwards than a spin downward.



We have drawn to our story thermodynamics as a way to describe systems whose quantum states are not exactly known because of their entanglement with other systems. Thermodynamics is a powerful tool for analyzing such systems, but its creators did not at all suggest such an application. Sadi Carnot, James Joel, Rudolf Clausius were figures of the industrial revolution of the XIX century, and they were interested in the most practical of all questions: how do engines work? Pressure, volume, temperature and heat are the flesh and blood of the engines. Carnot established that energy in the form of heat can never be completely turned into useful work, like lifting loads. Part of the energy will always be wasted. Clausius made the main contribution to the creation of the idea of ​​entropy as a universal tool for determining energy losses during any process associated with heat. His main achievement was the realization that entropy never decreases - in almost all processes it grows. The processes in which entropy increases are called irreversible - precisely because they cannot go backwards without a decrease in entropy. The next step in the development of statistical mechanics was made by Clausius, Maxwell and Ludwig Boltzmann (among many others) - they showed that entropy is a measure of disorder. Usually, the more you act on something, the more confusion is brought in there. And even if you have developed a process whose goal is to restore order, in the course of it more entropy is inevitably formed than it will be destroyed - for example, when heat is released. The crane, which lays the steel beams in an ideal order, creates orderliness in the sense of the location of the beams, but in the course of its operation, so much heat is released that the total entropy still grows.



But still, the difference between the view on the thermodynamics of nineteenth-century physicists and the view related to quantum entanglement is not as great as it seems. Each time a system interacts with an external agent, its quantum state is entangled with the quantum state of the agent. Usually this entanglement leads to an increase in the uncertainty of the quantum state of the system, in other words, to an increase in the number of quantum states in which the system can be. As a result of interaction with other systems, the entropy, defined in terms of the number of quantum states available to the system, usually increases.



In general, quantum mechanics gives a new way to characterize physical systems in which some parameters (for example, position in space) become uncertain, but others (for example, energy) are often known exactly. In the case of quantum entanglement, two fundamentally separate parts of the system have a known common quantum state, and each part separately is an indeterminate state. The standard example of entanglement is a pair of spins in the singlet state, in which it is impossible to say which spin is directed up and which is directed down. , , , , .



, , , , . — , , , 2π. , , , ‑. , , , . : - ? , , , . , , 6 . , ( , ) . , 1,5 , . , , , , ; , , . , , . ( « , …», , , , .) , , : , , , , , , . , , , , , , , , . : ! , , , , , . , , , , , , . , -. , , - , .



, - , - . , , - : , . , , , , -, . , - , (, , , ). -, , , , , . - , . , 60 . , , ( ) ,







Fig. 7.1. , a . , , t = 0. , t = 0 . , ‑.



, , « ». ? ? , . , , , . — , — , , . -, , ‑. - , , . , !



, , , — , , . «» , «», , , . 2, , , , . , , . , , ? , . , ? , , , . , , .