Levitating nanoparticle cooling via optical resonator

Spring is in full swing, the last snow has melted almost everywhere and the long-awaited warmth reigned. Heat-loving people are finally beginning to take off their knitted sweaters, scarves and hats, and walruses are already walking around in shorts and T-shirts. Meanwhile, in one of the laboratories of the University of Vienna the dog cold reigns and scientists are extremely happy about that, because it is extremely difficult to study the quantum effects of nanoparticles in a different way. If without literary revolutions, today we will get acquainted with the practical testing of a new method of cooling a levitating nanoparticle by means of a trap from an optical resonator. Why and how did scientists freeze the nanoparticle to almost absolute zero, how effective is their method and what can it bring to the study of quantum effects? We will find answers to these and other questions in the report of the research group. Go.

The basis of the experiment

Laser particle capture and cooling is one of the very popular tools in atomic physics, as scientists themselves say, and it’s hard not to agree with them. If you omit all the super-duper complex formulations from which the hair will stand on end even where there is no such thing, then the whole process can be described as follows: motor cooling of an atom or molecule is possible due to the complete rejection of the absorption of Doppler-shifted laser photons and subsequent spontaneous radiation. But this process depends on the internal structure of the cooled particle. This dependence can be avoided by combining the motion of the particle and the field of the optical resonator, which will result in a new cooling scheme.
')
Of great interest in research circles received a method of resonator cooling through coherent field scattering of an optical trap. In such a situation, the controlled dipole produces scattering that is coherent with the excitation field. The scattering of photons in the initially empty optical resonator provides a new method of cooling. If we apply the correct laser detuning of the excitation field from the resonator, then we can resonantly enhance the scattering process, which in turn eliminates the energy from the motion of the particles. It is the removal of this energy that is cooling.


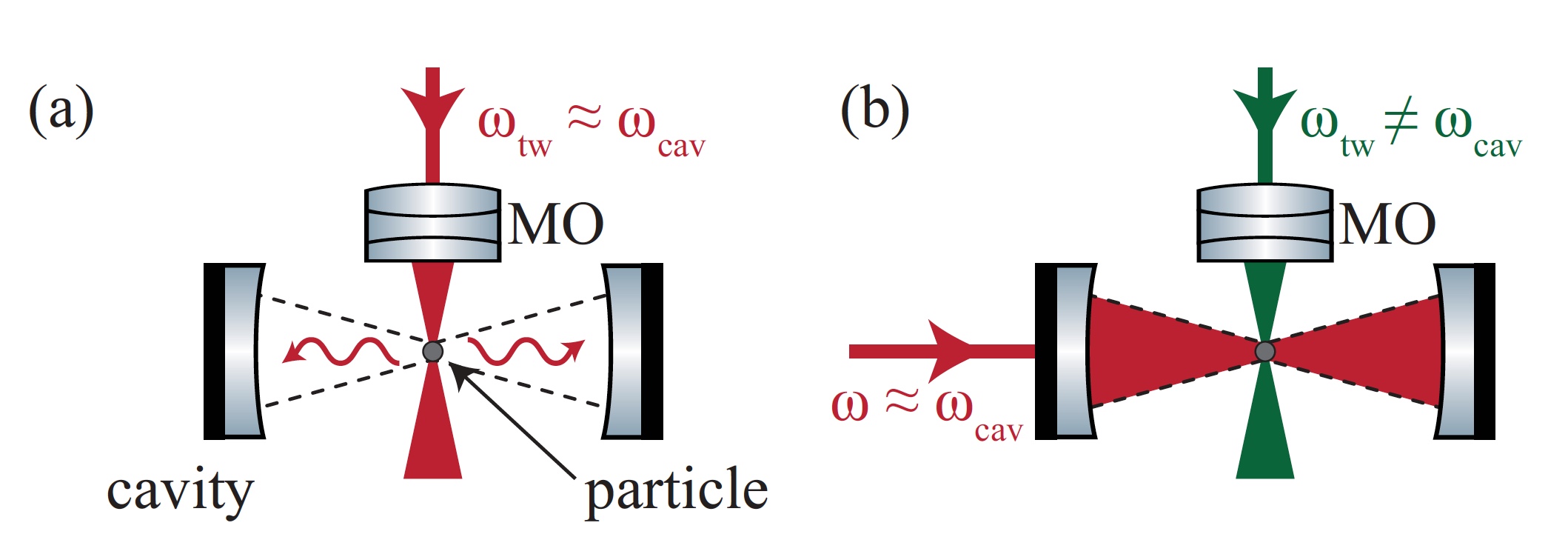
Image # 1: two variants of the resonator cooling of a levitating particle.

Image 1a shows that resonator cooling through coherent scattering from optical traps (sometimes “tweezers”) is based on dipole radiation emitted into an empty optical resonator.

Image 1b - in a standard dispersive optomechanics, an external laser controls both the resonator and scattering. Optimal cooling is possible with the greatest intensity gradient of the resonator mode.

In their work, scientists have demonstrated the practical implementation of the resonator cooling of a levitating nanoparticle through coherent scattering. At the same time, scientists managed to carry out this process in three directions of thermal motion, whereas previously similar experiments were successfully carried out only in one direction and only with atoms as subjects.

Theoretical basis

Who loves beautiful formulas resembling grandmother's lace, now we will look at a couple of these.


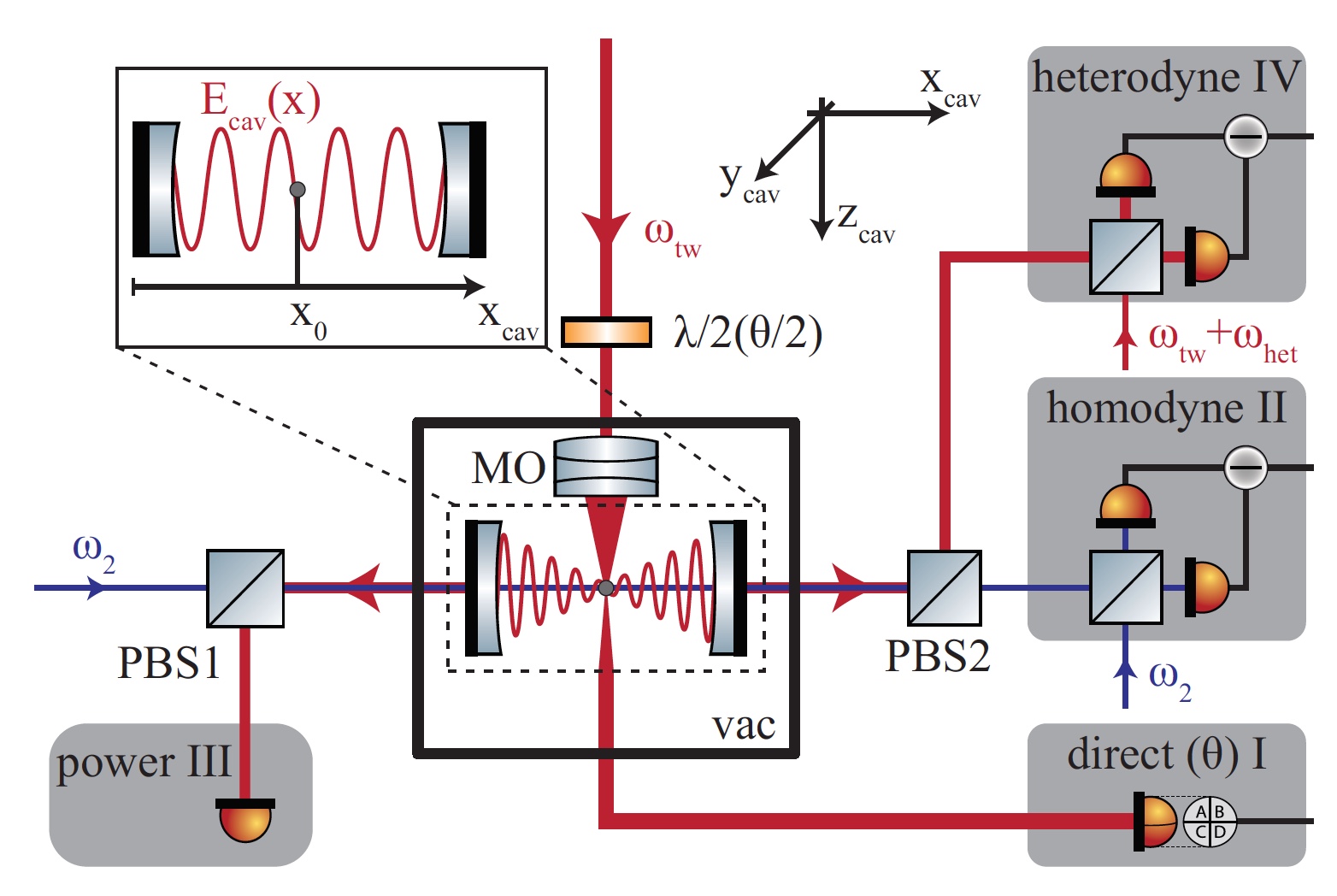
Image No. 2: resonator cooling scheme through coherent scattering.

An optical trap is formed by a laser with a frequency ω _tω , which is focused by a microscope objective ( OM ) inside a vacuum chamber ( vac ). The nanoparticle levitates in the center of the Fabry — Perot resonator. A weak blocking beam is output from the trap laser and resonator controls the optical resonator at frequency ω ₂ , allowing you to stably fix ω _tω and ω ₂ relative to the frequency of the optical resonator. The PBS in the image above is the polarization splitter, and ω _het is the heterodyne demodulation frequency.

Now consider a nanoparticle caught by laser tweezers with beam throats (W _{x; y} , the narrowest part of a laser beam) inside an empty optical resonator with a mode volume V _cav (beam _throttle w ₀ ) and at position x ₀ along the axis of the resonator (image No. 2 ).

In this case, the interaction between the induced dipole and the internal (local) electric field can be described as the following Hamiltonian:



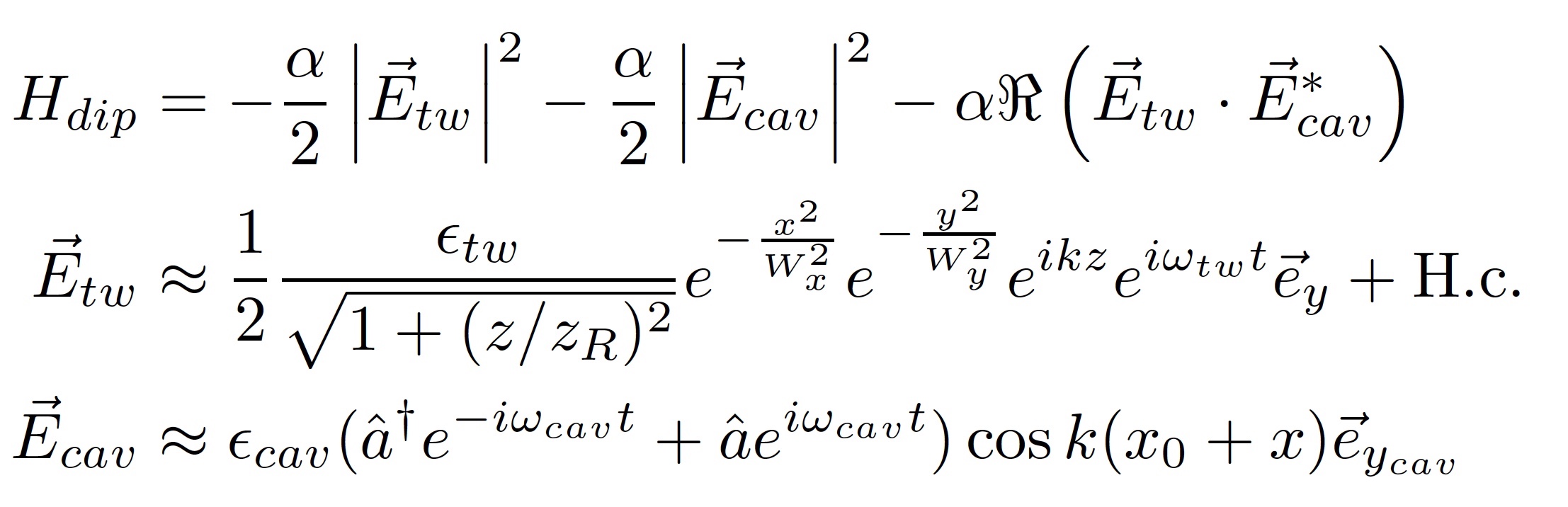
Legend:

E _tw and E _cav - electric fields of fashion tweezers and resonators;
ω _tw is the frequency of tweezers;
ω _cav is the resonator frequency;
⍺ is the polarizability of the particles;
â † and â– resonator field operators;
⍷ ₀ is the electric constant;
c is the speed of light;
k is the wave number;
zR - Rayleigh length.

When the frequency of the tweezers approaches the resonance of the optical resonator, the density of the resonator mode can change the emission spectrum of the dipole, which leads to resonator-enhanced coherent scattering.

Such scattering has several distinctive features. First, due to the directivity of the scattered dipole radiation, the interaction force is very dependent on the polarization of the trapping laser. Secondly, this interaction will be amplified in parallel with the field strength of both the optical trap and the resonator. Third, the interaction in the electric field of the resonator is linear.

Practical implementation

As we can see from the diagram in image 2, the microscope objective and the Fabry — Perot resonator are located inside the vacuum chamber. The lens focuses a 1064 nm laser on a W _x 0.67 μm throat and a W _y 0.77 μm, forming an optical tweezer (trap) that captures a quartz nanosphere with a diameter of 71.5 nm. The trap is elliptic in the transverse plane with non-degenerate mechanical frequencies (Ωx, Ωy, Ωz) / 2Π = (190, 170, 38) kHz. The lens itself is mounted on a three-axis nanopositioner with a step of 8 nm. To control the detuning between the frequencies of the laser and the resonator, a part of the light is shifted in frequency ω ₂ = ω _cav -FSR-Δ and weakly pumps the optical resonator. This provides a fixing signal that allows the laser source for optical tweezers to follow the free-drifting Fabry-Perot cavity.

The experimental setup has 4 detection / detection channels (I-IV). Directly detecting the movement of a particle in all three directions (I), obtained by scattering optical tweezers. Homodyne detection of the transmittance of the fixing laser in the resonator (II), which makes it possible to obtain optomechanical detection of the position along the cavity axis. This is necessary to align the particles with respect to the resonator field without the need to rely on coherently scattered light. The power of coherently scattered photons in the optical resonator (III) was also measured, simultaneously tracking the leakage of the field from the left mirror of the resonator. The fourth (IV) detection is the detection of radiation from the right mirror of the resonator due to the spectrally resolved characteristic of the photons.

Coherent scattering strongly depends on the polarization of optical tweezers. Scientists analyzed three scattering variants for the following linear polarization angles: θ = 0, θ = π / 4, and θ = π / 2.


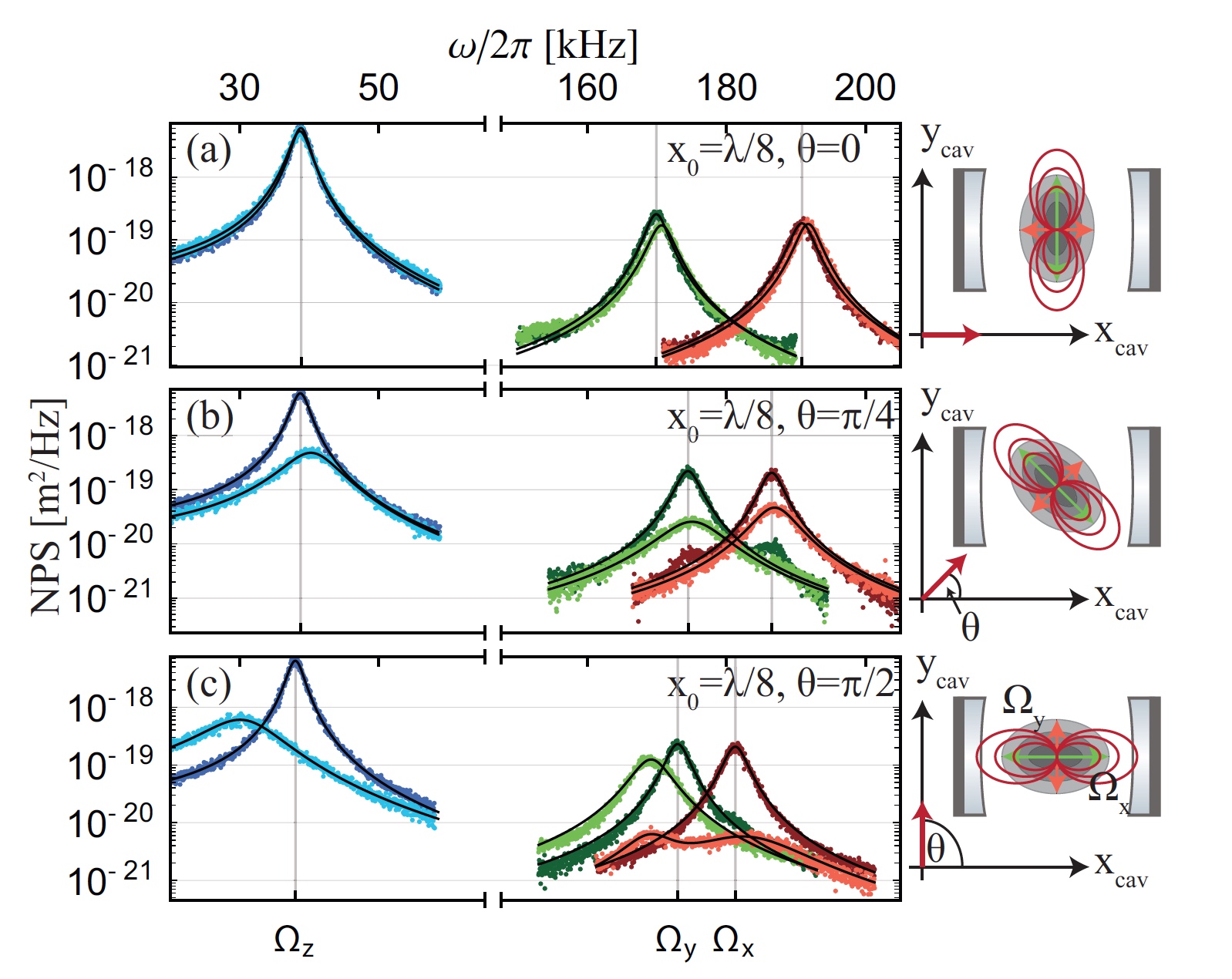
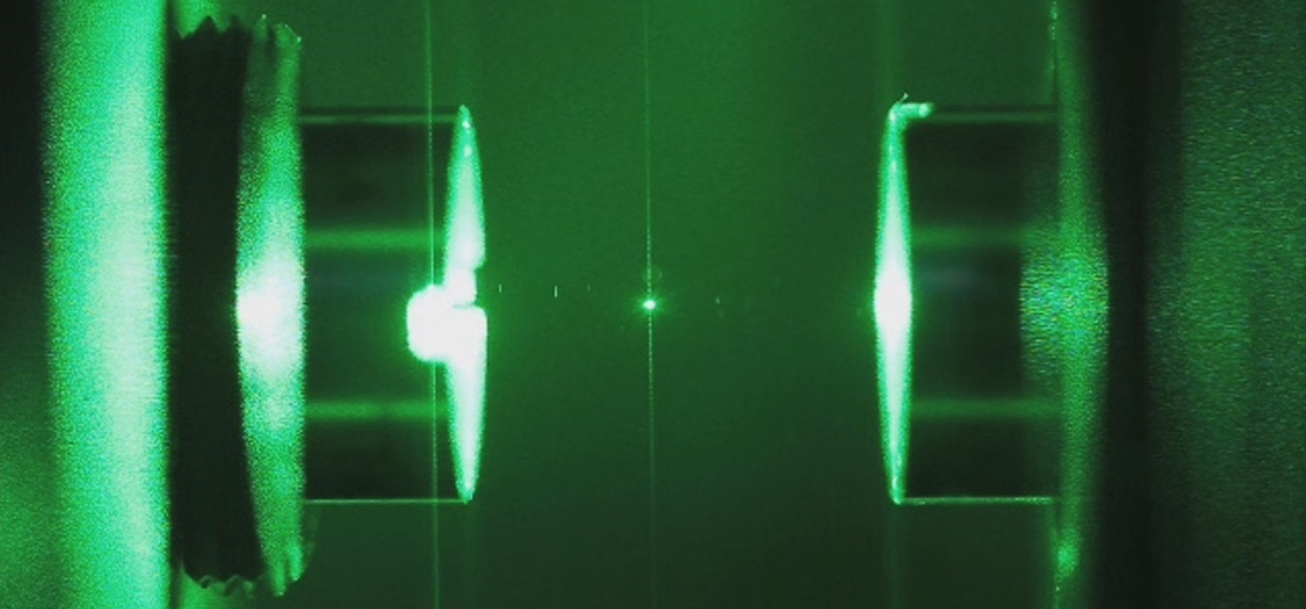
Image number 3

First, the polarization of the pickup laser was set along the cavity axis (ie, θ = 0), minimizing scattering in the empty resonator mode ( 3a ). For perfect alignment of polarizations, it is necessary to achieve complete suppression of this scattering. Scientists managed to achieve 100-fold suppression, limited by alignment between the axes of the tweezers and the resonator. As a result, coherent scattering was obtained, which gave insignificant resonator cooling along the y and z axes.

When polarization changed to θ = π / 4, scientists observed three-dimensional (along all axes of thermal motion) cooling by means of coherent scattering ( 3b ). This effect is lost during the transition to the polarization θ = π / 2. In this case, there was a good, but still weak, cooling along the x and z axes ( 3c ). The y-axis did not show similar results, which is explained by the slightly elliptical shape of the trap polarization.

For a more detailed acquaintance with the theory and practice of this experiment, I strongly recommend to look into the report of the research group .

Epilogue

Summarizing the above, we can summarize. The nanoparticle in optical tweezers is, so to speak, a lens of light in all directions. If this particle is placed inside an optical resonator, then some of this light can be stored between its mirrors. Thus, the photons will be scattered inside the resonator. If you use light whose photon energy will be less than necessary, the nanoparticle will give up part of the kinetic energy so that the photons can scatter inside the resonator. A decrease in the kinetic energy inside the particle leads to its cooling.

One of the authors of this study, Vladan Vuletic, has already conducted similar experiments, but then good results were achieved only with the use of atoms and only along one axis of thermal motion.

This method, according to the researchers, will allow much more detailed study of the quantum properties of certain nanoparticles, thereby expanding our knowledge and general understanding of the quantum world and its sometimes strange and intricate laws.

Thank you for your attention, remain curious and have a good working week, guys.

Thank you for staying with us. Do you like our articles? Want to see more interesting materials? Support us by placing an order or recommending to friends, 30% discount for Habr's users on a unique analogue of the entry-level servers that we invented for you: The whole truth about VPS (KVM) E5-2650 v4 (6 Cores) 10GB DDR4 240GB SSD 1Gbps from $ 20 or how to share the server? (Options are available with RAID1 and RAID10, up to 24 cores and up to 40GB DDR4).

VPS (KVM) E5-2650 v4 (6 Cores) 10GB DDR4 240GB SSD 1Gbps before summer for free if you pay for a period of six months, you can order here .

Dell R730xd 2 times cheaper? Only we have 2 x Intel Dodeca-Core Xeon E5-2650v4 128GB DDR4 6x480GB SSD 1Gbps 100 TV from $ 249 in the Netherlands and the USA! Read about How to build an infrastructure building. class c using servers Dell R730xd E5-2650 v4 worth 9000 euros for a penny?