In memory of Joe Polchinsky, Master of Arts
At the beginning of February 2018, the community of high-energy physicists — interested in particles, fields, strings, black holes, and the whole universe — grieved at the loss of one of the greatest theoretical physicists of our time, Joe Polchinsky . It is extremely painful for me to write these lines.
Anyone who knew him personally would miss his special qualities - a boyish smile, a weird sense of humor, a charming manner to stop at half the phrase to think, physical form and the desire for friendly competitions. Anyone familiar with his research will miss his special genius, exceptional ideas, a unique combination of abilities, which I will try to describe to you later. Those of us who are lucky to be familiar with him personally and professionally are experiencing a double loss.
Polchinski - and for all his colleagues, just Joe - possessed one of those minds that work in a magical way and betray the magic. Scientific minds differ in the same way as individuals. Each physicist has a unique combination of skills and talents (and weaknesses); in modern terms, each of us has one or two superpowers. It is rare to find two scientists with the same abilities.
Joe had several super powers, and very strong ones. He had an amazing ability to look at old tasks and see them in a new light, which often turned common sense or reformulated it in a new, clearer way. And he had amazing technical skills that allowed him to follow the paths of complex computations that would have pushed most of us to the very end.
')
Working together with Joe was one of the greatest privileges in my life — and this happened not once, but as many as four. I think that the best way for me will be to tell you about him and about several of his greatest achievements through the prism of this unforgettable experience.
Our collaborations from 1999 to 2006 represented a definite sequence aimed at understanding the stable relationship between quantum field theory — the language of particle physics — and string theory — today is best known as a candidate for the quantum theory of gravity. In each of these works, as in the many thousands of others written after 1995, Joe’s influential contribution to physics played a major role. It was the discovery of objects known as D-branes , discovered by him in the context of string theory.
I can already hear the debaters hating string theory screaming to me. “Discovery in string theory,” someone will shout, knocking on the table, “cannot be called a discovery in physics in an untested and untestable theory.” Do not pay attention to them - as you will see closer to the end of this text, they understand little.
In 1989, Joe, working with two young scientists, Gene Dai and Rob Leigh [Jin Dai and Rob Leigh], studied some of the features of string theory and did little mathematical exercises. In string theory, strings are usually small lines or loops that can move freely - like particles moving in a room. But in some cases the particles cannot move freely; it is possible, for example, to study particles trapped on the surface of a liquid or in a very thin metallic antenna. Strings may have other types of grippers that particles do not have — you can, say, fix one end, or both ends of a string on the surface, allowing the middle part of the string to move freely. The place where the end of the string can be attached - be it a point, line or surface, or something more exotic from higher dimensions - today we call it “D-brane”.
Joe and colleagues stumbled upon a treasure, they just didn't understand it right away. Looking back, they discovered that D-branes belong to the automatic properties of string theory. They are not optional; one cannot study string theories that have no D-branes. And these are not just fixed surfaces or lines. These are physical objects that can move around the world. They have a mass and they have a gravitational effect. They move and repel each other. They are as real and as important as the strings themselves!
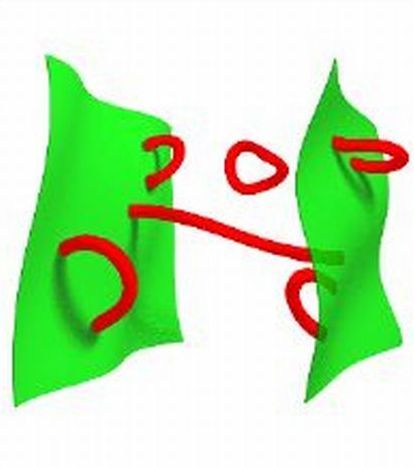
Fig. 1: D-branes (green) - physical objects on which the fundamental strings can end (red)
It turned out that Joe and his colleagues tried to understand why the chicken crossed the road [a popular beginning of the same type of anecdotes]. trans. ], and as a result discovered the existence of bicycles, cars, trucks, buses and jet planes. So it was unexpected and deep.
And yet no one, including Joe and his colleagues, fully understood what they had done. Rob Leigh, co-author Joe, sat in the neighboring office for a couple of years, and we wrote five papers with him between 1993 and 1995. At the same time, it seems to me, Rob mentioned his work on D-branes one or two times, briefly, and never explained it to me in detail. In early 1995, their work was mentioned no more than 20 times.
In 1995, the understanding of string theory stepped far forward. It was then that it became clear that all five known types of string theory are different sides of the same die - that string theory is in fact one. A whole stream of works appeared in which special black holes played a major role and their generalization — black strings, black surfaces, and so on. The relationships between them were very interesting, but often incomprehensible.
And then, in October 1995, a work appeared that changed the whole discussion forever. This Joe explained about the D-branes to us, those who barely heard of his early work, and demonstrated that many of these black holes, black strings and black surfaces were actually D-branes. Thanks to his work, all calculations have become simpler, clearer and more accurate; she immediately became a hit. By early 1996, it was mentioned 50 times; in twelve months the number of mentions was close to 300.
So what? For string theory experts, this is great, but it has no connection with the real world and experiments. Why does everyone else need it? Patience, I'm leading to this.
Today we are trying to understand how the universe works, with the help of particles. Material objects consist of atoms, they consist of electrons moving in an orbit around the nucleus; The nucleus consists of neutrons and protons. In the 1970s, we learned that protons and neutrons themselves consist of particles called quarks, antiquarks, and gluons — specifically, from a “sea” of gluons and several quark / antiquark pairs, plus three additional quarks that do not have their own antiquark pair. they are often called "valent quarks". Protons, neutrons and all other particles with three valence quarks are called " baryons ". Note that particles with one, or two, or four valence quarks do not exist - there are only baryons with three. [ they say that there are still pentaquarks - particles with five valence quarks / approx. trans. ]
In the 1950s and 1960s, physicists discovered proton and neutron-like short-lived particles with the same sea, but containing one valent quark and one valent antiquark. Particles of this type are called " mesons ". In fig. 2 I sketched a typical meson and a typical baryon. The simplest meson is called " peony "; this is the most common particle from protons produced in collisions with protons at the Large Hadron Collider.
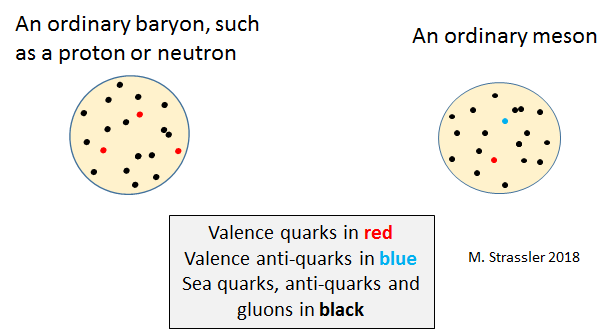
Fig. 2: red quarks, antiquarks blue; sea quarks, antiquarks and black gluons.
But in the 1960s, the fact that mesons and baryons were composed of quarks and gluons was just an idea — and she competed with the suggestion that mesons were tiny strings. I hasten to clarify that they are not strings from the “theory of everything”, which can be read in the books of Brian Green , and which are a billion billion times smaller than a proton. In the “theory of everything” strings, all types of nature particles, including electrons, photons, and Higgs bosons, are tiny strings. And now I'm talking about strings from the "theory of mesons" - not such an ambitious idea that only mesons are strings. They are much larger: their length is comparable to the diameter of the proton. For a man, this is a small size, but compared to the strings from the “theory of everything” - a giant one.
Why did people think that mesons are strings? Because that was experimental evidence ! And this evidence has not gone away after the discovery of quarks. Instead, theoretical physicists gradually understood better why quarks and gluons are able to produce mesons that behave like strings. If you unleash a meson quickly enough (and this can happen by chance in an experiment), its valence quark and antiquark can split, and a sea of objects between them form a “flow tube” (see Fig. 3). (In some superconductors, similar flow tubes can pick up magnetic fields). It looks more like a thick than a thin string, but it still has properties in common with a string, so we can get experimental results similar to the predictions of string theory.
Fig. 3
Therefore, since the mid-1970s, people have been convinced that quantum field theory, like the one that describes quarks and gluons, can produce objects that behave like strings. Many physicists - including the most famous and respected - made even more bold statements: that quantum field theory and string theory are deeply connected to each other at a fundamental level. But they could not pinpoint exactly how; they had clear evidence, but they were not completely clear and convincing.
In particular, there was an important unsolved mystery. If mesons are strings, then what are baryons? What are protons and neutrons, with their three valence quarks? How will they look if they are quickly unwound? People drew pictures a bit like pic. 3. The baryon may turn into three connected flow tubes (and one may be much longer than the other two), each of which has its own valence quarks at the end. Such a baryon would be three strings, each of which has a free end, having a common junction. This compound was called the baryon vertex [baryon vertex]. If mesons are small strings, fundamental objects in string theory, what then is a baryon top in terms of string theory? Where does she hide in the mathematics of string theory and what does it consist of?
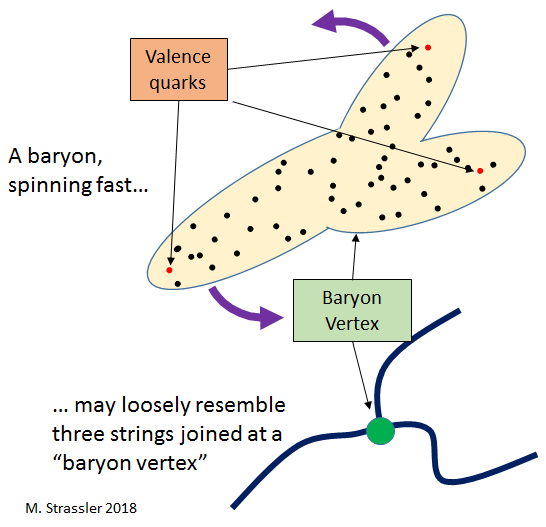
Fig. four
(Note: the top is in no way connected with quarks. This property of the sea - specifically, gluons. Therefore, in a world where there are only gluons - in a world whose strings form loops without ending - it should be possible, with enough energy, to create a pair of top / anti-vertex. Therefore, field theory predicts that these vertices should exist in the theories of closed strings, although they should be linearly bounded.)
No one knew. But isn't it interesting that the most distinctive feature of this peak was that this is the place to which the end of the string is attached?
In the period from 1997 to 2000 everything changed. After the ideas proposed by many other physicists, and using D-branes as the main tool, Juan Maldacena finally built this exact connection between quantum field theory and string theory. He was able to connect the strings with gravity and additional dimensions , which can be read in Brian Green's books, with particle physics in only three spatial dimensions, similar to the real world and in the presence of non-gravitational forces. It soon became clear that the most ambitious and radical ideas of the 1970s turned out to be correct: that almost any quantum field theory, with its particles and interactions, can be regarded as string theory. This is a bit like the same picture can be described in English or Japanese: fields / particles and strings / gravity in this context are two very different languages that speak the same thing.
The baryon top saga went on a new path in May 1998, when Ed Witten showed how a similar top appears in the examples of Maldacena. It is not surprising that this vertex was a D-brane — specifically, a D-particle, an object on which strings may end, extending from freely moving quarks. This result was not completely satisfactory, because the gluons and quarks from the Maldacena examples move freely without forming mesons or baryons. Accordingly, a baryon top is not a physical object; if done, it quickly dissolves into nothing. However, from Whitten's work, it became obvious what was happening. To the extent that real mesons can be considered as strings, real protons and neutrons can be viewed as strings connected to a D-brane.
More realistic examples found by theorists did not have to wait long. I do not remember who was the first, but I know that one of the earliest examples appeared in our work with Joe in 2000.
This project appeared during my visit in September 1999 to KITP (Kavli Institute of Theoretical Physics) in Santa Barbara, where Joe worked. Shortly before this, it turned out that I studied field theory called N = 1 *, which was only slightly different from the examples of Maldasena, in which meson-like objects could form. One of the first reports I heard on my arrival at KITP was a report by Rob Myers about the strange property of D-branes that he discovered. During the report, I was struck by the connection between the observation of Myers and one of the properties of the theory N = 1 *, and I experienced a moment of enlightenment for which physicists live. I suddenly realized how string theory should look, describing field theory N = 1 *.
But I did not like this answer. It became obvious that detailed calculations would be extremely difficult and would require the use of aspects of string theory, about which I knew almost nothing (non-holomorphic warped branes in the warped geometry of higher dimensions). All that I could hope for working alone is to write conceptual work with a bunch of pictures and with a predominance of hypotheses over provable facts.
But I was at KITP. Joe and I had a good rapport for some time, and I knew that the same questions seemed interesting to us. Joe was also a master of bran; he knew all about the D-branes. So I decided that the best way out for me would be to convince Jo to join the work. I launched into persistent pleading, and, fortunately, it worked.
I returned to the east coast, and Joe and I got to work. Every one to two weeks Joe sent me research notes with preliminary calculations on string theory. Their level of technical complexity was so high, and there were so few learning moments in them that I felt like a child; I could hardly understand what was going on. We moved slowly. Joe did important preliminary calculations, but it was very difficult for me to keep track of them. And if the preliminary calculations for string theory were so complex, could we hope to solve the whole problem? Even Joe was a little worried.
One day I received a message full of victorious clucking — something like “we made them!”, A mood that anyone familiar with Joe could recognize. Using a terrific trick, he figured out how to use his preliminary calculations to facilitate the complete task! Instead of months of hard work, it turned out that we were almost done.
And from that moment on, the work became very interesting! Almost every week has evolved like this. I reflected on the phenomenon known to me from quantum field theory, which should be described from the point of view of string theory, such as the baryon vertex. I knew about D-branes enough to work out a heuristic proof of how it should look. I called Joe, told him about it, and possibly sent him sketches. A few days later an email came in a set of notes containing full calculations confirming this phenomenon. Each calculation was unique, precious, including a characteristic study of D-branes of an exotic form, located in a curved space. The spirit captured the observation of the speed with which Joe worked, the breadth and depth of his mathematical talent, his incomparable understanding of these branes.
Over the years of our joint work, when we wanted to delve into the equations, it was always like this; Joe inevitably left me far behind, shaking his head in amazement. This is my drawback - for a physicist, I am quite a mean computation. But Joe was incredibly good at it.
Fortunately for me, it was possible to enjoy teamwork, because I could almost always withstand Joe’s tempo in the area of conceptual problems, and sometimes overtook him. Among my favorite memories as a scientist, there are moments when I taught Joe something that he did not know; he paused for a few seconds, nodded quickly, and, sorting out the problem, made a close look, squinting his eyes and opening his mouth. “Aha, aha,” he would say.
Another face Joe opened while working on our second scientific work. We were standing in the KITP hall, talking on a new topic, and even before we decided on exactly which question we would work on, Joe suddenly guessed the answer! And I could not make him explain what task he had solved, let alone pull out a solution, within a few days! It was confusing.
This was another classic Joe ability. Sometimes he knew that he had found a solution (and almost always turned out to be right), but he could not say anything definite about it until he thought a few days and turned his ideas into equations. During our collaboration this happened several times. (I never told him "Use the words, Joe," but, apparently, it was necessary). His mind somehow worked in areas not subject to language, and, like none of us, being outside his brain, would not understand. It was something of an oracle.
After 2006, our interests gradually dispersed; I concentrated on the Large Hadron Collider (also known as the Large D-Branded Collider), and after several studies Jo decided to take up the horizons of black holes and the information paradox . But I enjoyed his work at a distance, especially when in 2012 Joe and three colleagues (Ahmed Almeyri, Don Marolph and James Sally) blew up the idea of complementarity of black holes, which many pinned their hopes on by solving the information paradox. The remnants of this idea are still smoking, but the paradox has not gone away.
Then Joe got sick, and we started losing him - at a too young age. One of his last gifts for us was his memoirs, from which each of us learned something about him that we did not know before. Finally, he crossed the horizon, because of which they did not return. If there is no firewall, then he finally found out.
Can we think about what will happen to Joe’s scientific legacy in a few decades? It is difficult to foresee how the theorist’s work will be assessed in a hundred years; sometimes changes occur in an unexpected direction, and what seems unimportant now may become the main thing in the future - as was the case with the D-branes themselves during the 1990s. For those who work with them today, D-branes in string theory are clearly Joe’s most important discovery - although his contribution to our understanding of black holes, cosmic strings and aspects of field theory will not be forgotten soon, perhaps never. But who knows? By 2100, string theory can turn out to be both a generally accepted theory of gravity, and a little-known tool for studying quantum fields.
But even if the last one happens, I still suspect that Joe will be remembered for D-branes. Because - as I tried to prove - they are real. Actually real. In each proton, in each neutron, there is one. Our bodies contain their billions of billions of billions. For this idea, for this elementary contribution to human knowledge, our descendants should blame Joseph Polchinsky.
Thanks for everything, Joe. We will be terribly missed. You have so often taught us new ways to look at the world, and even at ourselves.
Anyone who knew him personally would miss his special qualities - a boyish smile, a weird sense of humor, a charming manner to stop at half the phrase to think, physical form and the desire for friendly competitions. Anyone familiar with his research will miss his special genius, exceptional ideas, a unique combination of abilities, which I will try to describe to you later. Those of us who are lucky to be familiar with him personally and professionally are experiencing a double loss.Polchinski - and for all his colleagues, just Joe - possessed one of those minds that work in a magical way and betray the magic. Scientific minds differ in the same way as individuals. Each physicist has a unique combination of skills and talents (and weaknesses); in modern terms, each of us has one or two superpowers. It is rare to find two scientists with the same abilities.
Joe had several super powers, and very strong ones. He had an amazing ability to look at old tasks and see them in a new light, which often turned common sense or reformulated it in a new, clearer way. And he had amazing technical skills that allowed him to follow the paths of complex computations that would have pushed most of us to the very end.
')
Working together with Joe was one of the greatest privileges in my life — and this happened not once, but as many as four. I think that the best way for me will be to tell you about him and about several of his greatest achievements through the prism of this unforgettable experience.
Our collaborations from 1999 to 2006 represented a definite sequence aimed at understanding the stable relationship between quantum field theory — the language of particle physics — and string theory — today is best known as a candidate for the quantum theory of gravity. In each of these works, as in the many thousands of others written after 1995, Joe’s influential contribution to physics played a major role. It was the discovery of objects known as D-branes , discovered by him in the context of string theory.
I can already hear the debaters hating string theory screaming to me. “Discovery in string theory,” someone will shout, knocking on the table, “cannot be called a discovery in physics in an untested and untestable theory.” Do not pay attention to them - as you will see closer to the end of this text, they understand little.
Great discovery
In 1989, Joe, working with two young scientists, Gene Dai and Rob Leigh [Jin Dai and Rob Leigh], studied some of the features of string theory and did little mathematical exercises. In string theory, strings are usually small lines or loops that can move freely - like particles moving in a room. But in some cases the particles cannot move freely; it is possible, for example, to study particles trapped on the surface of a liquid or in a very thin metallic antenna. Strings may have other types of grippers that particles do not have — you can, say, fix one end, or both ends of a string on the surface, allowing the middle part of the string to move freely. The place where the end of the string can be attached - be it a point, line or surface, or something more exotic from higher dimensions - today we call it “D-brane”.
Joe and colleagues stumbled upon a treasure, they just didn't understand it right away. Looking back, they discovered that D-branes belong to the automatic properties of string theory. They are not optional; one cannot study string theories that have no D-branes. And these are not just fixed surfaces or lines. These are physical objects that can move around the world. They have a mass and they have a gravitational effect. They move and repel each other. They are as real and as important as the strings themselves!
Fig. 1: D-branes (green) - physical objects on which the fundamental strings can end (red)
It turned out that Joe and his colleagues tried to understand why the chicken crossed the road [a popular beginning of the same type of anecdotes]. trans. ], and as a result discovered the existence of bicycles, cars, trucks, buses and jet planes. So it was unexpected and deep.
And yet no one, including Joe and his colleagues, fully understood what they had done. Rob Leigh, co-author Joe, sat in the neighboring office for a couple of years, and we wrote five papers with him between 1993 and 1995. At the same time, it seems to me, Rob mentioned his work on D-branes one or two times, briefly, and never explained it to me in detail. In early 1995, their work was mentioned no more than 20 times.
In 1995, the understanding of string theory stepped far forward. It was then that it became clear that all five known types of string theory are different sides of the same die - that string theory is in fact one. A whole stream of works appeared in which special black holes played a major role and their generalization — black strings, black surfaces, and so on. The relationships between them were very interesting, but often incomprehensible.
And then, in October 1995, a work appeared that changed the whole discussion forever. This Joe explained about the D-branes to us, those who barely heard of his early work, and demonstrated that many of these black holes, black strings and black surfaces were actually D-branes. Thanks to his work, all calculations have become simpler, clearer and more accurate; she immediately became a hit. By early 1996, it was mentioned 50 times; in twelve months the number of mentions was close to 300.
So what? For string theory experts, this is great, but it has no connection with the real world and experiments. Why does everyone else need it? Patience, I'm leading to this.
How does this relate to nature?
Today we are trying to understand how the universe works, with the help of particles. Material objects consist of atoms, they consist of electrons moving in an orbit around the nucleus; The nucleus consists of neutrons and protons. In the 1970s, we learned that protons and neutrons themselves consist of particles called quarks, antiquarks, and gluons — specifically, from a “sea” of gluons and several quark / antiquark pairs, plus three additional quarks that do not have their own antiquark pair. they are often called "valent quarks". Protons, neutrons and all other particles with three valence quarks are called " baryons ". Note that particles with one, or two, or four valence quarks do not exist - there are only baryons with three. [ they say that there are still pentaquarks - particles with five valence quarks / approx. trans. ]
In the 1950s and 1960s, physicists discovered proton and neutron-like short-lived particles with the same sea, but containing one valent quark and one valent antiquark. Particles of this type are called " mesons ". In fig. 2 I sketched a typical meson and a typical baryon. The simplest meson is called " peony "; this is the most common particle from protons produced in collisions with protons at the Large Hadron Collider.
Fig. 2: red quarks, antiquarks blue; sea quarks, antiquarks and black gluons.
But in the 1960s, the fact that mesons and baryons were composed of quarks and gluons was just an idea — and she competed with the suggestion that mesons were tiny strings. I hasten to clarify that they are not strings from the “theory of everything”, which can be read in the books of Brian Green , and which are a billion billion times smaller than a proton. In the “theory of everything” strings, all types of nature particles, including electrons, photons, and Higgs bosons, are tiny strings. And now I'm talking about strings from the "theory of mesons" - not such an ambitious idea that only mesons are strings. They are much larger: their length is comparable to the diameter of the proton. For a man, this is a small size, but compared to the strings from the “theory of everything” - a giant one.
Why did people think that mesons are strings? Because that was experimental evidence ! And this evidence has not gone away after the discovery of quarks. Instead, theoretical physicists gradually understood better why quarks and gluons are able to produce mesons that behave like strings. If you unleash a meson quickly enough (and this can happen by chance in an experiment), its valence quark and antiquark can split, and a sea of objects between them form a “flow tube” (see Fig. 3). (In some superconductors, similar flow tubes can pick up magnetic fields). It looks more like a thick than a thin string, but it still has properties in common with a string, so we can get experimental results similar to the predictions of string theory.
Fig. 3
Therefore, since the mid-1970s, people have been convinced that quantum field theory, like the one that describes quarks and gluons, can produce objects that behave like strings. Many physicists - including the most famous and respected - made even more bold statements: that quantum field theory and string theory are deeply connected to each other at a fundamental level. But they could not pinpoint exactly how; they had clear evidence, but they were not completely clear and convincing.
In particular, there was an important unsolved mystery. If mesons are strings, then what are baryons? What are protons and neutrons, with their three valence quarks? How will they look if they are quickly unwound? People drew pictures a bit like pic. 3. The baryon may turn into three connected flow tubes (and one may be much longer than the other two), each of which has its own valence quarks at the end. Such a baryon would be three strings, each of which has a free end, having a common junction. This compound was called the baryon vertex [baryon vertex]. If mesons are small strings, fundamental objects in string theory, what then is a baryon top in terms of string theory? Where does she hide in the mathematics of string theory and what does it consist of?
Fig. four
(Note: the top is in no way connected with quarks. This property of the sea - specifically, gluons. Therefore, in a world where there are only gluons - in a world whose strings form loops without ending - it should be possible, with enough energy, to create a pair of top / anti-vertex. Therefore, field theory predicts that these vertices should exist in the theories of closed strings, although they should be linearly bounded.)
No one knew. But isn't it interesting that the most distinctive feature of this peak was that this is the place to which the end of the string is attached?
In the period from 1997 to 2000 everything changed. After the ideas proposed by many other physicists, and using D-branes as the main tool, Juan Maldacena finally built this exact connection between quantum field theory and string theory. He was able to connect the strings with gravity and additional dimensions , which can be read in Brian Green's books, with particle physics in only three spatial dimensions, similar to the real world and in the presence of non-gravitational forces. It soon became clear that the most ambitious and radical ideas of the 1970s turned out to be correct: that almost any quantum field theory, with its particles and interactions, can be regarded as string theory. This is a bit like the same picture can be described in English or Japanese: fields / particles and strings / gravity in this context are two very different languages that speak the same thing.
The baryon top saga went on a new path in May 1998, when Ed Witten showed how a similar top appears in the examples of Maldacena. It is not surprising that this vertex was a D-brane — specifically, a D-particle, an object on which strings may end, extending from freely moving quarks. This result was not completely satisfactory, because the gluons and quarks from the Maldacena examples move freely without forming mesons or baryons. Accordingly, a baryon top is not a physical object; if done, it quickly dissolves into nothing. However, from Whitten's work, it became obvious what was happening. To the extent that real mesons can be considered as strings, real protons and neutrons can be viewed as strings connected to a D-brane.
More realistic examples found by theorists did not have to wait long. I do not remember who was the first, but I know that one of the earliest examples appeared in our work with Joe in 2000.
Working with Joe
This project appeared during my visit in September 1999 to KITP (Kavli Institute of Theoretical Physics) in Santa Barbara, where Joe worked. Shortly before this, it turned out that I studied field theory called N = 1 *, which was only slightly different from the examples of Maldasena, in which meson-like objects could form. One of the first reports I heard on my arrival at KITP was a report by Rob Myers about the strange property of D-branes that he discovered. During the report, I was struck by the connection between the observation of Myers and one of the properties of the theory N = 1 *, and I experienced a moment of enlightenment for which physicists live. I suddenly realized how string theory should look, describing field theory N = 1 *.
But I did not like this answer. It became obvious that detailed calculations would be extremely difficult and would require the use of aspects of string theory, about which I knew almost nothing (non-holomorphic warped branes in the warped geometry of higher dimensions). All that I could hope for working alone is to write conceptual work with a bunch of pictures and with a predominance of hypotheses over provable facts.
But I was at KITP. Joe and I had a good rapport for some time, and I knew that the same questions seemed interesting to us. Joe was also a master of bran; he knew all about the D-branes. So I decided that the best way out for me would be to convince Jo to join the work. I launched into persistent pleading, and, fortunately, it worked.
I returned to the east coast, and Joe and I got to work. Every one to two weeks Joe sent me research notes with preliminary calculations on string theory. Their level of technical complexity was so high, and there were so few learning moments in them that I felt like a child; I could hardly understand what was going on. We moved slowly. Joe did important preliminary calculations, but it was very difficult for me to keep track of them. And if the preliminary calculations for string theory were so complex, could we hope to solve the whole problem? Even Joe was a little worried.
One day I received a message full of victorious clucking — something like “we made them!”, A mood that anyone familiar with Joe could recognize. Using a terrific trick, he figured out how to use his preliminary calculations to facilitate the complete task! Instead of months of hard work, it turned out that we were almost done.And from that moment on, the work became very interesting! Almost every week has evolved like this. I reflected on the phenomenon known to me from quantum field theory, which should be described from the point of view of string theory, such as the baryon vertex. I knew about D-branes enough to work out a heuristic proof of how it should look. I called Joe, told him about it, and possibly sent him sketches. A few days later an email came in a set of notes containing full calculations confirming this phenomenon. Each calculation was unique, precious, including a characteristic study of D-branes of an exotic form, located in a curved space. The spirit captured the observation of the speed with which Joe worked, the breadth and depth of his mathematical talent, his incomparable understanding of these branes.
Over the years of our joint work, when we wanted to delve into the equations, it was always like this; Joe inevitably left me far behind, shaking his head in amazement. This is my drawback - for a physicist, I am quite a mean computation. But Joe was incredibly good at it.
Fortunately for me, it was possible to enjoy teamwork, because I could almost always withstand Joe’s tempo in the area of conceptual problems, and sometimes overtook him. Among my favorite memories as a scientist, there are moments when I taught Joe something that he did not know; he paused for a few seconds, nodded quickly, and, sorting out the problem, made a close look, squinting his eyes and opening his mouth. “Aha, aha,” he would say.
Another face Joe opened while working on our second scientific work. We were standing in the KITP hall, talking on a new topic, and even before we decided on exactly which question we would work on, Joe suddenly guessed the answer! And I could not make him explain what task he had solved, let alone pull out a solution, within a few days! It was confusing.
This was another classic Joe ability. Sometimes he knew that he had found a solution (and almost always turned out to be right), but he could not say anything definite about it until he thought a few days and turned his ideas into equations. During our collaboration this happened several times. (I never told him "Use the words, Joe," but, apparently, it was necessary). His mind somehow worked in areas not subject to language, and, like none of us, being outside his brain, would not understand. It was something of an oracle.
Watching the horizon
After 2006, our interests gradually dispersed; I concentrated on the Large Hadron Collider (also known as the Large D-Branded Collider), and after several studies Jo decided to take up the horizons of black holes and the information paradox . But I enjoyed his work at a distance, especially when in 2012 Joe and three colleagues (Ahmed Almeyri, Don Marolph and James Sally) blew up the idea of complementarity of black holes, which many pinned their hopes on by solving the information paradox. The remnants of this idea are still smoking, but the paradox has not gone away.
Then Joe got sick, and we started losing him - at a too young age. One of his last gifts for us was his memoirs, from which each of us learned something about him that we did not know before. Finally, he crossed the horizon, because of which they did not return. If there is no firewall, then he finally found out.
Can we think about what will happen to Joe’s scientific legacy in a few decades? It is difficult to foresee how the theorist’s work will be assessed in a hundred years; sometimes changes occur in an unexpected direction, and what seems unimportant now may become the main thing in the future - as was the case with the D-branes themselves during the 1990s. For those who work with them today, D-branes in string theory are clearly Joe’s most important discovery - although his contribution to our understanding of black holes, cosmic strings and aspects of field theory will not be forgotten soon, perhaps never. But who knows? By 2100, string theory can turn out to be both a generally accepted theory of gravity, and a little-known tool for studying quantum fields.
But even if the last one happens, I still suspect that Joe will be remembered for D-branes. Because - as I tried to prove - they are real. Actually real. In each proton, in each neutron, there is one. Our bodies contain their billions of billions of billions. For this idea, for this elementary contribution to human knowledge, our descendants should blame Joseph Polchinsky.
Thanks for everything, Joe. We will be terribly missed. You have so often taught us new ways to look at the world, and even at ourselves.
Source: https://habr.com/ru/post/410793/
All Articles