The beauty of numbers. How to quickly calculate in mind

Old record on the receipt of payment of the tax ("yasaka"). It means the amount of 1232 rubles. 24 kopecks Illustration from the book: Jacob Perelman “Entertaining arithmetic”

More Richard Feynman in the book “ You are kidding of course, Mr. Feynman! »Told several techniques of oral account. Although these are very simple tricks, they are not always included in the school curriculum.

For example, to quickly square the number X around 50 (50 ² = 2500), you need to subtract / add a hundred for each unit the difference between 50 and X, and then add the difference in the square. The description sounds much more complicated than the actual calculation.

52 ² = 2500 + 200 + 4
47 ² = 2500 - 300 + 9
58 ² = 2500 + 800 + 64

Young Feynman was taught this trick by fellow physicist Hans Bethe, who also worked at Los Alamos at the time on the Manhattan project.
')
Hans showed a few tricks he used for quick calculations. For example, for calculating cubic roots and exponentiation, it is convenient to remember the logarithm table. This knowledge greatly simplifies complex arithmetic operations. For example, calculate in mind the approximate value of a cubic root of 2.5. In fact, with such calculations, a kind of slide rule works in your head, in which the multiplication and division of numbers is replaced by the addition and subtraction of their logarithms. The most convenient thing.


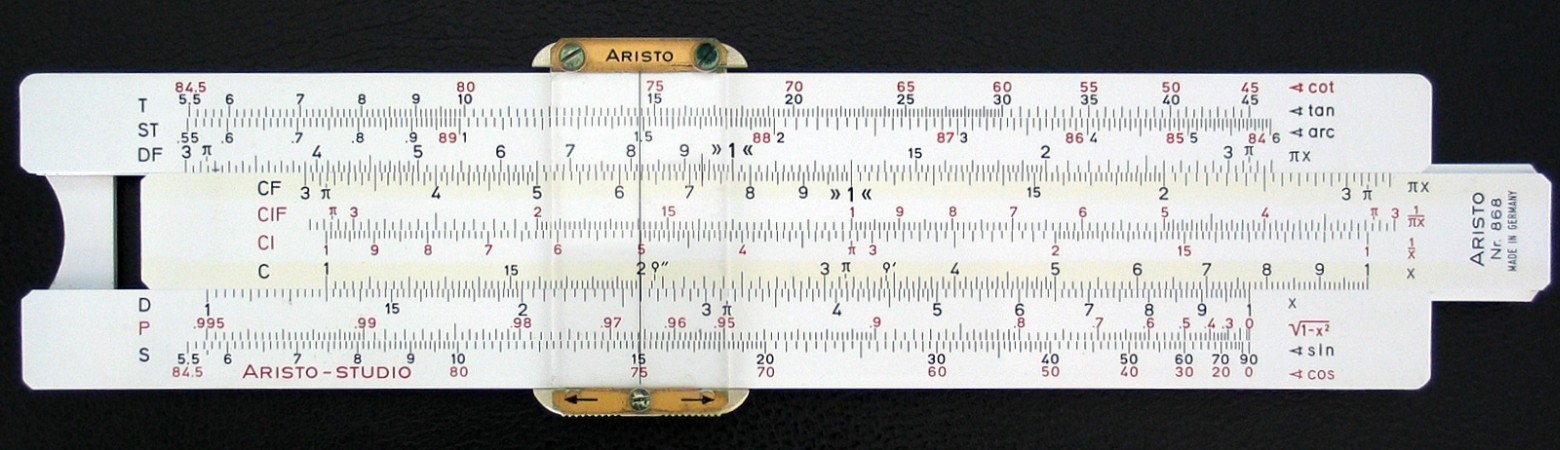
Logarithmic ruler

Before the advent of computers and calculators, a logarithmic line was used everywhere. This is a kind of analog "computer" that allows you to perform several mathematical operations, including multiplication and dividing numbers, squaring and cube, calculating square and cubic roots, calculating logarithms, potentiating, calculating trigonometric and hyperbolic functions and some other operations. If we divide the calculation into three actions, then with the help of a slide rule, you can raise numbers to any real degree and extract the root of any real degree. The accuracy of the calculations is about 3 significant digits.

In order to quickly carry out complex calculations in the mind even without a slide rule, it is not bad to remember the squares of all numbers, at least up to 25, simply because they are often used in calculations. And the table of degrees - the most common. It is easier to remember than to calculate again each time, that 5 ⁴ = 625, 3 ⁵ = 243, 2 ²⁰ = 1 048 576, and √3 ≈ 1.732.

Richard Feynman improved his skills and gradually noticed all the new interesting patterns and relationships between the numbers. He gives the following example: “If someone started dividing 1 by 1.73, you could immediately answer that it would be 0.577, because 1.73 is a number close to the square root of three. Thus, 1 / 1.73 is about one third of the square root of 3. "

Such an advanced oral account could surprise colleagues at a time when there were no computers and calculators. In those days, absolutely all scientists knew how to count well in their minds, so to achieve mastery it was necessary to dive deep enough into the world of numbers.

Nowadays, people take out a calculator to simply divide 76 by 3. It has become much easier to surprise others. At the time of Feynman, instead of a calculator, there were wooden accounts, on which one could also perform complex operations, including taking cubic roots. The great physicist had already noticed that using such tools, people do not need to memorize many arithmetic combinations at all, but simply learn how to roll the balls correctly. That is, people with brain expanders do not know the numbers. They are worse at coping with tasks in "offline" mode.

Here are five very simple oral account tips that are recommended by Jacob Perelman in the “ Fast Score ” manual of the publishing house, 1941.

1. If one of the multiplied numbers is factorized, it is convenient to multiply them sequentially.

225 × 6 = 225 × 2 × 3 = 450 × 3
147 × 8 = 147 × 2 × 2 × 2, i.e., double the result three times

2. When multiplying by 4, it is enough to double the result twice. Similarly, when divided by 4 and 8, the number is halved twice or three times.

3. When multiplying by 5 or 25, the number can be divided by 2 or 4, and then one or two zeros can be added to the result.

74 × 5 = 37 × 10
72 × 25 = 18 × 100

Here it is better to immediately evaluate how easy it is. For example, 31 × 25 is more convenient to multiply as 25 × 31 in a standard way, that is, as 750 + 25, and not as 31 × 25, that is, 7.75 × 100.

When multiplying by a number close to a round (98, 103), it is convenient to immediately multiply by a round number (100), and then subtract / add the product of the difference.

37 × 98 = 3700 - 74
37 × 104 = 3700 + 148

4. To square the number ending in 5 (for example, 85), multiply the number of tens (8) by one plus one (9), and is credited with 25.

8 × 9 = 72, we assign 25, so that 85 ² = 7225

Why this rule works is evident from the formula:

(10X + 5) ² = 100X ² + 100X + 25 = 100X (X + 1) + 25

The trick applies to decimals that end in 5:

8.5 ² = 72.25
14.5 ² = 210.25
0.35 ² = 0.1225

5. When building in a square, do not forget about a convenient formula.

(a + b) ² = a ² + b ² + 2ab
44 ² = 1600 + 16 + 320

Of course, all methods can be combined with each other, creating more convenient and effective techniques for specific situations.