The power of known physical interactions
In this article I want to discuss the basic properties of the known interactions - the four observables and the fifth - the new - about whose existence we draw a conclusion from the discovery of the Higgs particle.
Specifically, I want to discuss what particle physics specialists have in mind when describing interactions as weak or strong. You can come across such terminology often, but if no one has explained it to you, it is impossible to guess what it means. So here is an explanation for you - albeit a long one, but I hope it will open your eyes to how nature works, and also raise many new questions that I hope to answer later.
What do these terms mean? In ordinary life, we would imagine that a strong interaction can lift us into the air, and we can cope with the weak by tightening our muscles a little. But experts in particle physics do not mean that.
')
Speaking of the strong and the weak, physicists do not mean the absolute strength or weakness of the interaction. We are not talking about whether the interaction can break a window or hold a gold bar. In this context, the terms “strong” and “weak” are not entirely absolute, in the sense in which we use them in everyday life or even in elementary classes in physics. This terminology has emerged due to a deep understanding of quantum field theory, a modern mathematical language used to describe known elementary particles and forces. But it is fundamental to the modern discussion of these problems by physicists. So I will start with the rationale for the occurrence of such terms.
Take a pair of objects of a certain type, say, elementary particles, and place them at a distance r from each other. Suppose each has an effect F on the other. Then we say that the impact is weak if
Where h is Planck's constant, c is the speed of light. Often in physics it is convenient to use not h, but
In short, in particle physics:
• For weak interaction
• For strong interaction
Usually, even in theoretical studies, we do not encounter interactions much stronger . Such power makes them so complex that we work with them in a different way. But it is a long story.
It turns out that this characteristic is not talking about the absolute strength or weakness of the interaction, but about whether it is strong or weak compared to typical interactions operating at a distance r. It is not the interaction itself that is taken into account; interaction multiplied by the square of the distance is taken into account, and this value is compared with ℏ c.
To explain the usefulness of this concept, I will give an illustration for the case of electromagnetic interactions acting on simple charged particles - electrons, positrons and protons. The electric charge of electrons is –e; in protons and positrons, the charge is + e.
First, imagine two motionless protons, each of mass m and an electric charge + e, located at a distance r from each other. Electric force pushes them apart, and its value is given by the formula
The same formula is applicable for two electrons with a –e charge. For an electron and a positron, the interaction will be the same, only it will attract them and not push them apart.
What is k? This is the Coulomb constant , and its value depends on how to determine e, the basic unit of charge. But this is unimportant, since when discussing electrical interactions and elementary particles, we will always see the joint appearance of ke 2 . We don't need to know how big k is, we just need to know how big ke 2 is ?
It turns out that if r is greater than a millionth millionth of a meter, then ke 2 is approximately equal to 0.007, multiplied by (hc / 2π), where h is Planck's constant, and c is the speed of light. Therefore, we can write the electric force multiplied by r 2 as approximately equal to
Since 0.007 is much less than 1, electromagnetism is a weak interaction, and remains so at all distances measured by us.
It is very important not to get confused! Only from the fact that electromagnetism is a weak interaction, it does not follow that the interaction of two protons is weak in absolute terms. In fact, the electrical force trying to push two protons into the helium nucleus can be compared to the weight of a truck! And all this force acts on two tiny pieces! But for such small distances, this effect is rather weak, and a stronger interaction (“strong nuclear interaction”) resists electromagnetic repulsion, keeping protons and neutrons in the helium nucleus together.
By the way, for this value of 0.007 there is a historical name; it is called the fine structure constant (since it sets the size of small differences in the energies of different configurations of atoms), and is usually denoted α:
This is one of the most accurately measured quantities of nature. Often, people write it approximately equal to 1/137 (and for many years some scientists thought that the number 137 is some kind of special), but if you do it quite accurately, then you will have to write down 1 / 137.0359990 ...
So why does the fact that α is much less than 1 indicate that this interaction should be written down as weak and not as strong?
Why does α << 1 mean that the electromagnetic interaction is weak
The easiest way to show this is with an example in which force attracts particles — for example, an electron and a positron, or an electron and a proton. It is easier to start with an electron and a positron, since they have equal masses; they form a state similar to an atom called positronium , similar to the hydrogen atom formed by an electron and a proton, but more symmetrical, in which two particles orbit each other around each other. In a hydrogen atom, an electron moves in an orbit around a practically stationary proton. In fact, the formulas for hydrogen are also suitable for positronium, with small changes (differ by a factor of 2) in several places. (Yes, an electron and positron in positronium eventually annihilate and turn into two or three photons, but only after the particles make many billions of revolutions - which, however, takes a fraction of a second). For positronium in the lowest energy state:
• The typical speed of each particle is α / 2 × c;
• typical energy of motion (kinetic) of each particle is mc 2 × α 2/8;
the interaction energy (potential) of two particles is –mc 2 × α 2/2;
• the binding energy B of the positronium (the sum of the energy of motion and the energy of interaction) is equal to mc 2 × α 2/4;
• energy of the mass of the positronium 2 mc 2 - B; and since the second is much smaller than the first, the mass of the atom is only slightly less than the sum of the masses of the electron and positron.
In short, due to the fact that α is much less than 1, there are three important, interconnected facts:
• Electron and positron move at speeds comparable to the speed of light c.
• The kinetic energy, the potential energy and the binding energy B are small compared to the mass energy of the electron and positron, E = mc 2 .
• The mass of positronium is very close to the sum of the masses of the electron and positron.
All these statements are true regardless of how large or small the electron mass is; they depend only on a small value of α.
All this together means that for describing this atom-like state, Einstein’s special theory of relativity is not important. Newton's laws of motion are well suited for predictions, down to details not larger than α — that is, with an accuracy of 1% or better. And, as we will see later, this means that the system is relatively simple. It can be described using quantum mechanics with a fairly simple mathematics, without the participation of quantum field theory, which would have been necessary if SRT was important. The mathematics of the hydrogen atom is the same as that of the positronium, and it is so simple that physicists get to know it at the institute, in the first lessons on quantum mechanics.
This can be thought of in another useful, albeit less well-known way. It must be remembered that electrons, like all elementary particles, are in reality quanta — tiny perturbations of quantum fields. They are more like waves than small balls. Accordingly, they vibrate, like all waves: they have a frequency of vibrations. The time that passes from one vibration to another — which I like to call poetically “heartbeat” —is equal to hc / m. If α is small, then the time required for the light to cross the atom-like state is much more than 1 / α times than the heartbeat of the particles it contains. In this sense, positronium is quite large. And since the particles themselves move much slower than light, the particles take even more time to intersect this atom-like state — something in the region of 1 / α 2 heartbeats.
When α is small, other things that could be more complex are also simplified. For example, the effect of a positron on an electron can cause an electron to turn into a virtual electron and a virtual photon - sometimes not for long. (Virtual “particles” are not particles; a real particle is a well-behaved quantum field wave, and a virtual one is a more general perturbation of these fields). But this rarely happens when α is small. More rarely, the virtual photon itself is perturbed and turns into a virtual electron and positron. Since the energy of 2mc 2 needed to obtain a real electron and positron is nowhere to take (remember that the energies of motion and interactions are much smaller), the virtual electron and positron appear very rarely. The fact that virtual particles appear rarely allows us to say that “the positronium atom consists of an electron and a positron” —that is, most of the time. Only in very precise calculations is it necessary to be more careful and remember that this is not always the case. The same works for the hydrogen atom: it is (almost all the time) only one electron and one proton, held by simple electrical interaction.
Now imagine that α gradually grows and approaches 1. What happens to the positronium?
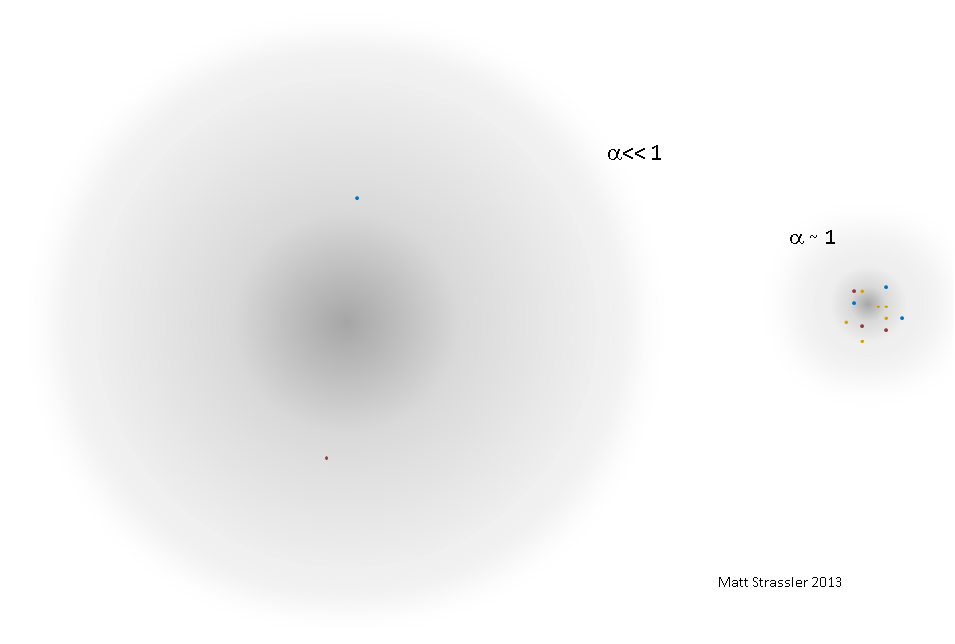
Fig. one
With increasing α, the interaction (at any distance) between the electron and the positron becomes stronger, and since they attract more strongly, the particles in an atom-like state move closer. Particles move faster, approaching the speed of light. The energy of motion of the particles increases, the value of the interaction energy increases, as the binding energy grows - and approaches 2m. Accordingly, the mass of the atom-like state is no longer equal to about 2m. The size of the atom-like state becomes smaller; the time required for its intersection by light, the time required for its intersection by particles, and the time elapsed between two heartbeats of particles begin to be compared with each other.
The increased interaction of the electron and positron leads to a more frequent appearance of virtual photons; the presence of a larger amount of energy in an atom facilitates the transformation of a virtual photon into a virtual electron and positron. When this happens, it becomes difficult to say which electron is real and which is virtual, since powerful forces also act between two electrons, as well as between an electron and any of the positrons. This can lead to the fact that the particle, being real, becomes virtual, and makes the virtual particle real - and vice versa. Meanwhile, virtual electrons and positrons can also emit or absorb photons, which can be both virtual and real.
The very separation between real and virtual particles becomes more difficult to hold. Real particles must be properly disturbed quantum field perturbations. But the atom-like state is so small that an electron and a positron take only one heartbeat to cross, and at that moment powerful interactions will force them to change direction. How can we show that such a particle is like a well-behaved indignation? A well-behaved wave must worry for a while — a few heartbeats — before external forces begin to influence it. And here our electron, although it is more like a real particle than a virtual one, is still strongly distorted, and no longer fits the definition of a “real particle”. And this electron may not exist for long. The appearance of a virtual electron-positron pair can be followed by the annihilation of a former real electron with a newly formed positron, after which a real / possibly virtual electron will remain.
So, instead of having a small α - a simple system with a mass slightly less than 2m, consisting of an electron and a positron moving at speeds much less than light - as α approaches 1, we find an extremely complex system in which many particles move with near-light speeds, with a mass that is very different from 2m (see Fig. 1). It is impossible to say how many particles are inside - will we only consider real ones? If so, how to distinguish precisely the almost real from the almost virtual? The number of real particles can constantly change.
This is what characterizes a really strong interaction; the objects it forms are much more complex than atoms. Scientists, in a sense, were lucky that the first objects encountered on the way to quantum field theory were atoms. They are weak interaction - electromagnetic force - and they were easy to understand with the help of simple mathematics of quantum mechanics, in which the number of particles is constant. Protons, on the other hand, retain a strong interaction - a strong nuclear interaction. Therefore, it is not surprising that the structure of protons is much, much more complicated than that of atoms. The number of particles inside a proton is constantly changing - and this requires a much more complex mathematics of quantum field theory.
By the way, the electrical interaction between two electrons is weak due to the fact that α is small. The same is true for interactions between two elementary particles, since the charges of all known particles are in the range from –e to e — for example, the charge of the top quarks is 2/3 e. You may be interested in the interaction between the electron and the uranium nucleus, since the uranium nucleus charge is 92 e. Yes, in this case, the interaction is very strong! But in this case only a part of the effects I described for strong interactions manifest, since a change in the charge of only one of the interacting objects (in particular, a heavy one) does not increase the probability of detecting virtual electron-positron pairs. This will change only if the charge of the electron itself becomes much larger than e! So even a uranium atom remains much simpler than a proton.
How strong are other known interactions of nature? We have seen that in electrical interactions, the force is equal to α — at least at the microscopic, atomic, and subatomic level. And at such distances, down to millionths of a meter, α is constant. It does not depend on r, and in particular, therefore, is such a convenient measure. But in fact, the force of interaction can vary with distance, which complicates things. For electromagnetism, this is not so important, this effect is very small. But for other forces it is important.
The so-called weak nuclear interaction, of course, is weak. It is weak at the macroscopic, atomic and even nuclear level. But his strength is not constant. At distances large compared to ℏ c / M W ~ 3 × 10 -18 meters (on the order of 1/300 of the proton radius), where M W = 80 GeV / c 2 is the mass of the particle W, its strength α is approximately equal to
Exhibitor makes this interaction surprisingly weak! Even at distances comparable to the size of a proton, this factor is already equal to e -300 , which means reducing its strength by so much that I can’t even write down this number - this is 1 with 130 zeros. (This is more googol, units with one hundred zeros). And then this force quickly decreases further. Why? The same effect that gives the particle W (the perturbation of the field W) mass, makes it impossible to perturb the field W at large distances, unlike the effect exerted by an electron or a proton on an electric field. Accordingly, the effect of the W field does not work over long distances.
But for even smaller distances
Please note that it is several times larger than the electromagnetic force! Weak interaction is not inherently weak at all - see fig. 2. Warning: I do not include here the subtleties associated with the interaction of weak and electromagnetic interactions at such short distances, as well as with a very slow change in the force, which becomes noticeable at much shorter distances.
A weak interaction looks so weak when it is observed using nuclear physics, atoms and everyday life as an example, a huge mass of particle W. If particle W had no mass, then the effect of a “weak” nuclear interaction would be stronger than that of an electric one! This is another context in which the Higgs field, which gives the mass of W to its mass, plays an important role in our lives!
A strong nuclear interaction that attracts and repels quarks and gluons (but not electrons) works quite differently. At distances that we discussed in the case of a weak nuclear interaction - 3 × 10 -18 meters - a strong nuclear interaction is much stronger than both weak and electromagnetic:
It is not particularly strong; this is about ten times weaker than a truly strong interaction, and only ten times stronger than electromagnetism. In fact, although at macroscopic distances they are very different, but strong nuclear, weak nuclear and electromagnetic interactions differ only about 10 times from each other at distances less than 3 × 10 -18 m. This is surprising, and probably not by chance. From there, to the idea of a “ grand unification ” of these three forces, the step is quite small - there is an opinion that at much shorter distances all three interactions have the same power, and become parts of a more universal interaction.
But at large distances the strong nuclear interaction gradually becomes relatively strong. And again, I remind you that we mean "weak" and "strong"; the interaction becomes weaker in absolute terms with increasing r, but compared to, say, an electromagnetic interaction at the same distance, it becomes stronger.
It is very strong! And by the time r reaches 10 -15 m, the radius of the proton, α strong becomes greater than 1 and can no longer be determined in a unique way.
In short, a strong nuclear interaction, which at distances much shorter than the radius of the proton, demonstrates moderate strength, grows (in relative sense) with increasing distances, and becomes really strong at a distance of 10-15 m (this is shown in Fig. 2). It is precisely this strong interaction that creates the proton and the neutron, and the residual effect of this interaction combines these objects into the nucleus of an atom. Other important effects from the enhancement of this interaction are the transformation of high-energy quarks and gluons into hadron jets .
Why does the strong interaction gradually increase with increasing r? I will tell you this some other time, but in fact, this is a very subtle effect, arising due to perturbations (virtual particles) in the fields of quarks and gluons, which are affected by strong interaction. The same effects affect the weak and electromagnetic interaction, but not so much, so I did not mention it before. For example, at a distance of 3 × 10 -18 m, electromagnetic α becomes closer to 1/128 than to its value for large distances, equal to 1/137.
Given the strength of strong nuclear interaction, why do we not encounter it in everyday life? This is due to the subtleties of how it packs quarks, gluons and antiquarks so closely into protons and neutrons, that we never observe them separately. All this is very different from how weak electromagnetic interaction allows electrons to easily escape from atoms, allowing such phenomena as static electricity (which includes lightning) and electric current (including wires).
What about gravity? For particles known to us, gravity is surprisingly weak. For two fixed particles of mass m, gravity will have the value
Where G N is the Newton gravitational constant. Compare this with the electric force, which has α = ke 2 / ℏ c. The roles of k and e of electric forces are played here by G N and m. I note that I use Newton's formula for gravity, but as long as α gravity is small compared to 1, the Einstein formula for the attraction of two objects will be essentially the same.
Now let us rewrite the formula in terms of the Planck mass M P = 10 19 GeV / s 2 , or on the order of the mass of 10 million million protons, or 20 thousand million million million electrons. It is about one-tenth the mass of a grain of salt.
So for two protons with a mass of 1 GeV / c 2, the gravitational interaction between them will be expressed by a square of 10 -19 :
This is a unit in front of which there are 37 zeros and a decimal separator! And for two electrons
Which, since the electron mass is about 2000 times smaller than the proton mass, 4 million times weaker. Even for a pair of upper quarks, which are almost 200 times heavier than a proton, and whose mass is greatest among the masses of all known particles, the gravitational force will be equal
This is approximately 100,000,000,000,000,000,000,000,000,000,000 times smaller than the electrical interaction of the two upper quarks. Therefore, in fig. 2 gravity is not displayed.
If you think this amazing weakness of gravity explains why you (using the electrical forces that feed your muscles and hold your body) can move so freely, despite the fact that you are attracted by the whole huge Earth. It even explains how the Earth can be an atom so many times larger; gravity wants to compress the Earth, but the integrity of the atoms, whose electrical forces resist compression, hinders this. If the gravitational forces were much stronger, or the electric ones were weaker, gravity would compress the Earth to a much smaller size and much greater density.
Gravity is so weak that it's amazing that we discovered it at all. Why did she become the first force known to people? Because it is the only force that survives at very long distances in ordinary matter.
• Weak nuclear interaction becomes extremely weak over long distances.
• Electromagnetism survives longer, and although this interaction is not very strong, it is enough to bind most electrons and atomic nuclei into electrically neutral combinations, whose electrically forces are mutually destroyed. For example, a hydrogen atom does not attract a distant electron, because an electron in a hydrogen atom pushes it away, and a proton in a nucleus attracts it, and these two forces are balanced.
• Strong nuclear interaction is so strong that it binds quarks, gluons and antiquarks in combination, which also exhibit similar balancing effects.
• But there is nothing to balance gravity. There are no particles that create a gravitational interaction that repels matter; therefore, it is impossible to combine two particles so that their gravitational effects on distant objects are balanced.
Since 2012, we have a new food for thought: the interaction of particles caused by the Higgs field. It should not be confused with the effect due to which the Higgs field gives all known particles their mass; The Higgs field can have this effect on a single, isolated particle. It is not an impact, it does not pull or push. But the Higgs field can also generate the interaction of two particles; This happens very much like electromagnetism. However, with ordinary matter, this effect is very, very difficult to detect. At short distances for particles such as electrons and the upper and lower quarks that dominate the proton, the Higgs interaction is very weak (weaker than electromagnetism, but much stronger than gravity). At large distances, like the weak nuclear interaction, the Higgs interaction becomes extremely weak,since the Higgs particle, like the W particle, has mass.
The Higgs field generates an interaction similar to a weak nuclear interaction in that it has a very small impact distance and that it becomes ineffective at distances large compared to ℏc / Mh ~ 2 × 10 -18 m (1/500 of the proton radius ), where M h ≈ 125 GeV / c 2 , is the mass of the Higgs particle. At first glance, the formula is similar to the formula for gravity, since the force of attraction is proportional to the masses of two elementary particles.
Where v = 246 GeV, this is the constant value of the Higgs field that exists in the entire Universe. (Actually, strictly speaking, there is another square root of 2 in the formula, but let's simplify it to improve understanding).
But be careful! Similarity to gravity can be confusing. This formula works exactly for well-known elementary particles - objects that get their mass from the Higgs field. It works for electrons, muons, and quarks. It does not work for protons, neutrons, atoms or you! Because the mass of the proton (and the neutron, and consequently, the atom, and consequently, yours) is not completely generated by the Higgs field. This is different from the formula for gravity, which is true for all slow objects! Instead, in the case of ordinary atomic matter, we would need to replace the formula with a similar, but having a different factor in front, its own for each atom. But qualitatively, the dependence on distance would remain similar.
In addition, the formula I wrote presupposes the existence of only one Higgs field and one Higgs particle (which has not yet been proved, but is the simplest possibility corresponding to the data obtained). If this is not the case, the formula will become more complicated, although it will retain a similar form.
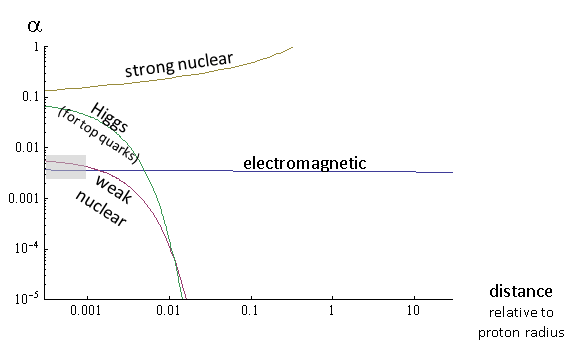
Fig. 2
How strong is this interaction? At very short distances, shorter than 2 × 10 -18 m, the Higgs interaction for two quarks is comparable to the strong nuclear interaction at the same distance (see Fig. 2)! But in the case of electrons with a smaller mass due to less interaction with the Higgs field, this interaction even at shorter distances will be much weaker than electrical - more than a thousand million times weaker - although thousands of millions of millions more times than the gravitational interaction of electrons . However, if we take two electrons in an atom that are located ten million times farther apart than 2 × 10 -18 m, then the Higgs interaction between them will be much, much less than even a tiny gravitational interaction, in e10,000,000 times And even if the Higgs field would be responsible for the entire mass of protons and neutrons, the Higgs interaction in the nucleus would still be much weaker than gravity, which, in turn, is incredibly small compared to the strong nuclear interaction that holds parts of the nucleus.
It is the surprising weakness of the Higgs interaction in the context of ordinary matter that makes it so difficult to detect. On the other hand, the Higgs interaction, like gravity, always works on gravity, and is not balanced. But on the third hand, this does not matter, since, like the weak nuclear interaction, the Higgs interaction does not survive over long distances, because the Higgs particle, like the W particle, has mass. The Higgs interaction at ultrashort distances is much stronger than gravity, but at nuclear and atomic distances it is much weaker because of the mass of the Higgs particle. And for small particles, of which we are composed, weakly interacting with the Higgs field, the Higgs interaction is always thousands of millions of times weaker than electrical forces, even very small distances.
So, although every atom of the Earth interacts through the Higgs with every other atom of the Earth, this force is so tiny, even for neighboring atoms, and especially for the distant ones, that its effect cannot be detected. Therefore, we had to directly find the Higgs particle to confirm the existence of the Higgs field; we could not search for the force created by him as we can observe the electric or magnetic forces and confirm in this way the existence of electric and magnetic fields.
When will we be able to observe the action of this force? Its effect will be detected for the first time either by scattering W and Z particles with each other (which will be done sooner or later, not directly, in collisions of protons in the Large Hadron Collider) or in interactions of the upper quark and the upper antiquark collider - by the way, I wrote my first work on particle physics about this phenomenon).
Specifically, I want to discuss what particle physics specialists have in mind when describing interactions as weak or strong. You can come across such terminology often, but if no one has explained it to you, it is impossible to guess what it means. So here is an explanation for you - albeit a long one, but I hope it will open your eyes to how nature works, and also raise many new questions that I hope to answer later.
"Weak" against "strong"
What do these terms mean? In ordinary life, we would imagine that a strong interaction can lift us into the air, and we can cope with the weak by tightening our muscles a little. But experts in particle physics do not mean that.
')
Speaking of the strong and the weak, physicists do not mean the absolute strength or weakness of the interaction. We are not talking about whether the interaction can break a window or hold a gold bar. In this context, the terms “strong” and “weak” are not entirely absolute, in the sense in which we use them in everyday life or even in elementary classes in physics. This terminology has emerged due to a deep understanding of quantum field theory, a modern mathematical language used to describe known elementary particles and forces. But it is fundamental to the modern discussion of these problems by physicists. So I will start with the rationale for the occurrence of such terms.
Take a pair of objects of a certain type, say, elementary particles, and place them at a distance r from each other. Suppose each has an effect F on the other. Then we say that the impact is weak if
Where h is Planck's constant, c is the speed of light. Often in physics it is convenient to use not h, but
In short, in particle physics:
• For weak interaction
• For strong interaction
Usually, even in theoretical studies, we do not encounter interactions much stronger . Such power makes them so complex that we work with them in a different way. But it is a long story.
It turns out that this characteristic is not talking about the absolute strength or weakness of the interaction, but about whether it is strong or weak compared to typical interactions operating at a distance r. It is not the interaction itself that is taken into account; interaction multiplied by the square of the distance is taken into account, and this value is compared with ℏ c.
To explain the usefulness of this concept, I will give an illustration for the case of electromagnetic interactions acting on simple charged particles - electrons, positrons and protons. The electric charge of electrons is –e; in protons and positrons, the charge is + e.
First, imagine two motionless protons, each of mass m and an electric charge + e, located at a distance r from each other. Electric force pushes them apart, and its value is given by the formula
The same formula is applicable for two electrons with a –e charge. For an electron and a positron, the interaction will be the same, only it will attract them and not push them apart.
What is k? This is the Coulomb constant , and its value depends on how to determine e, the basic unit of charge. But this is unimportant, since when discussing electrical interactions and elementary particles, we will always see the joint appearance of ke 2 . We don't need to know how big k is, we just need to know how big ke 2 is ?
It turns out that if r is greater than a millionth millionth of a meter, then ke 2 is approximately equal to 0.007, multiplied by (hc / 2π), where h is Planck's constant, and c is the speed of light. Therefore, we can write the electric force multiplied by r 2 as approximately equal to
Since 0.007 is much less than 1, electromagnetism is a weak interaction, and remains so at all distances measured by us.
It is very important not to get confused! Only from the fact that electromagnetism is a weak interaction, it does not follow that the interaction of two protons is weak in absolute terms. In fact, the electrical force trying to push two protons into the helium nucleus can be compared to the weight of a truck! And all this force acts on two tiny pieces! But for such small distances, this effect is rather weak, and a stronger interaction (“strong nuclear interaction”) resists electromagnetic repulsion, keeping protons and neutrons in the helium nucleus together.
By the way, for this value of 0.007 there is a historical name; it is called the fine structure constant (since it sets the size of small differences in the energies of different configurations of atoms), and is usually denoted α:
This is one of the most accurately measured quantities of nature. Often, people write it approximately equal to 1/137 (and for many years some scientists thought that the number 137 is some kind of special), but if you do it quite accurately, then you will have to write down 1 / 137.0359990 ...
So why does the fact that α is much less than 1 indicate that this interaction should be written down as weak and not as strong?
Why does α << 1 mean that the electromagnetic interaction is weak
The easiest way to show this is with an example in which force attracts particles — for example, an electron and a positron, or an electron and a proton. It is easier to start with an electron and a positron, since they have equal masses; they form a state similar to an atom called positronium , similar to the hydrogen atom formed by an electron and a proton, but more symmetrical, in which two particles orbit each other around each other. In a hydrogen atom, an electron moves in an orbit around a practically stationary proton. In fact, the formulas for hydrogen are also suitable for positronium, with small changes (differ by a factor of 2) in several places. (Yes, an electron and positron in positronium eventually annihilate and turn into two or three photons, but only after the particles make many billions of revolutions - which, however, takes a fraction of a second). For positronium in the lowest energy state:
• The typical speed of each particle is α / 2 × c;
• typical energy of motion (kinetic) of each particle is mc 2 × α 2/8;
the interaction energy (potential) of two particles is –mc 2 × α 2/2;
• the binding energy B of the positronium (the sum of the energy of motion and the energy of interaction) is equal to mc 2 × α 2/4;
• energy of the mass of the positronium 2 mc 2 - B; and since the second is much smaller than the first, the mass of the atom is only slightly less than the sum of the masses of the electron and positron.
In short, due to the fact that α is much less than 1, there are three important, interconnected facts:
• Electron and positron move at speeds comparable to the speed of light c.
• The kinetic energy, the potential energy and the binding energy B are small compared to the mass energy of the electron and positron, E = mc 2 .
• The mass of positronium is very close to the sum of the masses of the electron and positron.
All these statements are true regardless of how large or small the electron mass is; they depend only on a small value of α.
All this together means that for describing this atom-like state, Einstein’s special theory of relativity is not important. Newton's laws of motion are well suited for predictions, down to details not larger than α — that is, with an accuracy of 1% or better. And, as we will see later, this means that the system is relatively simple. It can be described using quantum mechanics with a fairly simple mathematics, without the participation of quantum field theory, which would have been necessary if SRT was important. The mathematics of the hydrogen atom is the same as that of the positronium, and it is so simple that physicists get to know it at the institute, in the first lessons on quantum mechanics.
This can be thought of in another useful, albeit less well-known way. It must be remembered that electrons, like all elementary particles, are in reality quanta — tiny perturbations of quantum fields. They are more like waves than small balls. Accordingly, they vibrate, like all waves: they have a frequency of vibrations. The time that passes from one vibration to another — which I like to call poetically “heartbeat” —is equal to hc / m. If α is small, then the time required for the light to cross the atom-like state is much more than 1 / α times than the heartbeat of the particles it contains. In this sense, positronium is quite large. And since the particles themselves move much slower than light, the particles take even more time to intersect this atom-like state — something in the region of 1 / α 2 heartbeats.
When α is small, other things that could be more complex are also simplified. For example, the effect of a positron on an electron can cause an electron to turn into a virtual electron and a virtual photon - sometimes not for long. (Virtual “particles” are not particles; a real particle is a well-behaved quantum field wave, and a virtual one is a more general perturbation of these fields). But this rarely happens when α is small. More rarely, the virtual photon itself is perturbed and turns into a virtual electron and positron. Since the energy of 2mc 2 needed to obtain a real electron and positron is nowhere to take (remember that the energies of motion and interactions are much smaller), the virtual electron and positron appear very rarely. The fact that virtual particles appear rarely allows us to say that “the positronium atom consists of an electron and a positron” —that is, most of the time. Only in very precise calculations is it necessary to be more careful and remember that this is not always the case. The same works for the hydrogen atom: it is (almost all the time) only one electron and one proton, held by simple electrical interaction.
What would happen if α were about equal to 1?
Now imagine that α gradually grows and approaches 1. What happens to the positronium?
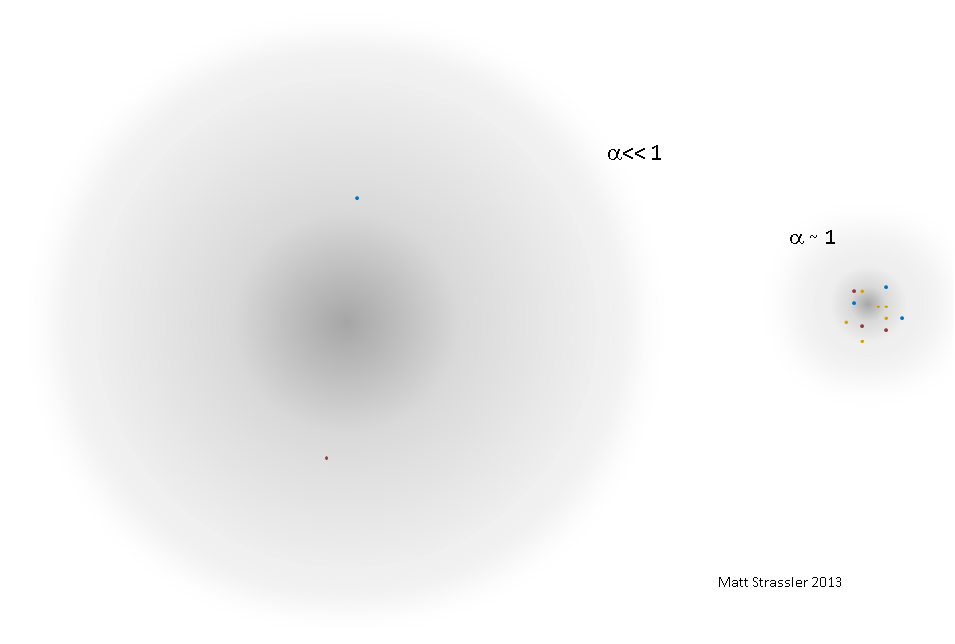
Fig. one
With increasing α, the interaction (at any distance) between the electron and the positron becomes stronger, and since they attract more strongly, the particles in an atom-like state move closer. Particles move faster, approaching the speed of light. The energy of motion of the particles increases, the value of the interaction energy increases, as the binding energy grows - and approaches 2m. Accordingly, the mass of the atom-like state is no longer equal to about 2m. The size of the atom-like state becomes smaller; the time required for its intersection by light, the time required for its intersection by particles, and the time elapsed between two heartbeats of particles begin to be compared with each other.
The increased interaction of the electron and positron leads to a more frequent appearance of virtual photons; the presence of a larger amount of energy in an atom facilitates the transformation of a virtual photon into a virtual electron and positron. When this happens, it becomes difficult to say which electron is real and which is virtual, since powerful forces also act between two electrons, as well as between an electron and any of the positrons. This can lead to the fact that the particle, being real, becomes virtual, and makes the virtual particle real - and vice versa. Meanwhile, virtual electrons and positrons can also emit or absorb photons, which can be both virtual and real.
The very separation between real and virtual particles becomes more difficult to hold. Real particles must be properly disturbed quantum field perturbations. But the atom-like state is so small that an electron and a positron take only one heartbeat to cross, and at that moment powerful interactions will force them to change direction. How can we show that such a particle is like a well-behaved indignation? A well-behaved wave must worry for a while — a few heartbeats — before external forces begin to influence it. And here our electron, although it is more like a real particle than a virtual one, is still strongly distorted, and no longer fits the definition of a “real particle”. And this electron may not exist for long. The appearance of a virtual electron-positron pair can be followed by the annihilation of a former real electron with a newly formed positron, after which a real / possibly virtual electron will remain.
So, instead of having a small α - a simple system with a mass slightly less than 2m, consisting of an electron and a positron moving at speeds much less than light - as α approaches 1, we find an extremely complex system in which many particles move with near-light speeds, with a mass that is very different from 2m (see Fig. 1). It is impossible to say how many particles are inside - will we only consider real ones? If so, how to distinguish precisely the almost real from the almost virtual? The number of real particles can constantly change.
This is what characterizes a really strong interaction; the objects it forms are much more complex than atoms. Scientists, in a sense, were lucky that the first objects encountered on the way to quantum field theory were atoms. They are weak interaction - electromagnetic force - and they were easy to understand with the help of simple mathematics of quantum mechanics, in which the number of particles is constant. Protons, on the other hand, retain a strong interaction - a strong nuclear interaction. Therefore, it is not surprising that the structure of protons is much, much more complicated than that of atoms. The number of particles inside a proton is constantly changing - and this requires a much more complex mathematics of quantum field theory.
By the way, the electrical interaction between two electrons is weak due to the fact that α is small. The same is true for interactions between two elementary particles, since the charges of all known particles are in the range from –e to e — for example, the charge of the top quarks is 2/3 e. You may be interested in the interaction between the electron and the uranium nucleus, since the uranium nucleus charge is 92 e. Yes, in this case, the interaction is very strong! But in this case only a part of the effects I described for strong interactions manifest, since a change in the charge of only one of the interacting objects (in particular, a heavy one) does not increase the probability of detecting virtual electron-positron pairs. This will change only if the charge of the electron itself becomes much larger than e! So even a uranium atom remains much simpler than a proton.
How weak is weak nuclear interaction? Complex issue…
How strong are other known interactions of nature? We have seen that in electrical interactions, the force is equal to α — at least at the microscopic, atomic, and subatomic level. And at such distances, down to millionths of a meter, α is constant. It does not depend on r, and in particular, therefore, is such a convenient measure. But in fact, the force of interaction can vary with distance, which complicates things. For electromagnetism, this is not so important, this effect is very small. But for other forces it is important.
The so-called weak nuclear interaction, of course, is weak. It is weak at the macroscopic, atomic and even nuclear level. But his strength is not constant. At distances large compared to ℏ c / M W ~ 3 × 10 -18 meters (on the order of 1/300 of the proton radius), where M W = 80 GeV / c 2 is the mass of the particle W, its strength α is approximately equal to
Exhibitor makes this interaction surprisingly weak! Even at distances comparable to the size of a proton, this factor is already equal to e -300 , which means reducing its strength by so much that I can’t even write down this number - this is 1 with 130 zeros. (This is more googol, units with one hundred zeros). And then this force quickly decreases further. Why? The same effect that gives the particle W (the perturbation of the field W) mass, makes it impossible to perturb the field W at large distances, unlike the effect exerted by an electron or a proton on an electric field. Accordingly, the effect of the W field does not work over long distances.
But for even smaller distances
Please note that it is several times larger than the electromagnetic force! Weak interaction is not inherently weak at all - see fig. 2. Warning: I do not include here the subtleties associated with the interaction of weak and electromagnetic interactions at such short distances, as well as with a very slow change in the force, which becomes noticeable at much shorter distances.
A weak interaction looks so weak when it is observed using nuclear physics, atoms and everyday life as an example, a huge mass of particle W. If particle W had no mass, then the effect of a “weak” nuclear interaction would be stronger than that of an electric one! This is another context in which the Higgs field, which gives the mass of W to its mass, plays an important role in our lives!
Strong nuclear interaction
A strong nuclear interaction that attracts and repels quarks and gluons (but not electrons) works quite differently. At distances that we discussed in the case of a weak nuclear interaction - 3 × 10 -18 meters - a strong nuclear interaction is much stronger than both weak and electromagnetic:
It is not particularly strong; this is about ten times weaker than a truly strong interaction, and only ten times stronger than electromagnetism. In fact, although at macroscopic distances they are very different, but strong nuclear, weak nuclear and electromagnetic interactions differ only about 10 times from each other at distances less than 3 × 10 -18 m. This is surprising, and probably not by chance. From there, to the idea of a “ grand unification ” of these three forces, the step is quite small - there is an opinion that at much shorter distances all three interactions have the same power, and become parts of a more universal interaction.
But at large distances the strong nuclear interaction gradually becomes relatively strong. And again, I remind you that we mean "weak" and "strong"; the interaction becomes weaker in absolute terms with increasing r, but compared to, say, an electromagnetic interaction at the same distance, it becomes stronger.
It is very strong! And by the time r reaches 10 -15 m, the radius of the proton, α strong becomes greater than 1 and can no longer be determined in a unique way.
In short, a strong nuclear interaction, which at distances much shorter than the radius of the proton, demonstrates moderate strength, grows (in relative sense) with increasing distances, and becomes really strong at a distance of 10-15 m (this is shown in Fig. 2). It is precisely this strong interaction that creates the proton and the neutron, and the residual effect of this interaction combines these objects into the nucleus of an atom. Other important effects from the enhancement of this interaction are the transformation of high-energy quarks and gluons into hadron jets .
Why does the strong interaction gradually increase with increasing r? I will tell you this some other time, but in fact, this is a very subtle effect, arising due to perturbations (virtual particles) in the fields of quarks and gluons, which are affected by strong interaction. The same effects affect the weak and electromagnetic interaction, but not so much, so I did not mention it before. For example, at a distance of 3 × 10 -18 m, electromagnetic α becomes closer to 1/128 than to its value for large distances, equal to 1/137.
Given the strength of strong nuclear interaction, why do we not encounter it in everyday life? This is due to the subtleties of how it packs quarks, gluons and antiquarks so closely into protons and neutrons, that we never observe them separately. All this is very different from how weak electromagnetic interaction allows electrons to easily escape from atoms, allowing such phenomena as static electricity (which includes lightning) and electric current (including wires).
Gravity
What about gravity? For particles known to us, gravity is surprisingly weak. For two fixed particles of mass m, gravity will have the value
Where G N is the Newton gravitational constant. Compare this with the electric force, which has α = ke 2 / ℏ c. The roles of k and e of electric forces are played here by G N and m. I note that I use Newton's formula for gravity, but as long as α gravity is small compared to 1, the Einstein formula for the attraction of two objects will be essentially the same.
Now let us rewrite the formula in terms of the Planck mass M P = 10 19 GeV / s 2 , or on the order of the mass of 10 million million protons, or 20 thousand million million million electrons. It is about one-tenth the mass of a grain of salt.
So for two protons with a mass of 1 GeV / c 2, the gravitational interaction between them will be expressed by a square of 10 -19 :
This is a unit in front of which there are 37 zeros and a decimal separator! And for two electrons
Which, since the electron mass is about 2000 times smaller than the proton mass, 4 million times weaker. Even for a pair of upper quarks, which are almost 200 times heavier than a proton, and whose mass is greatest among the masses of all known particles, the gravitational force will be equal
This is approximately 100,000,000,000,000,000,000,000,000,000,000 times smaller than the electrical interaction of the two upper quarks. Therefore, in fig. 2 gravity is not displayed.
If you think this amazing weakness of gravity explains why you (using the electrical forces that feed your muscles and hold your body) can move so freely, despite the fact that you are attracted by the whole huge Earth. It even explains how the Earth can be an atom so many times larger; gravity wants to compress the Earth, but the integrity of the atoms, whose electrical forces resist compression, hinders this. If the gravitational forces were much stronger, or the electric ones were weaker, gravity would compress the Earth to a much smaller size and much greater density.
Gravity is so weak that it's amazing that we discovered it at all. Why did she become the first force known to people? Because it is the only force that survives at very long distances in ordinary matter.
• Weak nuclear interaction becomes extremely weak over long distances.
• Electromagnetism survives longer, and although this interaction is not very strong, it is enough to bind most electrons and atomic nuclei into electrically neutral combinations, whose electrically forces are mutually destroyed. For example, a hydrogen atom does not attract a distant electron, because an electron in a hydrogen atom pushes it away, and a proton in a nucleus attracts it, and these two forces are balanced.
• Strong nuclear interaction is so strong that it binds quarks, gluons and antiquarks in combination, which also exhibit similar balancing effects.
• But there is nothing to balance gravity. There are no particles that create a gravitational interaction that repels matter; therefore, it is impossible to combine two particles so that their gravitational effects on distant objects are balanced.
Higgs interaction?
Since 2012, we have a new food for thought: the interaction of particles caused by the Higgs field. It should not be confused with the effect due to which the Higgs field gives all known particles their mass; The Higgs field can have this effect on a single, isolated particle. It is not an impact, it does not pull or push. But the Higgs field can also generate the interaction of two particles; This happens very much like electromagnetism. However, with ordinary matter, this effect is very, very difficult to detect. At short distances for particles such as electrons and the upper and lower quarks that dominate the proton, the Higgs interaction is very weak (weaker than electromagnetism, but much stronger than gravity). At large distances, like the weak nuclear interaction, the Higgs interaction becomes extremely weak,since the Higgs particle, like the W particle, has mass.
The Higgs field generates an interaction similar to a weak nuclear interaction in that it has a very small impact distance and that it becomes ineffective at distances large compared to ℏc / Mh ~ 2 × 10 -18 m (1/500 of the proton radius ), where M h ≈ 125 GeV / c 2 , is the mass of the Higgs particle. At first glance, the formula is similar to the formula for gravity, since the force of attraction is proportional to the masses of two elementary particles.
Where v = 246 GeV, this is the constant value of the Higgs field that exists in the entire Universe. (Actually, strictly speaking, there is another square root of 2 in the formula, but let's simplify it to improve understanding).
But be careful! Similarity to gravity can be confusing. This formula works exactly for well-known elementary particles - objects that get their mass from the Higgs field. It works for electrons, muons, and quarks. It does not work for protons, neutrons, atoms or you! Because the mass of the proton (and the neutron, and consequently, the atom, and consequently, yours) is not completely generated by the Higgs field. This is different from the formula for gravity, which is true for all slow objects! Instead, in the case of ordinary atomic matter, we would need to replace the formula with a similar, but having a different factor in front, its own for each atom. But qualitatively, the dependence on distance would remain similar.
In addition, the formula I wrote presupposes the existence of only one Higgs field and one Higgs particle (which has not yet been proved, but is the simplest possibility corresponding to the data obtained). If this is not the case, the formula will become more complicated, although it will retain a similar form.

Fig. 2
How strong is this interaction? At very short distances, shorter than 2 × 10 -18 m, the Higgs interaction for two quarks is comparable to the strong nuclear interaction at the same distance (see Fig. 2)! But in the case of electrons with a smaller mass due to less interaction with the Higgs field, this interaction even at shorter distances will be much weaker than electrical - more than a thousand million times weaker - although thousands of millions of millions more times than the gravitational interaction of electrons . However, if we take two electrons in an atom that are located ten million times farther apart than 2 × 10 -18 m, then the Higgs interaction between them will be much, much less than even a tiny gravitational interaction, in e10,000,000 times And even if the Higgs field would be responsible for the entire mass of protons and neutrons, the Higgs interaction in the nucleus would still be much weaker than gravity, which, in turn, is incredibly small compared to the strong nuclear interaction that holds parts of the nucleus.
It is the surprising weakness of the Higgs interaction in the context of ordinary matter that makes it so difficult to detect. On the other hand, the Higgs interaction, like gravity, always works on gravity, and is not balanced. But on the third hand, this does not matter, since, like the weak nuclear interaction, the Higgs interaction does not survive over long distances, because the Higgs particle, like the W particle, has mass. The Higgs interaction at ultrashort distances is much stronger than gravity, but at nuclear and atomic distances it is much weaker because of the mass of the Higgs particle. And for small particles, of which we are composed, weakly interacting with the Higgs field, the Higgs interaction is always thousands of millions of times weaker than electrical forces, even very small distances.
So, although every atom of the Earth interacts through the Higgs with every other atom of the Earth, this force is so tiny, even for neighboring atoms, and especially for the distant ones, that its effect cannot be detected. Therefore, we had to directly find the Higgs particle to confirm the existence of the Higgs field; we could not search for the force created by him as we can observe the electric or magnetic forces and confirm in this way the existence of electric and magnetic fields.
When will we be able to observe the action of this force? Its effect will be detected for the first time either by scattering W and Z particles with each other (which will be done sooner or later, not directly, in collisions of protons in the Large Hadron Collider) or in interactions of the upper quark and the upper antiquark collider - by the way, I wrote my first work on particle physics about this phenomenon).
Source: https://habr.com/ru/post/373921/
All Articles