Flying bucket

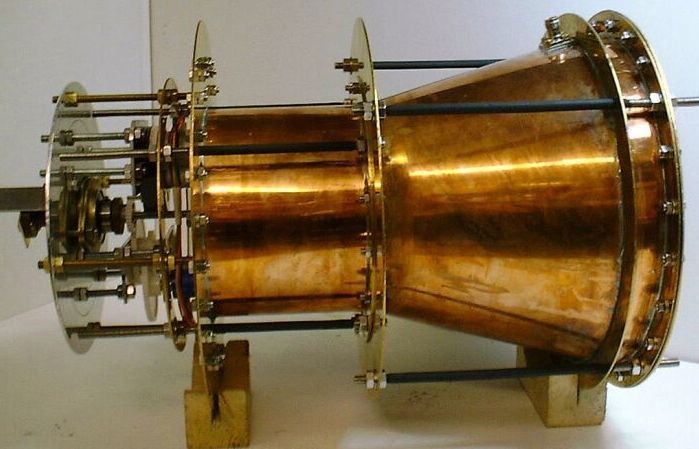
Just yesterday, my friend had a question: how long does it take to reach the first cosmic velocity on EmDrive? Let's count this case together.

Block theory

A couple of reservations: we will fly in space (otherwise we will not even be able to rise above the Earth) on that same EmDrive from the acclaimed NASA report . Apply only school physics.

We are looking for the time to reach a certain speed setpoint. $$$v$. According to the formula of uniformly accelerated motion $$$v = a⋅t$(initial speed is zero). Express time: $$$t = v / a$.
According to the second law of Newton $$$a = f / m$.
We know EmDrive specific traction: $$$u = 1,2 \,$mN / kW To find the strength $$$F$we need power $$$P$then get $$$F = u⋅P$.
Total:

$$

$$t = \ frac {v} {a} = \ frac {v⋅m} {F} = \ frac {v⋅m} {u⋅P}$$

We collect numbers

We need: target speed ( $$$v$), load mass ( $$$m$) and the power of the generator that will feed our EmDrive ( $$$P$).
')
The first cosmic speed is 7.9 km / s, everything is simple.

We will accelerate 3 tons of cargo (“Proton” brings up to 3.7 tons into geostationary orbit). A lot of small EmDrive write down there, so that no longer distracted by it.

It remains to power our "bucket". We will not consider the diesel generator, for calculations (taking into account the varying mass of fuel) will go beyond the school knowledge, and it is much more profitable to make a classic rocket than to power the EmDrive from a fuel generator.

The most adequate solution seems to be the energy of the sun. The maximum theoretical power of solar panels is 0.3 kW / kg. Let two of our three tons be solar panels. Total power - 600 kW.

Substitute all our values:
$$$ inline $ t = \ frac {7.9⋅10 ^ 3⋅3000} {1.2⋅10 ^ {- 3} 0.3⋅2000} $ inline $ ≈ 32,916,667 c = 380 days 23 h 31 min 7 s

For comparison, I counted another time to achieve the 2nd and 3rd space, and also tried to connect an "endless" AA battery and a Tesla Powerpack to our EmDrive:

Speed	AA battery	Tesla Powerpack	Solar panels
1 space (7.9 km / s)	869,222 years	12.5 years	381 days
2 space (11.16 km / s)	1,227,913 years old	17.7 years	1.47 years
3 space (16.65 km / s)	1,831,968 years	26.4 years	2.2 years

To mars?

But this crazy engineering genius did not want to stop and proposed a new task: how much to fly from Earth to Mars on the “solar bucket”? No sooner said than done.

We use the formula for the distance with uniformly accelerated motion: $$$s = \ frac {a⋅t ^ 2} {2}$( $$$s_0 = 0 \, \ text {and} v_0 = 0$). Express time: $$$t = \ sqrt {\ frac {2⋅s} {a}}$.
From the formulas above $$$a = \ frac {F} {m} = \ frac {u⋅P} {m}$.
Total:

$$$$ display $$ t = \ sqrt {\ frac {2⋅s} {a}} = \ sqrt {\ frac {2⋅s⋅m} {u⋅P}} $$ display $$

We will deliver, according to the precepts of Ilona Mask , 450 tons of cargo. From them we will give 150 tons under solar batteries. Take the distance from Earth to Mars about 300 million kilometers. In fact, the calculations of the flight are not as simple as it seems, due to the movement of the planets around the Sun , but for our rough approximation, it will do. We will accelerate the first half of the journey, the second half will slow down (although it is possible to brake on the planet, it is up to you).

Substitute the numbers:$$ $ inline $ t = 2⋅ \ sqrt {\ frac {300⋅10 ^ 9⋅450⋅10 ^ 3} {1.2⋅10 ^ {- 3} ⋅45000}} = 100,000,000 $ inline $ with ≈ 3.17 years

At the same time, he calculated how much flying with such a burden to the nearest star and to TRAPPIST-1 and brought to the table:

Where are we flying?	Time
Mars	3.17 years
Proxima Centauri	1157 years
TRAPPIST-1	3514 years old

Total

Despite the fact that we used the “small” version of EmDrive, it showed an unexpectedly good result. Perhaps the "big" EmDrive will be even cooler. If, of course, it works at all.

Use the scientific approach more often and do not be afraid to pick up a calculator.

PS By the way, the Cannae company promises its EmDrive weighing 3500 kg, which is able to deliver a cargo weighing 2000 kg at a distance of 0.1 light years for 15 years.

Useful links:

1. An article on GT about EmDrive .
2. The original NASA report about EmDrive in the Journal of Propulsion and Power .
3. Uniformly accelerated motion .
4. The second law of Newton .
5. Cosmic speed
6. An article about the use of solar panels in space .
7. Characteristics of various batteries .
8. Features Tesla Powerpack .