Portrait photography: bokeh, focal length, aperture and distance
There is an update at the bottom
Greetings to you, photoshoot! Portrait photography, in an amicable way, is an art. And art is a subjective thing.
But for photographing we use technical devices, and therefore we are inevitably “constrained” by the laws that this technique obeys. And I would like to talk about them, because there are lots of myths here ...
')

Who wants to immediately read something new about blur, can immediately flip through much lower
For a start, I want to remember that we are often limited by some “genre conventions”. There are certain “classic” frame layouts. For example, a portrait “across the chest”, where a region of space approximately 75 cm diagonally fits in the frame. Or a portrait of "head and shoulders," where diagonally turns out about 50 cm. These two options are the most common "templates" of photographs. In the future, we will talk about frames with 50 and 75 cm diagonally.
Now a little geometry ... Strong perspective distortions of the face are considered aesthetically unattractive, "disfiguring". Because of this, shooting portraits from a distance of less than 1 m is considered incorrect. Of course, what is considered an ugliness, and that “the artist’s vision” is a moot point, but still many special portrait lenses simply cannot be focused much closer to this distance. The Canon EF 85mm f / 1.2L USM professional portrait lens, for example, focuses no closer than 95 cm.
But the complete absence of perspective distortion is also considered ... Distortion! They say "the face looks too flat", because with a naked eye from a distance the face is already poorly visible, so we are not accustomed to seeing a large face completely "without perspective." Because of this, shooting portraits from a distance of more than 3 meters is also not recommended.
It is considered optimal to shoot people from a distance of approximately 1.5-2.0 m.
What focal length should the lens have in order to capture 75 cm diagonally from a distance of 1.5-2.0 m? If we have a classic film frame 36x24 mm or “full frame” matrix, then 80-100 mm.
What is the focal length needed to capture 50 cm diagonally from a distance of 1.5-2.0 m? 110-145 mm.
And what are our classic focal lengths for portrait lenses? 85 and 135 mm ... I think it should now be quite obvious why these numbers were chosen. It’s just that these focal lengths ensure the shooting of “sample” frame composition from the optimal distance.
Often people misunderstand the meaning of the phrase that “these lenses provide minimal distortion of the face”, considering that the focal length itself affects the distortion. But no, this is a mistake, the distortions are determined by the distance. Of course, the lenses themselves make distortion, distortion. But the opinion that it is minimal for just 85 and 135 mm lenses is absolutely not true. For example, the Canon EF 35mm f / 1.4L II USM wide-angle lens has a barrel-shaped (it makes the face “puffier”) with a distortion of 0.1%. Because he is not suitable for portraits? .. No, the above-mentioned portrait 85-mm Canon also has a barrel, and even 0.2%!
So choosing exactly the focal length of the lens, you need to look at what angle of view it has on the matrix of your camera. On the Nikon 1, with its scanty matrix, a 35 mm lens will be an excellent “shad”, which, if properly designed, will not create any distortion of your face. All conversations “the cropping factor doesn’t matter, the lens has been and is wide-angle” - it’s just an indicator of people’s lack of understanding of optics and geometry.
Look right up here
Now let's move on to another interesting aspect of portraits shooting - to bokeh ...

There are a lot of holivars on “Wow! What a gorgeous blur the background! This is great! ”VS“ Only stupid beginners shoot at the open diaphragm, the professional chooses a beautiful background. ” Let's not climb into them, just take for granted that there is a significant layer of people who want to see the background in the “blurred in the trash” portraits, and we will try to help them choose the right lens.
It is well known that the smaller the aperture number, the more blurred the background will be. f / 1.4 is better than f / 2.0. And f / 1.2 is great.
It is also known that the greater the focal length (but do not forget about what was said in the first part of the article!) - the more blurred the background.
But what will blur the background more, 50 mm f / 1.4 or 85 mm f / 2.0 ?! And if they are also on cameras with different matrix sizes? ..
Actually, the whole article got out of this question. Taking about ten different lenses and “driving them away” through several cameras with different matrices, taking different portraits in different conditions is an interesting experiment, but it is long and expensive. So I decided to do mathematical modeling ...
The “sphericity” of the “horse in vacuum” in my case was that I considered the lens as a single lens, which for focusing moves back and forth. I simply had no other options, because I wanted to find out how the focal length and aperture in general, and not a specific lens, affect the blurring of the background. And the same focal length with aperture can be for lenses of completely different designs ... So the blurring of the background (and even the distance at which it will give the desired diagonal of the frame!) For a particular real lens can be somewhat different. So, for example, in the photo above you can clearly see that a point source of light out of focus can be depicted in a photo not even around, but as an oval ... But, nevertheless, in most cases the differences between my calculation and the results of a real lens will be insignificant.
What did I actually do? Using sheet A4 on both sides with the transformations of the main formula of a thin lens, I got a calculation of the distance (from the matrix / film plane, the distance on the focusing scale of the lenses is measured from it) to place an object to get the desired diagonal of the frame at a given focal length and the dimensions of the matrix, as well as the circle of what size, the point source of light will be washed away, located at a given distance behind the subject (I considered the distance exactly from the subject, because the photographer with m leaves / comes closer, and does not ask to approach / depart portrayed). I expressed this size as a percentage of the diagonal of the frame so that you can directly compare it for cameras with different matrices.
Next was created this exel file . Orange cells are what we set (diagonal of the frame, dimensions of the matrix, distance from the object to the background), yellow ones are the main results of calculations (distance to evaluate whether this focal is suitable for such a portrait, and background blur), gray ones intermediate results of calculations (for example, “defocusing of the background” is the distance from the plane in which the background was focused to the plane of the matrix).
I sincerely believed that the results of the calculation would be simple conclusions like “50 mm f / 1.4 blurs the background worse than 85 mm f / 2.0”. But no!
If you shoot the portrait “across the chest” (75 cm) by placing both lenses on a camera with a 23.5x15.6 mm matrix (the most common APS-C format), then 50 mm f / 1.4 will blur 2.76% and 85 mm f / 2.0 - 2.56%, i.e. 50 mm "won." But at the same time, 50 mm f / 1.4 will blur 4.30%, and 85 mm f / 2.0 - 4.91%, i.e. "Ahead" 85 mm!
How so? .. It is very simple: the first pair of numbers is given for the case when the background is just a couple of meters behind the person’s back (which usually happens indoors), and the second when the background is already 20 m (which happens regularly in nature).
Those. When choosing which lens to take, in order to blur the background as much as possible, you need to be guided not only by their focal lengths and aperture sizes, but also by the distance from the portrait to the background .
And how exactly to be guided - download the file and “play”. I will cite here only a few heavily "truncated" tables.
Format:
focal length (focusing distance): aperture number (1.2, 1.4, etc.) - blurring (percentage)
Full frame (36x24 mm), 50 cm diagonal, up to a background of 2 m
85 mm (1.16 m): 1.2 - 8.75; 1.4 - 7.50; 1.8 - 5.83
135 mm (1.84 m): 2.0 - 6.99; 2.8 - 4.99; 3.5 - 3.99
Full frame (36x24 mm), 50 cm diagonal, up to a background of 20 m
85 mm (1.16 m): 1.2 - 12.43; 1.4 - 10.65; 1.8 - 8.29
135 mm (1.84 m): 2.0 - 11.53; 2.8 - 8.23; 3.5 - 6.59
Full frame (36x24 mm), 75 cm diagonal, up to a background of 2 m
85 mm (1.65 m): 1.2 - 5.14; 1.4 - 4.41; 1.8 - 3.43
135 mm (2.62 m): 2.0 - 3.92; 2.8 - 2.80; 3.5 - 2.24
Full frame (36x24 mm), 75 cm diagonal, to a background of 20 m
85 mm (1.65 m): 1.2 - 8.32; 1.4 - 7.13; 1.8 - 5.54
135 mm (2.62 m): 2.0 - 7.62; 2.8 - 5.44; 3.5 - 4.35
APS-C matrix (22.3x14.9 mm, cropping 1.61), 50 cm diagonal, up to a background of 2 m
50 mm (1.03 m): 1.2 - 5.40; 1.4 - 4.63; 1.8 - 3.60
85 mm (1.76 m): 1.2 - 7.51; 1.4 - 6.43; 1.8 - 5.00
APS-C matrix (22.3x14.9 mm, cropping 1.61), 50 cm diagonal, 20 m background
50 mm (1.03 m): 1.2 - 7.56; 1.4 - 6.48; 1.8 - 5.04
85 mm (1.76 m): 1.2 - 12.46; 1.4 - 10.68; 1.8 - 8.31
APS-C matrix (22.3x14.9 mm, cropping 1.61), 75 cm diagonal, background 2 m
50 mm (1.50 m): 1.2 - 3.16; 1.4 - 2.71; 1.8 - 2.10
85 mm (2.55 m): 1.2 - 4.17; 1.4 - 3.57; 1.8 - 2.78
APS-C matrix (22.3x14.9 mm, cropping 1.61), 75 cm diagonal, 20 m background
50 mm (1.50 m): 1.2 - 5.01; 1.4 - 4.30; 1.8 - 3.24
85 mm (2.55 m): 1.2 - 8.15; 1.4 - 6.99; 1.8 - 5.43
I hope at least someone will help this a bit with the choice of a lens for portrait shooting :)
UPDATE:
I updated the exel file .
Now, for a number of lenses, he plots the dependence of the background blur on the distance to it. Here is this:
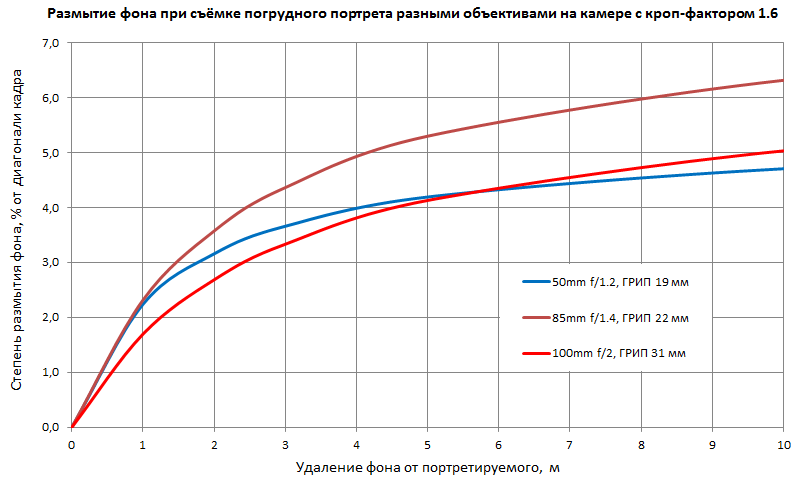
The graph clearly demonstrates the capabilities of different lenses at different distances from the background.
Well, a comparison of the theoretical model with practice ...
Canon EOS M5, matrix of 22.3x14.9 mm in size, Canon EF 50mm f / 1.8 STM lens, focusing distance - 1.5 m, LED at a distance of 3.5 m from the camera (2 m behind the focusing plane).
The image of the LED in the photo turned out to be approximately the size (it is not perfectly round and has not ideally clear edges, so there is an error of ± 2-3 pixels) 150 pixels.
The resolution of the matrix is 6000x4000 pixels, which gives 7211 conditional pixels on the diagonal of the frame.
150/7211 = 2.08%
The mathematical model predicted 2.10%. Within the limits of measurement error, the result of the experiment coincided with the predictions of the theory.
Greetings to you, photoshoot! Portrait photography, in an amicable way, is an art. And art is a subjective thing.
But for photographing we use technical devices, and therefore we are inevitably “constrained” by the laws that this technique obeys. And I would like to talk about them, because there are lots of myths here ...
')

Who wants to immediately read something new about blur, can immediately flip through much lower
For a start, I want to remember that we are often limited by some “genre conventions”. There are certain “classic” frame layouts. For example, a portrait “across the chest”, where a region of space approximately 75 cm diagonally fits in the frame. Or a portrait of "head and shoulders," where diagonally turns out about 50 cm. These two options are the most common "templates" of photographs. In the future, we will talk about frames with 50 and 75 cm diagonally.
Now a little geometry ... Strong perspective distortions of the face are considered aesthetically unattractive, "disfiguring". Because of this, shooting portraits from a distance of less than 1 m is considered incorrect. Of course, what is considered an ugliness, and that “the artist’s vision” is a moot point, but still many special portrait lenses simply cannot be focused much closer to this distance. The Canon EF 85mm f / 1.2L USM professional portrait lens, for example, focuses no closer than 95 cm.
But the complete absence of perspective distortion is also considered ... Distortion! They say "the face looks too flat", because with a naked eye from a distance the face is already poorly visible, so we are not accustomed to seeing a large face completely "without perspective." Because of this, shooting portraits from a distance of more than 3 meters is also not recommended.
It is considered optimal to shoot people from a distance of approximately 1.5-2.0 m.
What focal length should the lens have in order to capture 75 cm diagonally from a distance of 1.5-2.0 m? If we have a classic film frame 36x24 mm or “full frame” matrix, then 80-100 mm.
What is the focal length needed to capture 50 cm diagonally from a distance of 1.5-2.0 m? 110-145 mm.
And what are our classic focal lengths for portrait lenses? 85 and 135 mm ... I think it should now be quite obvious why these numbers were chosen. It’s just that these focal lengths ensure the shooting of “sample” frame composition from the optimal distance.
Often people misunderstand the meaning of the phrase that “these lenses provide minimal distortion of the face”, considering that the focal length itself affects the distortion. But no, this is a mistake, the distortions are determined by the distance. Of course, the lenses themselves make distortion, distortion. But the opinion that it is minimal for just 85 and 135 mm lenses is absolutely not true. For example, the Canon EF 35mm f / 1.4L II USM wide-angle lens has a barrel-shaped (it makes the face “puffier”) with a distortion of 0.1%. Because he is not suitable for portraits? .. No, the above-mentioned portrait 85-mm Canon also has a barrel, and even 0.2%!
So choosing exactly the focal length of the lens, you need to look at what angle of view it has on the matrix of your camera. On the Nikon 1, with its scanty matrix, a 35 mm lens will be an excellent “shad”, which, if properly designed, will not create any distortion of your face. All conversations “the cropping factor doesn’t matter, the lens has been and is wide-angle” - it’s just an indicator of people’s lack of understanding of optics and geometry.
Look right up here
Now let's move on to another interesting aspect of portraits shooting - to bokeh ...

There are a lot of holivars on “Wow! What a gorgeous blur the background! This is great! ”VS“ Only stupid beginners shoot at the open diaphragm, the professional chooses a beautiful background. ” Let's not climb into them, just take for granted that there is a significant layer of people who want to see the background in the “blurred in the trash” portraits, and we will try to help them choose the right lens.
It is well known that the smaller the aperture number, the more blurred the background will be. f / 1.4 is better than f / 2.0. And f / 1.2 is great.
It is also known that the greater the focal length (but do not forget about what was said in the first part of the article!) - the more blurred the background.
But what will blur the background more, 50 mm f / 1.4 or 85 mm f / 2.0 ?! And if they are also on cameras with different matrix sizes? ..
Actually, the whole article got out of this question. Taking about ten different lenses and “driving them away” through several cameras with different matrices, taking different portraits in different conditions is an interesting experiment, but it is long and expensive. So I decided to do mathematical modeling ...
The “sphericity” of the “horse in vacuum” in my case was that I considered the lens as a single lens, which for focusing moves back and forth. I simply had no other options, because I wanted to find out how the focal length and aperture in general, and not a specific lens, affect the blurring of the background. And the same focal length with aperture can be for lenses of completely different designs ... So the blurring of the background (and even the distance at which it will give the desired diagonal of the frame!) For a particular real lens can be somewhat different. So, for example, in the photo above you can clearly see that a point source of light out of focus can be depicted in a photo not even around, but as an oval ... But, nevertheless, in most cases the differences between my calculation and the results of a real lens will be insignificant.
What did I actually do? Using sheet A4 on both sides with the transformations of the main formula of a thin lens, I got a calculation of the distance (from the matrix / film plane, the distance on the focusing scale of the lenses is measured from it) to place an object to get the desired diagonal of the frame at a given focal length and the dimensions of the matrix, as well as the circle of what size, the point source of light will be washed away, located at a given distance behind the subject (I considered the distance exactly from the subject, because the photographer with m leaves / comes closer, and does not ask to approach / depart portrayed). I expressed this size as a percentage of the diagonal of the frame so that you can directly compare it for cameras with different matrices.
Next was created this exel file . Orange cells are what we set (diagonal of the frame, dimensions of the matrix, distance from the object to the background), yellow ones are the main results of calculations (distance to evaluate whether this focal is suitable for such a portrait, and background blur), gray ones intermediate results of calculations (for example, “defocusing of the background” is the distance from the plane in which the background was focused to the plane of the matrix).
I sincerely believed that the results of the calculation would be simple conclusions like “50 mm f / 1.4 blurs the background worse than 85 mm f / 2.0”. But no!
If you shoot the portrait “across the chest” (75 cm) by placing both lenses on a camera with a 23.5x15.6 mm matrix (the most common APS-C format), then 50 mm f / 1.4 will blur 2.76% and 85 mm f / 2.0 - 2.56%, i.e. 50 mm "won." But at the same time, 50 mm f / 1.4 will blur 4.30%, and 85 mm f / 2.0 - 4.91%, i.e. "Ahead" 85 mm!
How so? .. It is very simple: the first pair of numbers is given for the case when the background is just a couple of meters behind the person’s back (which usually happens indoors), and the second when the background is already 20 m (which happens regularly in nature).
Those. When choosing which lens to take, in order to blur the background as much as possible, you need to be guided not only by their focal lengths and aperture sizes, but also by the distance from the portrait to the background .
And how exactly to be guided - download the file and “play”. I will cite here only a few heavily "truncated" tables.
Format:
focal length (focusing distance): aperture number (1.2, 1.4, etc.) - blurring (percentage)
Full frame (36x24 mm), 50 cm diagonal, up to a background of 2 m
85 mm (1.16 m): 1.2 - 8.75; 1.4 - 7.50; 1.8 - 5.83
135 mm (1.84 m): 2.0 - 6.99; 2.8 - 4.99; 3.5 - 3.99
Full frame (36x24 mm), 50 cm diagonal, up to a background of 20 m
85 mm (1.16 m): 1.2 - 12.43; 1.4 - 10.65; 1.8 - 8.29
135 mm (1.84 m): 2.0 - 11.53; 2.8 - 8.23; 3.5 - 6.59
Full frame (36x24 mm), 75 cm diagonal, up to a background of 2 m
85 mm (1.65 m): 1.2 - 5.14; 1.4 - 4.41; 1.8 - 3.43
135 mm (2.62 m): 2.0 - 3.92; 2.8 - 2.80; 3.5 - 2.24
Full frame (36x24 mm), 75 cm diagonal, to a background of 20 m
85 mm (1.65 m): 1.2 - 8.32; 1.4 - 7.13; 1.8 - 5.54
135 mm (2.62 m): 2.0 - 7.62; 2.8 - 5.44; 3.5 - 4.35
APS-C matrix (22.3x14.9 mm, cropping 1.61), 50 cm diagonal, up to a background of 2 m
50 mm (1.03 m): 1.2 - 5.40; 1.4 - 4.63; 1.8 - 3.60
85 mm (1.76 m): 1.2 - 7.51; 1.4 - 6.43; 1.8 - 5.00
APS-C matrix (22.3x14.9 mm, cropping 1.61), 50 cm diagonal, 20 m background
50 mm (1.03 m): 1.2 - 7.56; 1.4 - 6.48; 1.8 - 5.04
85 mm (1.76 m): 1.2 - 12.46; 1.4 - 10.68; 1.8 - 8.31
APS-C matrix (22.3x14.9 mm, cropping 1.61), 75 cm diagonal, background 2 m
50 mm (1.50 m): 1.2 - 3.16; 1.4 - 2.71; 1.8 - 2.10
85 mm (2.55 m): 1.2 - 4.17; 1.4 - 3.57; 1.8 - 2.78
APS-C matrix (22.3x14.9 mm, cropping 1.61), 75 cm diagonal, 20 m background
50 mm (1.50 m): 1.2 - 5.01; 1.4 - 4.30; 1.8 - 3.24
85 mm (2.55 m): 1.2 - 8.15; 1.4 - 6.99; 1.8 - 5.43
I hope at least someone will help this a bit with the choice of a lens for portrait shooting :)
UPDATE:
I updated the exel file .
Now, for a number of lenses, he plots the dependence of the background blur on the distance to it. Here is this:

The graph clearly demonstrates the capabilities of different lenses at different distances from the background.
Well, a comparison of the theoretical model with practice ...
Canon EOS M5, matrix of 22.3x14.9 mm in size, Canon EF 50mm f / 1.8 STM lens, focusing distance - 1.5 m, LED at a distance of 3.5 m from the camera (2 m behind the focusing plane).
The image of the LED in the photo turned out to be approximately the size (it is not perfectly round and has not ideally clear edges, so there is an error of ± 2-3 pixels) 150 pixels.
The resolution of the matrix is 6000x4000 pixels, which gives 7211 conditional pixels on the diagonal of the frame.
150/7211 = 2.08%
The mathematical model predicted 2.10%. Within the limits of measurement error, the result of the experiment coincided with the predictions of the theory.
Source: https://habr.com/ru/post/369973/
All Articles