Interstellar and Theory of Relativity [SPOILERS]
After returning home after the premiere of Interstellar in high spirits, I looked at the GT. The fact that over the next day I saw in the comments to the post I was very sad. In this publication, I will examine a few "shoals" that the community saw in the film.
Anyone who cares why I believe that the commentators are wrong - I invite you to habrakat (giktymeskat?).
')
[SPOILER ALERT!]
So, here are the most common movie quibbles (I chose the ones I have the answer to):
Let's sort them out.
It's all very simple. We have seen that the black hole has an accretion disk. Its temperature decreases with distance from the center, therefore, whereas the inner parts emit in the X-ray, and maybe in the gamma range, the outer emit in the optics, which illuminates the planet.
Where did he come from? It may be the remains of a star, absorbed earlier. Maybe when it was a dual system.
In addition to the effect of relativistic time dilation associated with motion, there is the effect of gravitational time dilation. In this case, its action will be much more noticeable due to the powerful gravitational field. The closer to the center of the hole, the greater the modulus of the gravitational potential and the greater the deceleration. The ship was in a higher orbit, so it experienced a lesser slowdown in time.
The main argument of the haters and the most important is their delusion.
In fact, this is not always true. Supermassive black holes can “swallow” even stars without breaking them by tidal forces. Why is the tidal forces of heavy after. weak?
Yes, what really there, let's count.
The tidal force that acts on the volume element of mass m at distance h from the center of mass of the body in the field of a black hole of mass M at a distance R is equal to:
f = (2G * M / R ^ 3) * m * h. [3]
Take R - the Schwarzschild radius (the radius of the horizon of a non-rotating black hole). Suppose the Garganty mass is 15 * 10 ^ 12 solar masses (this is given in the Thorn's book [1]), or in kilograms M = 3 * 10 ^ 43 kg.
R = 2 * G * M / c ^ 2
Substitute.
f = c ^ 6 / (2GM) ^ 2 * (mh)
It can be seen that the tidal forces at the horizon of events are proportional to the square of the black hole mass, and with a body size of even kilometers, near the gravitational radius of Gargantua will be insignificant.
On the word we do not believe, let us estimate, taking only tens in the appropriate degrees.
f = 10 ^ 48 / (10 ^ -22 * 10 ^ 86) m * h = 10 ^ (- 16) m * h - this is very, very small.
I want to remind you that this is only a rough estimate. For accurate calculations, you need to take the Kerr metric.
Coop, from his point of view, falls remarkably into a black hole. You argue that it should hang on the edge of a black hole, but this is true only from the point of view of an infinitely distant observer . And all because the light is difficult to get out of the gravitational pit. We will really see an image of an object falling on a hole that will move more and more into the red region, then we will generally stop seeing it. What the observer sees is a certain “snapshot” of the object, taken just before reaching the event horizon. And while these photons, getting out of the gravitational pit, fly to us - the object has long gone below the horizon and moves to (or has reached) the singularity.
In the publication ([1] p.255) Thorn quotes Wheeler’s lecture (the collapse of a star into a black hole is described):
“Due to more and more rapid fall, the surface of the collapsing star runs away from the observer faster and faster. The light moves to the red area. With every millisecond, it becomes paler and paler and in less than a second it becomes too dark to be seen ... The star, like the Cheshire Cat, gradually disappears. The cat leaves behind a smile, and the star - only gravitational attraction. Gravitational attraction remains, but the light does not. The same with particles. "
For clarity, let us turn to a beautiful analogy from the same book [1].
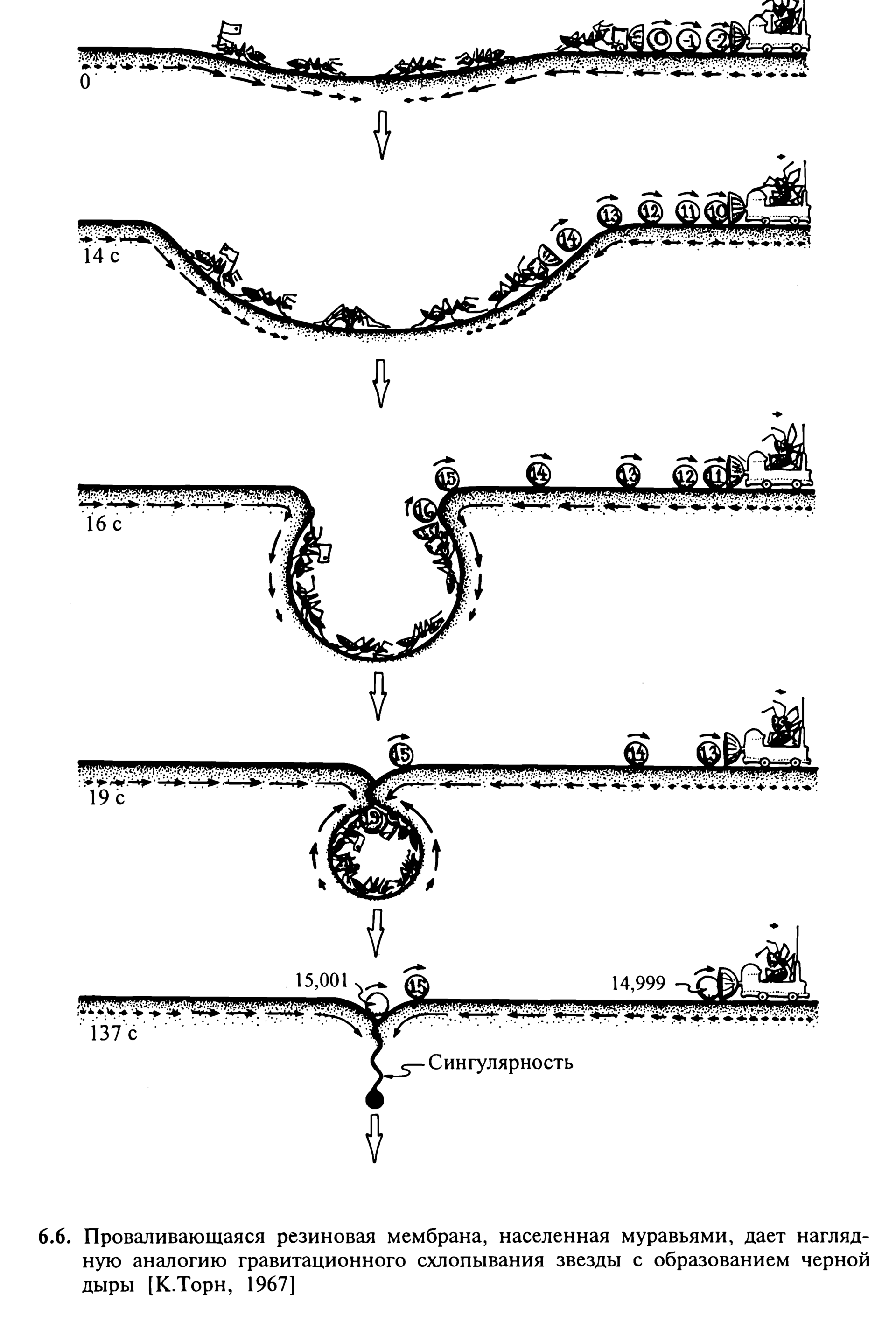
On the surface of the rubber membrane, the ants did not live themselves. The ants were pumped up very much, so they learned how to transfer information using balls with a constant speed (photons of local spill). Once, five ants gathered near the center of the membrane, and fell into the trap! The membrane began to sag so fast that they could not escape from there. Far from them was an ant astronomer. As soon as the membrane began to fall, the ants began to send signal balls, so that he could follow their fate. The collapse of the membrane leads to two effects. First, its surface is compressed, tightening objects to the center of the dip. Secondly, the membrane becomes curved, similar to the curved shape of the space around a collapsing star. As the collapse develops, the membrane shrinks faster and faster. Therefore, the astronomer gets ant signals at increasing intervals of time (reddening of the light). Ball number 15 was sent 15 seconds after the start of the crash, exactly at the moment of passing the critical circle. This ball remained there forever, because here the membrane shrank exactly with the speed of the balls. But in 0.001 seconds, the ants sent the ball 14,999. This ball reached an astronomer 122 seconds after the start of the disaster (the calculations that Thorn did here are unknown to me). The ball number 15.001 was tightened inside.
However, an astronomer ant will never know about a catastrophe, he will not get the ball 15 or more. And the fact that they started up to him, it will take so much time that the ant will seem that the collapse has slowed down and froze on the horizon of events.
What I would like to say. It is very nice to see that people are interested in such topics. But it is very unpleasant that people get into arguments and criticism, having a rather weak base under them. I hope I managed to convince you that you are mistaken, if not - read [1], but I cannot quote the whole book here.
I recommend reading to everyone [1]. I read it in my first year, caught my eye in the library. This popular science book, without formulas, is read very easily and naturally. The most important thing is that without knowing mathematics, after this book you will know and understand much more about black holes and other exotic objects. As a bonus - at the beginning of the book a physically authentic story about a journey to black holes, in which, by the way, that Interstellar is remotely visible.
You can also look at infographics here.
(thanks for the Lestat tip)
Still doubters can read this article with more accurate calculations, the Kerr metric and so on (thanks for the tip from Vladek ). Maybe I'll sip the translation. I take a timeout for a couple of days, I'm tired of GR, to be honest.
[1] C. Thorn “Black holes and folds of time. Bold legacy of Einstein. "
[2] Bisnovaty - Kogan "Relativistic astrophysics and physical cosmology."
[3] Thorn, Misner, Wheeler "Gravity."
Lord I will not and will not be able to answer all your angry comments and questions on the film. It took me enough time to search for confirmation of selected items from reliable sources. Of course, I could write it and so, empty words, but who would believe, eh? In addition, I have no desire to be besieged with books for a week to quench your thirst to show that you did not like the movie. The question is simple to ask. Finding a backed up answer is much more difficult.
Anyone who cares why I believe that the commentators are wrong - I invite you to habrakat (giktymeskat?).

')
[SPOILER ALERT!]
So, here are the most common movie quibbles (I chose the ones I have the answer to):
- Where does the light on the planet near the black hole come from?
- Why time on the water planet flew slower than on the ship?
- The ship at the horizon of events should break apart.
- The ship could not fall into a black hole, but had to hover near the horizon.
- How could he transmit messages by falling into a black hole?
Let's sort them out.
1. Light
It's all very simple. We have seen that the black hole has an accretion disk. Its temperature decreases with distance from the center, therefore, whereas the inner parts emit in the X-ray, and maybe in the gamma range, the outer emit in the optics, which illuminates the planet.
Where did he come from? It may be the remains of a star, absorbed earlier. Maybe when it was a dual system.
2. Time
In addition to the effect of relativistic time dilation associated with motion, there is the effect of gravitational time dilation. In this case, its action will be much more noticeable due to the powerful gravitational field. The closer to the center of the hole, the greater the modulus of the gravitational potential and the greater the deceleration. The ship was in a higher orbit, so it experienced a lesser slowdown in time.
3. Tidal forces
The main argument of the haters and the most important is their delusion.
In fact, this is not always true. Supermassive black holes can “swallow” even stars without breaking them by tidal forces. Why is the tidal forces of heavy after. weak?
Yes, what really there, let's count.
The tidal force that acts on the volume element of mass m at distance h from the center of mass of the body in the field of a black hole of mass M at a distance R is equal to:
f = (2G * M / R ^ 3) * m * h. [3]
Take R - the Schwarzschild radius (the radius of the horizon of a non-rotating black hole). Suppose the Garganty mass is 15 * 10 ^ 12 solar masses (this is given in the Thorn's book [1]), or in kilograms M = 3 * 10 ^ 43 kg.
R = 2 * G * M / c ^ 2
Substitute.
f = c ^ 6 / (2GM) ^ 2 * (mh)
It can be seen that the tidal forces at the horizon of events are proportional to the square of the black hole mass, and with a body size of even kilometers, near the gravitational radius of Gargantua will be insignificant.
On the word we do not believe, let us estimate, taking only tens in the appropriate degrees.
f = 10 ^ 48 / (10 ^ -22 * 10 ^ 86) m * h = 10 ^ (- 16) m * h - this is very, very small.
I want to remind you that this is only a rough estimate. For accurate calculations, you need to take the Kerr metric.
4. Falling to a black hole and signaling during a fall
Coop, from his point of view, falls remarkably into a black hole. You argue that it should hang on the edge of a black hole, but this is true only from the point of view of an infinitely distant observer . And all because the light is difficult to get out of the gravitational pit. We will really see an image of an object falling on a hole that will move more and more into the red region, then we will generally stop seeing it. What the observer sees is a certain “snapshot” of the object, taken just before reaching the event horizon. And while these photons, getting out of the gravitational pit, fly to us - the object has long gone below the horizon and moves to (or has reached) the singularity.
In the publication ([1] p.255) Thorn quotes Wheeler’s lecture (the collapse of a star into a black hole is described):
“Due to more and more rapid fall, the surface of the collapsing star runs away from the observer faster and faster. The light moves to the red area. With every millisecond, it becomes paler and paler and in less than a second it becomes too dark to be seen ... The star, like the Cheshire Cat, gradually disappears. The cat leaves behind a smile, and the star - only gravitational attraction. Gravitational attraction remains, but the light does not. The same with particles. "
For clarity, let us turn to a beautiful analogy from the same book [1].

On the surface of the rubber membrane, the ants did not live themselves. The ants were pumped up very much, so they learned how to transfer information using balls with a constant speed (photons of local spill). Once, five ants gathered near the center of the membrane, and fell into the trap! The membrane began to sag so fast that they could not escape from there. Far from them was an ant astronomer. As soon as the membrane began to fall, the ants began to send signal balls, so that he could follow their fate. The collapse of the membrane leads to two effects. First, its surface is compressed, tightening objects to the center of the dip. Secondly, the membrane becomes curved, similar to the curved shape of the space around a collapsing star. As the collapse develops, the membrane shrinks faster and faster. Therefore, the astronomer gets ant signals at increasing intervals of time (reddening of the light). Ball number 15 was sent 15 seconds after the start of the crash, exactly at the moment of passing the critical circle. This ball remained there forever, because here the membrane shrank exactly with the speed of the balls. But in 0.001 seconds, the ants sent the ball 14,999. This ball reached an astronomer 122 seconds after the start of the disaster (the calculations that Thorn did here are unknown to me). The ball number 15.001 was tightened inside.
However, an astronomer ant will never know about a catastrophe, he will not get the ball 15 or more. And the fact that they started up to him, it will take so much time that the ant will seem that the collapse has slowed down and froze on the horizon of events.
Conclusion
What I would like to say. It is very nice to see that people are interested in such topics. But it is very unpleasant that people get into arguments and criticism, having a rather weak base under them. I hope I managed to convince you that you are mistaken, if not - read [1], but I cannot quote the whole book here.
I recommend reading to everyone [1]. I read it in my first year, caught my eye in the library. This popular science book, without formulas, is read very easily and naturally. The most important thing is that without knowing mathematics, after this book you will know and understand much more about black holes and other exotic objects. As a bonus - at the beginning of the book a physically authentic story about a journey to black holes, in which, by the way, that Interstellar is remotely visible.
You can also look at infographics here.
(thanks for the Lestat tip)
Still doubters can read this article with more accurate calculations, the Kerr metric and so on (thanks for the tip from Vladek ). Maybe I'll sip the translation. I take a timeout for a couple of days, I'm tired of GR, to be honest.
Sources
[1] C. Thorn “Black holes and folds of time. Bold legacy of Einstein. "
[2] Bisnovaty - Kogan "Relativistic astrophysics and physical cosmology."
[3] Thorn, Misner, Wheeler "Gravity."
PS
Lord I will not and will not be able to answer all your angry comments and questions on the film. It took me enough time to search for confirmation of selected items from reliable sources. Of course, I could write it and so, empty words, but who would believe, eh? In addition, I have no desire to be besieged with books for a week to quench your thirst to show that you did not like the movie. The question is simple to ask. Finding a backed up answer is much more difficult.
Source: https://habr.com/ru/post/362677/
All Articles