Why did it take 100 years to find the exact value of the Planck constant?
Translation of Michael Trott's post " An Exact Value for the Planck Constant: Why Reaching It Took 100 Years ".
The code given in the article can be downloaded here .
I express my deep gratitude to Polina Sologub for assistance in translating and preparing the publication.
Content
- Some thoughts on the occasion of World Metrology Day in 2016
- Introduction and a little about me
- From the origins of the metric system to the present day.
- Increase the number of constants
- Existing SI system and kilogram problem
- New SI
- Second
- moth
- Kelvin
- Ampere
- candela
- Why are the basic units of measurement exactly 7?
- The way to change the definition of kilogram
The narration is conducted on behalf of Jean-Charles de Bord.
Some thoughts on the occasion of World Metrology Day in 2016
Let me introduce myself:
I am a man of science and I love accuracy.
All this time I was somewhere nearby.
I took the pound and the toaz from the people.
And I was next to Louis XVI
In moments of doubt and pain.
I'm damn sure that metric tape measure
Thanks to platinum standards will be established once and for all.
I am glad to meet you!
I hope you guessed my name?
')
Introduction and a little about me
If you have not guessed it yet, I am Jean-Charles de Borda : a sailor, a mathematician, a scientist and a member of the Academy of Sciences. I was born on May 4, 1733 in the city of Dax in France. Two weeks ago I celebrated my two hundred and eighty third birthday. Here I am:
In my hometown, a monument was erected in my honor. If you are nearby, linger to look at it. If you don’t know where Dax is, here’s a map:
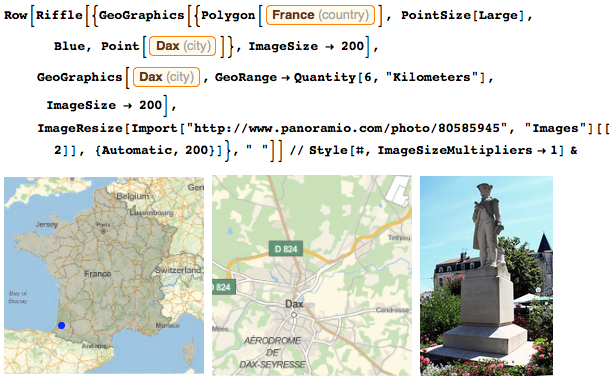
When I was a boy, France looked about the same as it is now. We had a little less territory on the east side, but in North America, my country owned a good piece of land:
I led an interesting life. By the age of 32, I spent a lot of time on the open sea doing research. When I was forty, I already commanded several ships in the battles of the Seven Years War (although I still spent most of my life in science).
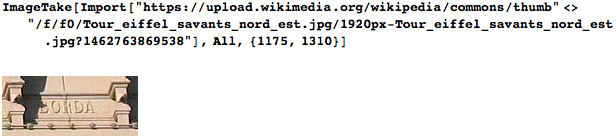
I died on February 19, 1799 in Paris, and no one knows where my grave is (I know, of course, but I cannot tell about it). My name is immortalized on the northeast side of the Eiffel Tower:
For centuries, many of my French colleagues, whose names joined mine, have told me that I deserve a place in the Pantheon. However, you will not find me either there or in the Pere Lachaise, Montparnasse or Montmartre cemeteries.
But this does not bother me. I am a modest person; The problem with the standard of the kilogram does not allow me to sleep at night. True, I will soon be able to rest for all the sleepless nights.
Let me tell you about it.
All my life I have been studying mathematics, geometry, physics and hydrology. I liked the measurements. You may have heard about the voting system (the Bord method) - this is also my invention . I also significantly improved the periodic circle (repeating circle) . This is where my story begins. The periodic circle played a decisive role in determining the exact size of the earth (for a discussion of my circle, see here ).
I lived in France during the time of the monarchy. This time was difficult for many people, and especially for peasants. This was partly because trade and commerce were in decline due to the lack of a unified system of units for the country as a whole. If you like reading on historical topics, I highly recommend you the book of Kula People and Measurements - you can imagine how things worked with measurements (first of all, weight and size) in France in 1790. A similar situation exists in other countries (see the report of Johann Georg Tralles on the situation in Switzerland ).
In August 1790, at the initiative of Louis XVI, I became the chairman of the Commission of Weights and Measures . And I still care about the fact that 1000 years after the initiative of Charlemagne to unify the measures and weights, a new work in this direction began. With the help of our commission, a metric system was created, which today is an international system of units, abbreviated to SI (in French, le Système international d'unités ).
The commission also included: Pierre-Simon Laplace (Laplace equations), Adrien-Marie Legendre ( Legendre polynomials), Joseph-Louis Lagrange ( Lagrange function), Antoine Lavoisier (formulation of the law of mass substance conservation), and Marquis de Condorcet (I always said Adrien Marie, that he should have ordered his portrait , but he denied it, saying that he was too busy with calculations). Over the past decade, Adrien-Marie became friends with Jacques-Louis David , who wrote a whole collection of paintings with him; unfortunately, none of the mortals will ever see them. Lagrange, Laplace, Monge , Condorcet and I were part of the original team (although at the very beginning with us was Jerome Lalande , and later Louis Lefebvre joined).
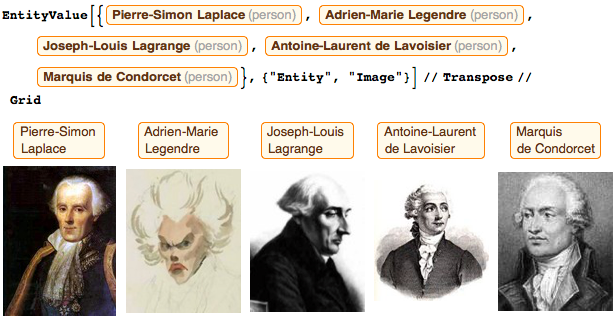
Three of us (Monge, Lagrange and Condorcet) were buried or immortalized in the Pantheon. I very much hope that one day they will be joined by Pierre-Simon, who deserves it.
As I have already said, it was difficult for French citizens to live in this era. Laplace wrote :
In use is a monstrous number of units; they are inconvenient for calculation, they are difficult to compare; the difficulties they produce in trade cannot be considered without recognizing that the adoption of a measuring system (in which its single parts are easily calculated and obtained at least arbitrarily, with units of measure to be indicated by nature itself) will become one of the most important services that any government can provide to society. A nation within which a new system of measures will appear will be the first to reap the benefits of its benefits, and then other nations will begin to follow its example, for which it will thus become a benefactor; the slow but inescapable dominion of the mind, which predominates over national jealousy, will crown all the obstacles overcome, which oppose advantages.
All five mathematicians (Monge, Lagrange, Laplace, Legendre and Condorcet) made a historically significant contribution to mathematics. Their names are called mathematical theorems, structures and operations:
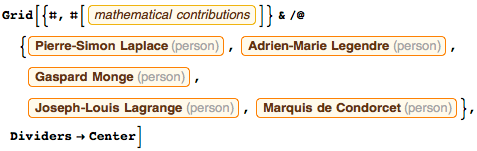
In 1979, Ruth Inés Champagne wrote a dissertation on the influence of five of my fellow citizens in creating a metric system (for more on Legendre’s contribution, see the article by Doris Hellman ). It seems to me that today mathematicians are mostly not interested in units of measurement anymore, and physics are the driving force in this area. But I did, as in Theodore P. Hill 's article on the method of combining probability distributions, which allows us to combine knowledge from various experiments (I’ll say that we have instant access to arXiv in the sky. I will say more: direct connection with arXiv was the biggest innovation of the past millennium ).
Our task was to create a unified unit of time, length, volume and mass. We needed such units of measure, with the help of which it would be possible to measure both tiny and astronomical objects. The principles of our approach were well summarized by John Quincy Adams , Secretary of State of the United States, in the 1821 book, Report on Weights and Measures .
Initially, we (we called ourselves metric men ) offered only a few prefixes: kilo-, deca-, hecto-, deci-, centi-, milli- and miri-, which is no longer used (in some old books you can find examples of the use of the prefix, meaning ten thousand).
This idea of ​​using prefixes appeared rather early in the process of developing new units of measurement. Here are our 1794 offers:
At one time, we also used demi- and double- (demi-hectoliter (= 50 liters) or double decaliter (= 20 liters)).
Time, length and mass, measured in physics, chemistry and astronomy, cover more than 50 orders of magnitude. And the units we created in the turbulent era of the French Revolution stood the test of time:
In the future, SI may need some more prefixes. In the newly opened LIGO observatory, the length of the interferometer arms was changed by approximately 10 yoktometers. Weight sensors with resolution in yoktogramma already exist. One yoktometr is 10 -24 meters. Humanity can already measure tiny forces of the order of Zeptonewtons.
On the other hand, astronomy needs prefixes greater than 10 24 . And one day they can become official.
I am a man of strict rules. It drives me crazy when in the twenty-first century I see people who do not follow the rules for using the SI prefixes. Recently, I saw someone write on a blackboard that a year consists of π decamegasseconds (π daMs):
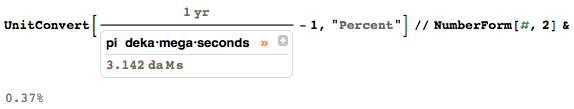
Let it even a good approximation (with a deviation of only 0.4%); only when this person learns that one should not combine prefixes?
Technological advances over the past two centuries have gone fast. And the prefixes mega-, giga-, tera- or nano-, pico- and femto- in the twenty-first century became common. Below you see the probability distribution of velocity values. Some speeds (for example, the speed of sound or the speed of light) are used more often than others, but in the distribution function you can find many local maxima:
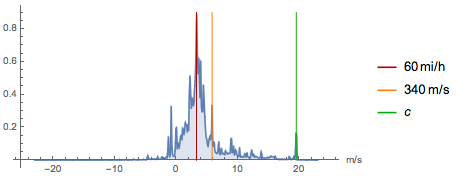
Here is the report (which we submitted in March 1791), which gave rise to the metric system and in which the conceptual value of meter and kilogram was described. It was signed by Lagrange, Laplace and Mongem (now it is available even through what the modern world calls “digital object identifier”, or DOI, like 10.3931 / e-ra-28950 ):
Most people think that base 10 is closely related to meter, second, and kilogram as units of measurement. However, we decided to use 10 as a basis for dividing units only on October 27, 1790. We seriously considered 12 as the basis, because the divisibility by 2, 3, 4 and 6 was convenient for trade. Today it is clear, however, that we made the right choice. Lagrange was right when he insisted that the number 10 should be the basis. During the French Revolution, we did not make any compromises. And on November 5, 1792, I proposed converting the clock into the decimal number system ( D'Alembert suggested this as early as 1754; for more details, see this article ). Humanity was not ready for such changes; perhaps in the twenty-first century decimal hours will finally be recognized as much more comfortable than 24 hours, 60 minutes, and 60 seconds. Our decimal hours were beautiful . For me it was a real surprise that humanity still divides the angle into 90 degrees. In my periodic circle, I divided the right angle by 100 degrees.
We wanted to make the new units truly equal for all people, and not to base them, for example, on the length of the king's forearm. In general, “for all times, for all nations” ( “À tous les temps, à tous les peuples ). Now this dream will come true.
I am sure that the day will come when Mendeleev predicted:
Let us facilitate the general distribution of the metric system, thereby contributing to the general well-being and the desired future rapprochement of peoples. It will not come immediately, but gradually and absolutely for sure.
will become a reality even in those 3 countries of the world that do not use this system:
SI units have been legally used in the United States since the mid-twentieth century, when conventional units of the United States began to be derived from the definitions of the basic SI units. Citizens can choose which units of measurement they want to use for trade.
We also put the decimal in the basis of the monetary system, and our franc was in circulation from 1793 to 2002. To date, all countries have money in circulation based on the decimal system. Here is a "breakdown" of coin values ​​by country:
We spoke very seriously about “all people”, as evidenced by our work with our sworn enemies - the UK and the new United States (through Thomas Jefferson personally) to create a new system of units for all major countries. But, as often happens, in the battle of politics and reason, politics won out.
I died on February 19, 1799, just a few months before the first successes of our group. On June 22, 1799, my dear friend Laplace made a speech about creating new units of length and mass even before new samples were delivered to the archives of the Republic (where they are still).
In case the reader became interested in my eventful life, Jean Mascart wrote in 1919 my biography, now available as a reprint .
From the origins of the metric system to today
Two of my friends, Jean-Baptiste-Joseph Delambre and Pierre MĂ©chain, went to France and Spain to take measurements to determine the meter as one ten-millionth distance from the North Pole to the Earth's equator. I am glad that the mission was approved. When Louis XVI approved the financing of the mission, he was already under arrest. My dear friend Lavoisier called his task "the most important mission that has ever been set before man ."
If you have not yet read the Ken Alder's Measure Book of All Things , I advise you to do so immediately. There is even a German film about the adventures of two of my old friends. Armed with a special tool that I designed for them, they did the work, the result of which was the creation of the meter. Despite the fact that we wanted to set a meter length equal to one ten-millionth half of the meridian passing from pole to the equator through Paris, I think that today this beautiful definition is entirely conceptual. We did not know at that time that the Earth was not completely round, and errors in the calculations of the flattening of the Earth resulted in a small, but regrettable error of 0.2 mm. Here is the length of half the Paris meridian, expressed in meters:
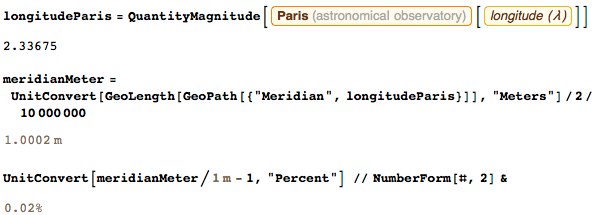
If all the elevations were taken into account (which Delambre and Mechene did not do, because for this they would have to travel along the entire meridian to take into account each mountain and hill!), And 3D-coordinates (including altitudes) were used, their meter in the end result would be shorter by 0.4 mm:
But the profile of the height of the Paris meridian:
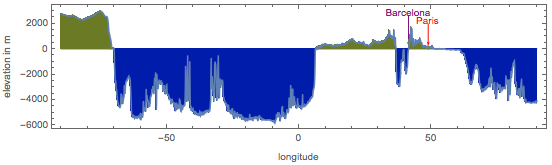
Due to the "fractality" of the Earth's surface, if we take the measurement tool even less, the error will increase significantly.
The meter will be another 0.9 mm longer if measured using a ruler several hundred meters long:
More realistic would be to follow the height of the sea level. The difference between the meter definitions obtained is only a few micrometers:
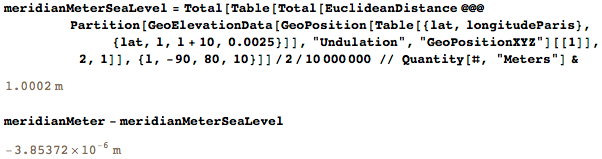
At a minimum, the meridian had to go through Paris (and not through London, as some British scientists of my time suggested). But in any case, finding the length of the meridian was just a stepping stone to creating a sample meter. After we received the sample, the meridian no longer interested us.
Here is a sketch of the triangulation carried out by Pierre and Jean-Baptiste during their adventurous six-year expedition. Thanks to the Internet and various French digitization projects, French readers interested in metrology and history can now read the results obtained online and reproduce our calculations:
Part of the Paris Meridian (and the Paris Observatory, marked in red) are marked with a marker: do not miss them during your next visit to Paris! Francois Arago peresimer Paris meridian. In 1804, Laplace received money from Napoleon to re-measure the meridian to test and improve our work:
Calculating the second, we repelled from the duration of the year. A kilogram as a unit of mass, we brought out of one liter of water. If any liquid is special, then it is, of course, water. Lavoisier and I argued a lot about the ideal temperature. There were two options: 0 ° and 4 ° . Initially, we thought about 0 ° C, because ice is easy to see. But then we chose 4 ° C, because it is at this temperature that water reaches its maximum density. A transition to 4 ° C was proposed by Louis Lefebvre-Jean . We defined the volume of a liter as a cube of one tenth of a meter. After comparison with high-precision measurements of distilled water, 1 kg turned out to be equal to 1.000028 dm 3 of water. An interested reader can find much more detailed information about the process of measuring water and creating the initial metric system. A shorter story in English can be found in a recent book by Williams .
I do not want to brag, but we also invented the name “meter” itself (derived from the Greek metron and the Latin metrum ), which we proposed on July 11, 1792 as the name for the new unit of length.
To observe historical accuracy, I want to note: until I entered the celestial realms, I always thought that our group was the first to accomplish this. I was amazed when, shortly after my arrival, I-Xing and Nanjing Yue told me about their expedition on the definition of the unit of measurement of length, which ran from 721 to 725, that is, more than 1,000 years before ours.
I am very pleased that we have defined the meter in this way. Initially, the idea was to determine the meter using a pendulum of the correct length with a half-period of 1 second. But I did not want any changes related to the second to affect the length of the meter. Since certain kinds of dependencies in the system of units are inevitable, they must be minimized.
At that time, the idea of ​​determining the meter based on the shape of the earth, and the second — on the motion of the earth around the sun, seemed good. It was even the best idea we could realize at the time. We did not know how the tides and time changed the shape of the Earth, or how the continents moved away from each other. But we believed in the future of mankind, believed that the accuracy of measurements would increase, although we did not know what exactly would change. These were our first steps taken to accurately measure distances in France. Today we have high-precision geo maps:
The best masters of my time melted platinum, and we forged meter-long bars and kilogram samples. It was an exciting time. Twice a week, I stayed with Mark-Étienne Janet , where he forged our first kilogram samples. Melting of platinum and giving it a form has not yet been worked out. Jeanette - the jeweler of Louis XVI - was a real master in working with platinum (to be exact, with platinum sponges). Just a few years earlier, on June 6, 1782, Lavoisier showed the melting of platinum in a hydrogen-oxygen flame to the future Tsar Paul I; the latter came to visit Marie Antoinette and Louis XVI. Subsequently, Etienne Lenoir made our platinum meter, and Jean-Nicolas Fortin made a platinum kilogram. For readers interested in platinum history, I recommend the book by McDonald's and Hunt .
Platinum is a special metal: it is characterized by high density and chemical inertness. In addition, it is not as soft as gold. The best kilogram standards to date are made of a platinum-iridium alloy (adding iridium to platinum really improves its mechanical properties ). Below is a comparison of some of the physical characteristics of platinum, gold and iridium:
It sounds simple, but at that time the best scientists spent countless hours in calculations and experiments to find the best materials, the best forms and the best conditions for defining new units. But both the new meter model and the new kilogram cylinder were macroscopic bodies. All macroscopic artifacts are difficult to transport (we developed special transport cases); they change quite a bit even after 100 years of use, absorption and desorption, heating and cooling. As a result of the technological progress of the nineteenth and twentieth centuries, it became possible to measure time, mass and length with an accuracy of one billion. And measuring time can be done a billion times better.
I still remember very well that after we made new standards of length and mass, Lavoisier said: " nothing more magnificent and simple did not come out of the hands of man ." I think so now.
Our goal was to create a unit of measure suitable for all. Our motto sounded like: “for all nations - at all times”. We have placed copies of the meter all over Paris, so that everyone knows exactly how long it was (the next time you visit Paris, do not forget to look at mètre étalon near the Luxembourg Palace). Here in this photo, which I recently found, it shows how interested a German tourist is studying the history of one of the few remaining standards of the meter:
It was an exciting time (even if I was not there when the commission completed this work). Our units of measurement were used in most European countries in the nineteenth and most of the twentieth century. We created a meter, a second and a kilogram. Later, four more basic values ​​(ampere, candela, moth and kelvin) were added to the results of our work. With these updates, the metric system has served humanity for more than two hundred years.
The graph below depicts a sharp rise in the frequency of use in books of the words kilogram , kilometer and kilohertz , which occurred after the Metric Convention of 1875:
We defined only meter, second, liter and kilogram. Today, SI includes many more units: Becquerel, pendant, farad, gray, henry, hertz, joule, rolled, lumen, lux, newton, ohm, pascal, siemens, sievert, tesla, volt, watt and weber. Here is a list of dimensional relationships (no physical meaning is implied) between derived units:
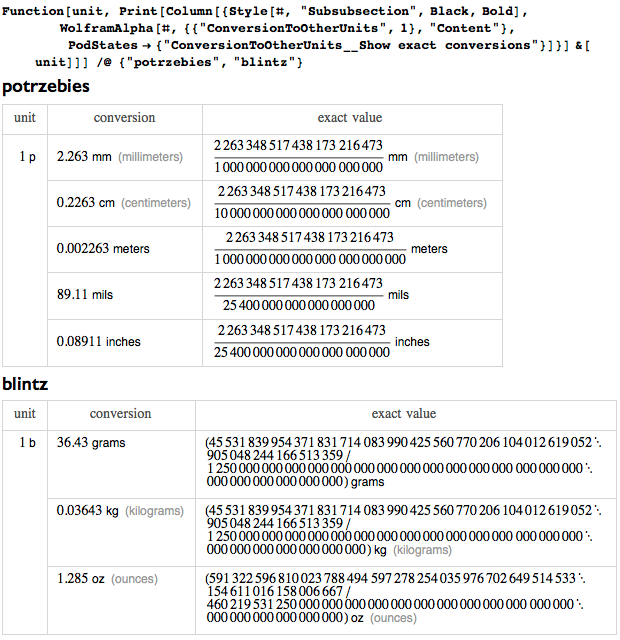
Many nominal units, added after my death, were associated mainly with electrical and magnetic phenomena that were not yet known during my life. And although I’m generally a serious person, I love jokes and puns. However, I don’t like it when they make fun of units. For example, there is a system with units of measurement: potrzebie, ngogn, blintz, whatmeworry, cowznofski, vreeble, hoo, and hah (a system introduced by Donald Knuth (Knuth's Potrzebie system of units)). Not only are their names meaningless, their meanings also have no meaning:
Or look at Max Peterson's proposal for units of measurement for biology . Unit names and prefixes may seem ridiculous, but for me units are too serious subject:
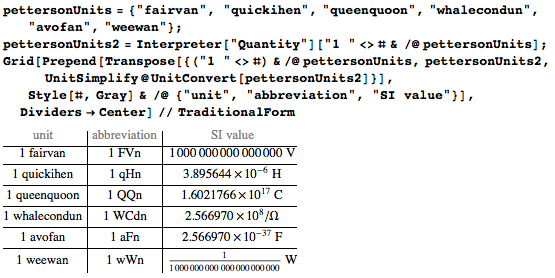
These unit names do not even rhyme with any of the proper names:
I repeat: I like to joke sometimes (even with units of measurement), but it should be clear that this is not serious :
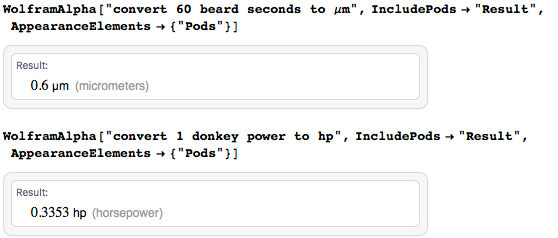
There are still clearly unscientific units, such as “helens” for beauty, “puppies” for happiness, or “darwins” for glory:
I am very proud that SI units are not dead paper symbols, but tools that govern the modern world. Although I am not a fan of comics, I liked the recent presentation of basic units of measurement in the form of superheroes from the National Institute of Standards and Technology (NIST):
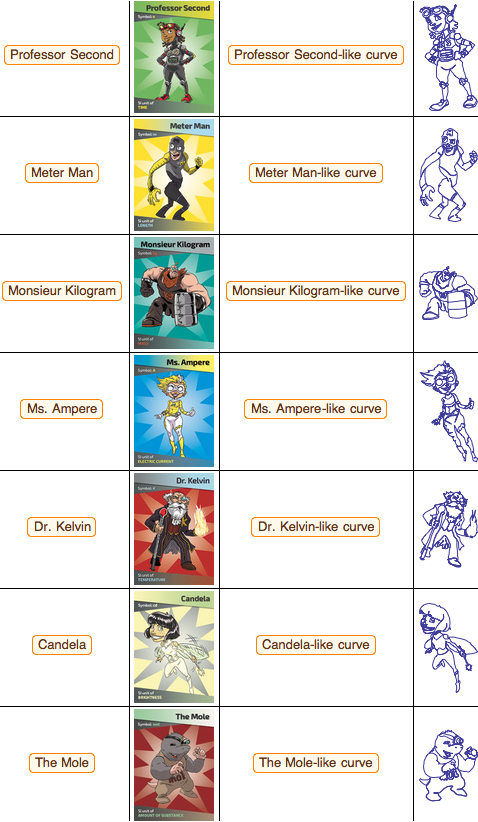
Note that in order to honor the contribution of five great mathematicians to the creation of the metric system, the curves in the rightmost column are given in the form of mathematical formulas; for example, for Dr. Kelvin, we have the following purely trigonometric parametrization:
So we can "build" Dr. Kelvin:
Having such characters in a parametric form is very convenient: when my family gets together, the kids do their favorite thing: paint superheroes. I just print the curves, and then the kids can have fun (I borrowed this idea a couple of years ago from the NCSA coloring book ).
And whenever a new episode comes out, everyone gets together to watch it. As you understand, the last episode is our favorite. Rumor has it that the upcoming book The Return of Metrologists (2018 will be the perfect year!) Complements the existing book .
I am glad that the importance of measurements and the main metric system is celebrated at the World Metrology Day - May 20.
At the time when I lived, people measured only goods: grain, potatoes and other products, as well as wine, fabrics, firewood, etc. So my country really needed units of length, area, volume, and, of course, units of time. I always knew that the importance of measurement will only grow over time. It is quite remarkable that only 200 years after I entered the celestial spheres, the number of measured physical quantities reached several hundred. To date, only the International Organization for Standardization (ISO) defines and describes which physical quantities should be used. Below is an image of the interactive demonstration (download the document at the top of this post to interact with it), which graphically shows the dimensions of physical quantities for subsets of the selected units. First choose two or three dimensions (basic units of measurement). Then the graph will show the spheres with dimensions proportional to the number of different units of measurement, which are obtained from the selected ones. For example, in the case of "meter", "second" and "kilogram", the chart shows such units of measurement of physical quantities as impulse (kg 1 m 1 s -1 ) or energy (kg 2 m 1 s -2 ):
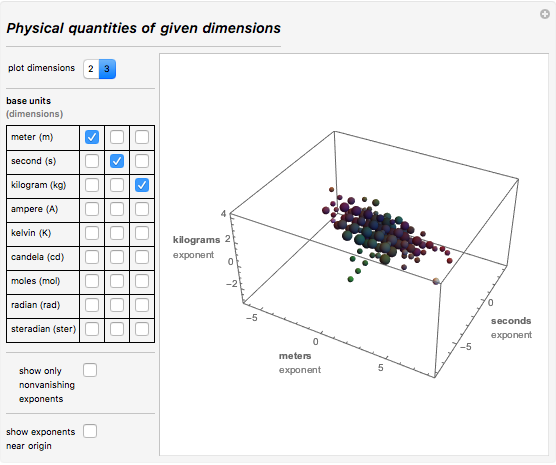
Here is a snippet of code that I used to create these graphs. These are all physical quantities with dimensions L 2 M 1 T -1 . The last one is a slightly exotic unit of electrodynamics.
:
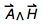Today, with the help of smartphones, ordinary people measure many physical quantities. I would say, "measurements they rule." Or how my dear friend William Thomson liked to say:
... When you can measure what you are talking about and express it in numbers, you know something about it; but when you cannot express it in numbers, your knowledge is scarce and unsatisfactory; this may be the beginning of knowledge, but, regardless of the subject, you are unlikely to get to the stage of science.
Here is a graphical visualization of physical quantities, which are measured using the most common measuring instruments:
Electrical and magnetic phenomena are just gaining popularity. Electromagnetic effects associated with physical quantities became popular much later:
I still remember how excited I was when, in the second half of the nineteenth century and at the beginning of the twentieth century, various physical quantities of electromagnetism were discovered and their interrelationships were understood (and do not forget about the recent addition of the memristor ). Below is a diagram showing the most important electrical / magnetic physical quantities q k that have relationships of the form q k = q i q j :
On the other hand, I was sure that temperature-related phenomena would soon be fully understood. Indeed, only 25 years later, Carnot proved that heat and mechanical work are equivalent. Now I also know about slowing down time and reducing length in accordance with Einstein's theory . However, humanity is still unknown whether a moving body is colder or warmer than a fixed one (or they have the same temperature ). Every week I hear from Willard Gibbs about the related topic of negative temperatures . And recently, he was very excited by the news that the value of the maximum temperature for a given volume V is expressed in fundamental constants :
Maximum temperature of one cubic centimeter:
Increase the number of constants
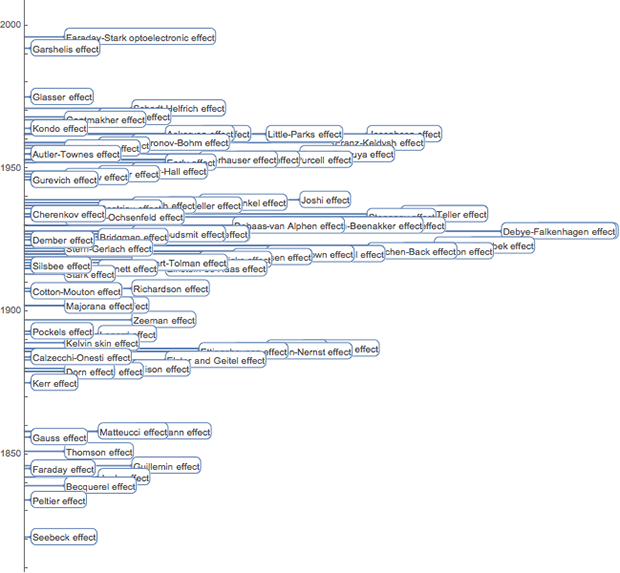
After a long time after my physical death, the best scientists in the field of physics of the 19th and early 20th centuries. - James Maxwell , George Stoney and Max Planck (and Gilbert Lewis ) discussed the units of time, length and mass, based on the constant properties of microparticles and related to fundamental physical constants (speed of light, gravitational constant, electron charge, Planck constant, etc. .):
Maxwell wrote in 1870:
Nevertheless, the size of our Earth and the time of its rotation are characterized by enviable constancy. The earth can shrink, cool, or expand at the expense of a layer of meteorites falling on it, or the speed of its rotation can weaken - and yet it will be the same planet as before.
But a molecule, say, hydrogen, if its mass or time of its vibration changes at least a little, will no longer be a hydrogen molecule.
So, if we want to get standards of length, time and mass that must be absolutely constant, we must look for them not in the dimensions, or movement, or mass of our planet, but in the wavelength, vibration period and in the absolute mass of these imperishable, unchanging and completely similar molecules.
When we see that here, as in the starry sky, there are an innumerable multitude of small bodies of the same mass, not dividing further and vibrating simultaneously many times a second, and when we reflect that no force in nature can now change mass or the period of any one of them - it is then that we seem to have moved along the path of natural knowledge to one of those points where we must accept that faith, through which we understand what all that is visible consists of.
At the time when Maxwell wrote this, I was in heaven for a long time, and when I read it, I applauded him (let me have some skepticism about all the ideas coming from the UK). I knew that this was the way forward to perpetuate the units we created during the French Revolution.
There are many physical constants. And not all of them are known with equal precision . Here are some examples:
Converting constant values ​​with a known error into arbitrary precision numbers is convenient for the following calculations. The relationship between the confidence intervals and the number of digits is given as follows: an arbitrary precision number that corresponds to v ± δ is the number v with an accuracy of -log 10 (2δ / v ). And vice versa: if a number is given with arbitrary precision, we can recreate v ± δ:
11- . G — . , G. , — . , Nature . , , G . Big G Challenge . , .
. , , . ( ), , , -- . .
, — . ( . ). (?) :
, lattice reduction :
, π ?
, 10 — :
, . , , . :
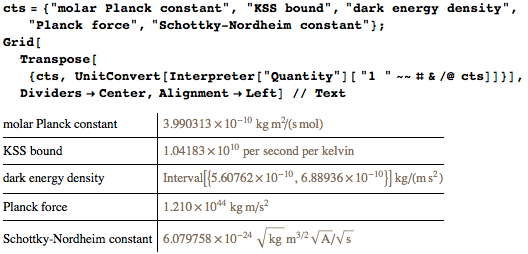
. 100 , :
, . , ( !) , — , :
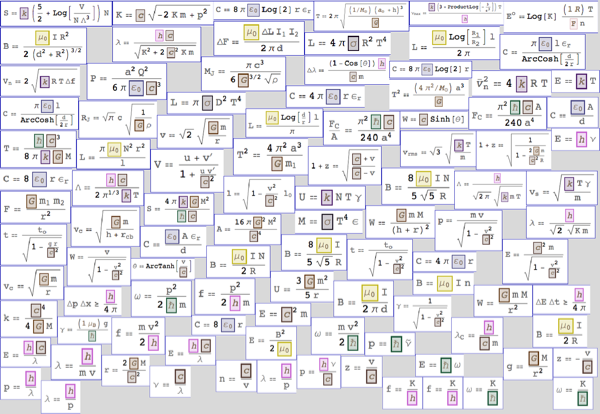
. 3D-, , . :
, — , . — , . , . , . :
, α –h β -G γ αγβγγ. , α · h β · G γ ; , . :
- , : , :
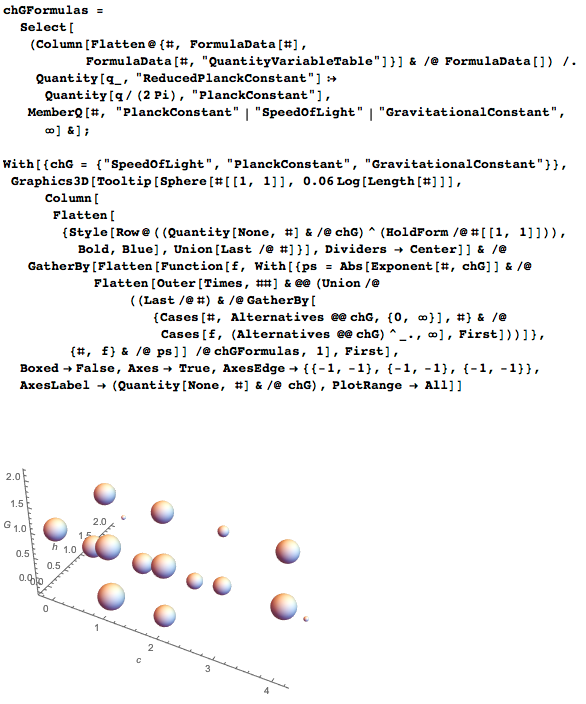
— . , h G :
, , - - ( - ). :
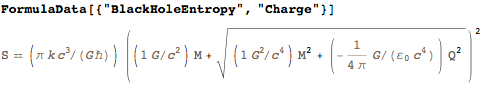
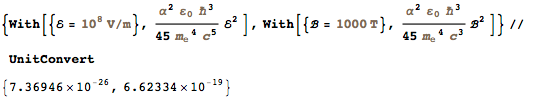
, , , . 1874 ., . , 1906-07 ., ( ), 1900 " ". , G . . :
, , , , , . , , ?
(: , 20 , h . , 1900 . . h/k B . , , ? ).
. . ?
, :
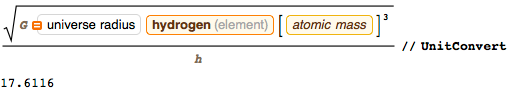
, , . , 10 , arXiv, , , . ( , ) , 2 , — , :
1983 , , (. ):
. , :
:
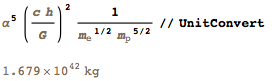
100 , : .
1967 , 9 192 631 770 , -133, , 1983 , , 1/299 792 458 . , , 0 . ; 2,5 - .
. , , , ( , ).
, , ? . , (!) , :
- ( ):
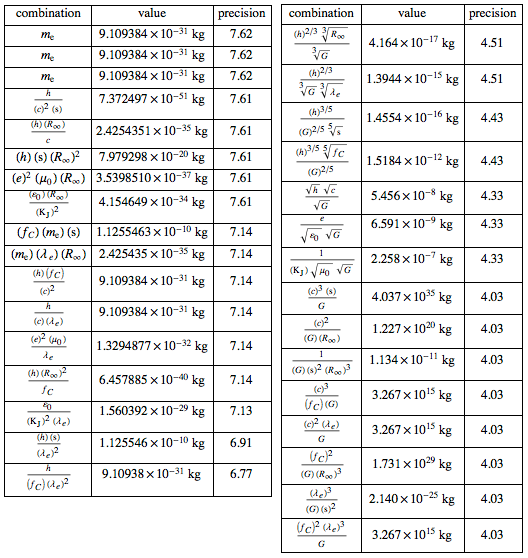
, ? : . , F = m , ( ):
PLANK :
, , , , Wolfram Open Cloud . - (), , (): , , , . , , :
, — — . , , . , , (, , , ). : , 2 = 1/ (ε 0 μ 0 ). :
, , 32 , α = 2 /(4πε 0 ħ ). , , - α . , . , . , . , , , . , , :
:
:
:
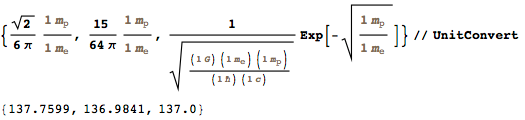
. , , ( ) Springer . :
, , , . Nature .
, , C, G h , , . . h . . :
, . . , , .
In addition to the meter and kilogram, other units of measurement are included in modern SI. An ampere is defined as the strength of an unchanging current passing through two parallel straight conductors of infinite length, kelvin through the thermodynamic temperature of the triple point of water, mol through kilogram and carbon-12, and candela through the radiation of a black body. If you have never read the SI brochure , I strongly recommend that you do it.
Two infinitely long wires - this is definitely a macroscopic level. And to maintain the state of the triple point of water, a macroscopic amount of it is necessary. It is not perfect, but normal. Carbon-12 is already a microscopic level. Radiation of an absolutely black body is again an ensemble of micro-objects. Thus, something of the current SI achieves, in a sense, Maxwell’s goals.
However, the main cause of my insomnia in the past 50 years has become ... kilogram. When I thought about him, my head ached and I was tormented by nightmares.
In 1799 (this is also the year of my death) the first prototype of the kilogram was created - a small platinum cylinder. He was about 39.7 mm in height and 39.4 mm in diameter, and for 75 years he served as the benchmark kilogram. It was created from a wrought platinum sponge made by Janet. Miller gives a lot of the details of this kilogram sample. They are now in the national archives . In 1879, Johnson Matthey , using new melting technologies, made the material for three new samples of the kilogram standard. Due to a slightly higher density, these kilograms were slightly smaller (39.14 mm lower). The cylinder was named KIII and became the new international prototype of the kilogram
. Here is the last phrase from the definition of the international prototype of the kilogram of 1885:
Several kilogram standards were selectively compared with our original standard; if you are interested in the details of measurements, see this book . All three samples were less than 1 mg lighter than the original kilogram. In one sample, the difference was less than 0.01 mg compared to the original kilogram. For a detailed history of creation
see quinn. And still, by definition, a kilogram is the mass of a small metal cylinder lying in the safe of the International Bureau of Weights and Measures not far from Paris (although technically it is not located in France). It lies in a safe that opens with three keys, under three glass domes, a small platinum-iridium cylinder that determines what a kilogram is. To orient you in geography, here’s a map of Paris with the current kilogram benchmark (in the southwest), our original (in the northeast), both in a yellow frame, and some other sights of Paris: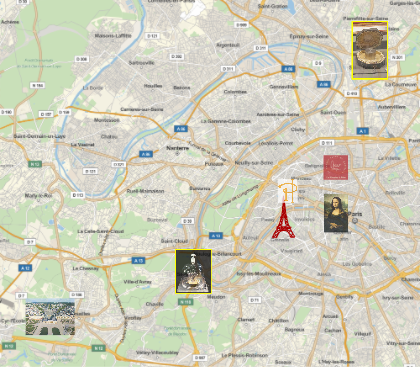
This benchmark kilogram, which made me miserable, is very difficult to access. Every few years a small group of people re-measures its weight (mass). And, of course, the result, according to the definition and the agreement concluded at the first General Conference on Measures and Weights in 1889, is equal to one kilogram.
Years later, the first standard of the kilogram received dozens of brothers and sisters in the form of national standards of other countries (of the same size, material and weight with an accuracy of several micrograms, which are carefully recorded). It is a pity that the Internet has not been invented before, because in that case I would have a communication channel to say what happened to the abducted Argentine benchmark 45 ; after that it was melted down. Immediately after creation, they had the same size and weight. It could be assumed that the weight of these cylinders will be stable. However, history judged otherwise. Repeated measurements showed that almost all the standards became harder every year. Or, more likely, this international prototype has become increasingly easy.
For many of these comparisons, I watched with great interest from my sky. Comparison of their masses presented significant difficulties. First, you must first deliver the national prototypes to Paris. I silently listened to lengthy discussions with TSA members when the metrologist came with a platinum kilogram, materials for which cost about $ 50,000 (you need to add another $ 20,000 for manufacturing), which lay in a brilliant golden container and which should only be opened in a clean room with gloves and with a mouthpiece, and so that the human hand never touched it), and explained all this to the TSA members (an official letter is in this case a great help). What I saw here was even funnier than the scenes from the movie 1001 grams .
Then comes the complicated cleaning procedure with hot water, alcohol and UV radiation. All samples lose weight in this process. Then they are all carefully compared with each other. And as a result, all of this with a high degree of probability leads to the fact that my favorite international standard kilogram is losing weight. This fact deprives me of sleep.
Here are the results of the third verification (from 1988 to 1992). The figure shows the difference in weight compared with the international prototype:
To find out about measurements taken in the last two years, see this article .
Saying that a kilogram is “losing weight”, I mean the following. By definition (independent of its “real objective” mass), an international standard has a mass of exactly 1 kg. Compared to this mass, most of the other kilogram standards seem to be gaining weight. Since other standards were created gradually over more than 100 years using various methods, it is very likely that the international standard becomes easier over time (this is not due to Ceausescu's greed stealing platinum; in 1889, the Romanian prototype was lighter than the international standard kilogram).
Willard Gibbs, my old friend, often says that in his home country he still uses a pound, not a kilogram. His vote in the elections this year will obviously be for Bernie . At the very least, a pound makes up a certain percentage of a kilogram, so everything that will happen to a kilogram will affect a pound in the same way:
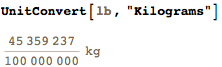
New SI
But soon all my dreams and centuries-old hopes will come true, and I will be able to sleep again. In 2018, that is, just two years later, the most ambitious (starting from my work with Laplace) changes in the history of units and dimensions will occur.
All units of measurement will be based on things available to everyone and everywhere (meaning access to some modern physical devices and devices).
Below, on Earth, they began to call them " reference constants ".
Some people also call the new SI "quantum SI" because of its dependence on Planck's constant and the elementary charge e . In addition to the importance of the Planck constant ( h ), the following quantum effects are related to h and e in quantum mechanics: the Josephson effect and the associated Josephson constant K j = 2 e / h , as well as the quantum Hall effect with the Klitzing constant R K = h / e 2. Quantum metrological triangle: connecting the frequency and electric current by tunneling single electrons, connecting the frequency and voltage through the Josephson effect and connecting the voltage and electric current through the quantum Hall effect will be an excellent realization of electr in many years, as Penin noted, we will have to worry about the effects of QED of the second order , but this will not be soon).
BIMP already has a new logo for the future International System of Units:
Specifically, the sentence is:
- The second will still be determined through the microwave radiation of the cesium atom.
- The meter will still be determined through a precisely defined speed of light.
- The kilogram will be determined by the exact value of the Planck constant.
- Ampere will be determined through a precisely defined amount of elementary charge.
- The kelvin will be determined by the precisely determined value of the Boltzmann constant.
- The mole will be determined by the exact (calculated) value.
- The candela will be determined by the exact value of the candela ratio of steradian-per-watt at a fixed frequency (already used in some cases).
I strongly recommend that you read the brochure of the new SI project . Laplace and I discussed it a lot, and we liked it. Here is a word cloud from the new SI brochure:
Until I forgot: below are some comments regarding other units.
Second
I still remember when we discussed the introduction of metric time in the 1790s: a 10-hour day, 100 minutes in an hour and 100 seconds in a minute. Looking back, I understand that it was not such a good idea. People’s habits are sometimes too hard to change. And I am so glad that in recent years I was able to interest Albert Einstein in metrology. We talked a lot about the value of time and the difference between measured local and coordinate time. But we will not talk about this today. The uncertainty of the second is today less than 10 -16 . Maybe one day, cesium will be replaced with aluminum or another element to reduce the uncertainty by 100 or even 1000 times. But this does not change the spirit of the new SI; these are minor technical changes (if you are interested in the details, see this article ).
Obviously, today's definition of a second is much better than the previous one. At a time when prices in the stock market are matched at the level of microseconds, the change in the length of a day due to earthquakes, melting glaciers, continental drift and other phenomena occurring for more than a hundred years turns out to be quite large:
Moth
I heard that some chemists complain that the mole (their favorite unit, introduced in the SI only in 1971) will be debased. In the currently used SI mole is determined through the number of atoms in carbon-12. The new SI will be talking simply about the counting of objects - the true chemical equivalent of the devil's dozen. Based on the Avogadro number, the mole plays an important role in connecting the microcosm with the macrocosm. A more mundane determination of a mole depends on a given quantitative value — for example, on a pH value. Second is the basic unit of time in the SI; mole is the basic unit of physical quantity or quantity of a substance :
However, the term " amount of substance " is not everyone likes. Even this year (2016) alternative names have been proposed: for example, stoichiometric amounts . In recent decades, it has been proposed to replace the entire variety of names with the phrase “quantity of a substance”. Here are some examples :
But in SI, only the name of the unit of measurement, “mol,” is determined. The naming of a physical quantity, measured in moles, remains the International Union of Pure and Applied Chemistry .
To see the latest discussions of this year, see Leonard's articles: " Why is the" amount of substance "so poorly understood? Avogadro's mysterious fault! "
But what if we could create an “ideal cube” that would represent the Avogadro number? It would be easy to conceptualize. This option was proposed several years ago - a variant compatible with the Avogadro constant value (the result would be a cube with an edge length of 84,446,888 elements). During a game of celestial cricket, I asked Srinivas Ramanujan and Harold Hardy , his long-time friend, that he was special in the number of 84,446,888, but so far they have not invented anything deep. He said that 84446888 = 2 3 * 17 * 620933, and the number 620 933 appears from the position of 1 031 622 decimal places of the number π, but I personally do not see anything related to metrology in this.
Here is the last CODATA value (Committee on Data for Science and Technology) from the list of constants, units of measurement and uncertainties of the National Institute of Standards and Technology :
The above cube is too small.
Interestingly, if we agreed on the option of a body-centered lattice with one additional atom in the unit cell, then we could still stick to the interpretation of the cube:
A face-centered grille will not work:
But the diamond (silicon) lattice will be:
Summarize:
Here's another little thing:
At the height of the Cold War, the accepted Avogadro number changed in the third digit. This was a major change, given that there is currently a protracted dispute over disagreements over the sixth grade. Can you explain the sudden decrease in the Avogadro number during the Cold War?
Do you know the answer? If not, look here or here .
However, I went away from the main idea. Since I am more interested in the units of measurement of mechanical quantities, I will give my old friend Antoine Lavoisier to judge the new definition of a mole, since it was he who was the chemist on our team.
Kelvin
Willard Gibbs convinced me that temperature must be determined mechanically. I'm still trying to understand what John von Neumann thinks about it (since I never fully understood his evening lectures on factors of type II and type III, my opinion about kelvin did not work out). Different temperatures correspond to nonequivalent representations of algebras. I did not think about how best to determine Kelvin from the point of view of algebraic quantum field theory. I asked John about his opinion on h / k's first-principle assessment, based on the theory of Tomita-Takesaki, and even he was not sure about that. He told me something about the thermal time and temperature of a diamond, but I didn't understand much of that.
And then we have the possibility of obtaining the value of the Boltzmann constant. Even 40 years after the publication of the Kopp-Huber article , it is not clear whether this is possible. I have already thought about this, and I take into account the various options . As I have already said, it is not clear to me how to determine the value of temperature and its measurement units. I have no doubt that the new definition of Kelvin will be a big step forward; only here last?
Ampere
This is one of the most direct, intuitive, and beautiful definitions in the new SI: the current is just a certain number of current electrons (per second). Determining the value of the ampere in terms of the number of elementary charges is simply ingenious. When it was first proposed, Robert Milliken was so happy that he invited many of us to meet in the afternoon in the courtyard of his house. Both in theory and in practice, when measuring the electric current of electrons in crystalline objects, we must remember that electrons are quasiparticles. However, since 1959, thanks to Walter Kohn, we know that we should not expect that the charge of an electron in a crystal will be the same as the charge of a bare electron . Since the elementary charge is a fairly small charge, the question of measuring fractional charges is not relevant now. Personally, I think that Robert’s contribution to the definition of physical constants at the beginning of the 20th century is clearly underestimated (Robert Andrews always knew what he was doing ).
Candela
Does this unit deserve to be among the main ones? The whole history of person-centered physiological units is rather confusing. Obviously, they are extremely helpful. We all see and hear every day, every second. But what if the human race continues to evolve (in the context of Darwin’s ideas)? How will this fit our mantra "for all time"? I have some thoughts on this subject, but to lay them out here and now would mean taking the narrative away from the main topic for discussion.
Why are the basic units of measurement exactly 7?
I want to note that at first I was very concerned about the introduction of some additional units of measurement used today. In the process of endless discussions, Gauss (my chess partner) managed to convince me that we can reduce all measurements of electrical quantities to measurements of mechanical properties, so I ended up with a good understanding of the GHS system, which I didn’t like at first. However, the system of units created by man should be as useful as possible, and if these seven units perform their functions better than anyone, there should be seven of them. In principle, you can even exclude the unit of measurement of mass and express the mass in terms of time and length . However, besides the fact that it is impractical, I am sure that this approach is conceptually wrong. I recently discussed this issue with Karl Friedrich. He said that in the late 1820s. the idea was to simply use time and length, but this approach was later abandoned. During his lifetime, Gauss did not have the opportunity to discuss the concept of mass with Kant , but over the last century they agreed on mass as a priori (at least in this universe).
The motto for the original metric system was the phrase: "for all people, for all time." The current SI is already implementing the first part of the motto - “for all people”, and taking into account the upcoming changes, the second part will soon become a reality. You can't imagine what that means to me. It seems that the fundamental constants can vary as much as possible by an amount equal to about 10 -18 per year. This is many orders of magnitude farther from what is currently available for most units of accuracy.
Of course, in the new SI some values ​​will be more cumbersome. If we consider the current CODATA values ​​as accurate, then, for example, the von Clitzing constant e 2 / h will turn out to be rather voluminous:
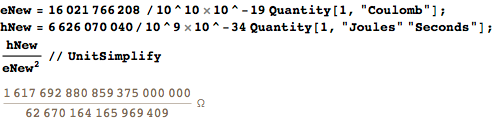
The whole part of the latter result is, of course, 25.812Ω. — ? :
— , , , . , , ( , . ).
, ( ) ÎĽ 0 4Ď€10 -7 N/A 2 ? . :
h μ 0 α . , α :
So what? . .
, μ 0 , — Z — :
, 225 , , . , , .
, , , , 1790- . , , , . , . , , , .
, . , . . , , , , , , , , , . , , . , , . — - . , , , , , - : . . , , .
, ( ) , . , «» . , .
: ! . , SP 811 : « — , ». , .
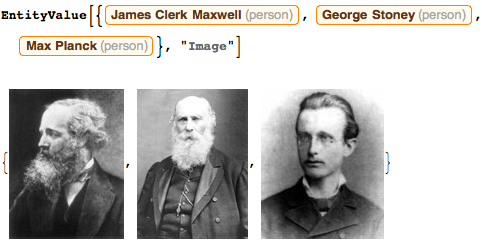
, . , . . , ( ) . , . ( ). , , . , , , , ( , , ). , , , . , - , . , , : (6,62… ×10 34 · ), (6,02… × 10 23 /), (6,6… × 10 -11 3 /( · )) 6. , , , , .
, , 66% (2443 , ). , .
G (m / (h)) 1/2 . :
, . ( ), .
: Sinfonia de motu , , C, G, E, H (- ), ( ). .
, , . .
, . , , , — . : , . .
CODATA :
. .
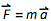. , ( , ). What's next? 1912 :, , , , , , , … , , , ; , … [] , … - … , , , , , … , , .
:
, ; ; , . , , .
, . , - : — .
. , — , .
« », , ( , ), 5 . , , -, - . , ( , ). , ; , . , , , ( ). . , :
- .
, , . : . , speed max ~ ln ( mass ). , ( )::
: ( ). , , , :
.
:
; , 6.62606X * 10 -34 , s -1 · m 2 · kg, J · s. X , .
. , , , . " " 1791 , 1793 , , , - , . . , . , , , , , . , :
, , , , 50 , , . , , , .
, , " " .
, , 12 . - , ( ), n = round(1 / m ( 12 )) , 1 , n -12 . ( E = m c 2 ), , 10 -10 . - , :
1 - .
: () , :
, . , m ( 12 C ), u = m ( 12 C )/12; , u :
. , -12 , . , .
, . 20 , .
11 , , , " : , " Metrologia ( , Hells 'Press, Heaven, Jannah Media Deva University Press), , . , .1 , h , , . , , .
1873 .:
, , , ; , , .
2005 , , .
(, ) ( , HHS (« „)), : E = mc 2 E = hf m , m = hf / 2 . , h , , m ( , , , E = mc 2 - , , — - , . , , Δm Δt ~ h/ 2 , , . , , 10 -52 m 1 .).
, f = m 2 / h , , , f~1,35Ă—10 50 , . , , , . 25 ( ) , , .
, h . . h . , h 10 -8 , h . . , . 100 50 ÎĽ ( 5Ă—10 -8 ), 2Ă—10 -8 , .
116 7 . ! “ » h , — ( , , b h):
, h, , . , , ( ). , h , Hilfsgrösse ( ); , , , , .
1919 :
6,55×10 -34 J · s 2016 6,626070073(94)×10 -34 J · s: .
, h . ( ) , ( h . ):
: - . , , -. , , , . LEGO « ». ! , . , , , , .
, , , . (99,995%) . N h . , , 11 ; , N h h . . ; , 20 , :
, . 99,995% 28:
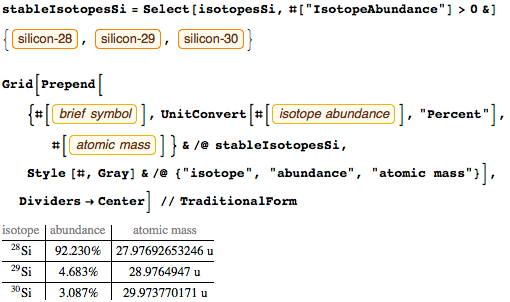
10 -11 , , . , -.
, . , .
- , , , . , - c . , ! .
, . — ; , - . , ( ). . , 2010 , , . ; , . , - ħ , BZO (--). . , (, « » ), , . , . , .
, ( ) h . , , — . , 15 . , 1930- . . . , , . . h :
( ) . 4 h :
— . . , , . (, CODATA , , ). . , , , , — 1 2017 .
- ,
, h 3D-, . h , 200 :
3D- . ( 2018 ), ( ; , , , 5000 , ).
Toh3D:
, , , . 2018 , . XXI .
If you have any questions or have any comments - feel free to contact me at jeancharlesdeborda@gmail.com; Thanks to the latest advances in technological effects (EPR = ER), we have a faster connection with the Earth.
Ă€ tous les temps, Ă tous les peuples!
Source: https://habr.com/ru/post/326390/
All Articles