Building perfect optics in Zemax
Building perfect optics in Zemax
Introduction
More and more modern automation systems are equipped with optical devices for solving problems of positioning, recognition, observation, etc. Building ideal optical systems using the Zemax calculation program can be useful to non-professionals, for example, to better understand the theory, features of optical devices and perform approximate calculations of optical systems . This paper discusses techniques for building perfect optics in the Zemax environment, gives examples of calculating the autofocus range of a camera, constructing an equivalent circuit for an MGT 2.5x17.5 monocular, a SUNNY P13N05B lens of a Huawei P7 smartphone, and replacing ideal optical elements with a real one.
Perfect optics
The image in perfect optics, in which there are no distortions, is built according to the laws of paraxial optics. The term paraxial means "near the axis." Paraxial optics are well described by linear expressions, which at small angles are replaced by linear equations. In the paraxial region, any real system behaves like an ideal.
Calculations of ideal lenses in the Zemax environment are performed with the assumption that the lenses have paraxial properties not only near the axis, but also on the entire working surface, which acts as an ideal thin lens with a single refractive index of air.
Paraxial optics should be used as a reference with which the aberrations (distortions) of real optics are compared.
Care should be taken to transfer the results of calculations of paraxial optics to real systems, especially when building systems where properties near the optical axis and at a distance differ significantly.
A number of techniques for reducing aberrations and overall dimensions of lenses have been developed: the use of non-spherical surfaces, composite lenses, heterogeneous optical materials, etc. But how would a real lens (Petzval, Gauss, Barlow, ...) not be arranged its characteristics can only approach characteristics of an ideal lens.
')
Build image by collecting lens
Consider the case when rays from each point of the object plane diverge in all directions as from point sources. From the extreme point of object A, as shown in Fig. 1. Only the rays that are focused by the lens will hit the corresponding point B on the image plane. The number of rays of the object falling into the image plane is proportional to the diameter of the lens. The more rays from the subject falls into the image plane, the higher the brightness of the image.
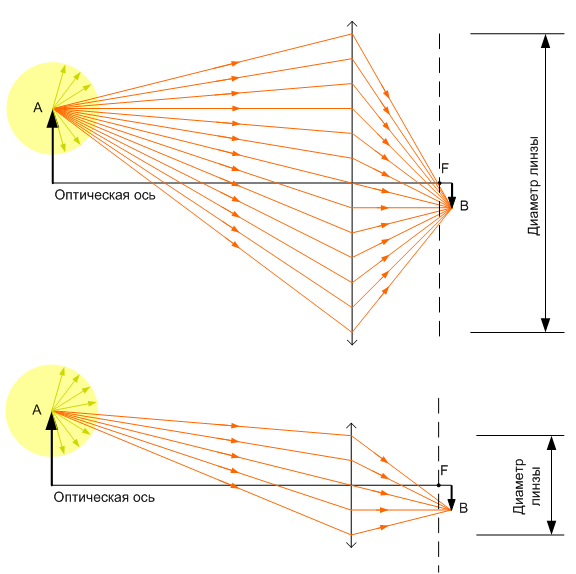
Fig. 1. Conjugate points. The path of the rays from the point of the object to the corresponding point
images on the plane of the photodetector.
To minimize the computation of finding the image, consider the course of only a few rays, for example, as in Fig. 2: beam from the object along the optical axis; the beam passing through the center of the lens and the beam parallel to the optical axis, refracted by the lens and passing through the main focus of the lens (point F on the optical axis).
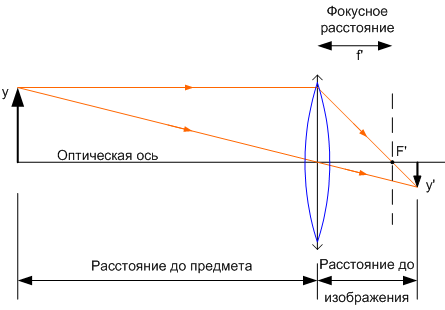
Fig. 2. Minimum constructions for finding the distance to the image plane, image size and lens magnification. For paraxial optics, the longitudinal increase (due to distances) is equal to the square of the linear increase (perpendicular to the axis), and the angular increase is inversely proportional to the linear.
Communication distance to the subject and image. Depth of field
The construction of the relationship between the focusing zone of the lens and the depth of field in the space of objects [1] is shown in Fig. 3. When the distance to the object is infinite, the plane of the focused image passes through the main focus (the displacement of the image plane relative to the focus is zero). The minimum depth of field in the space of objects is achieved at the maximum distance of the image plane (in the focus area) relative to the main focus.
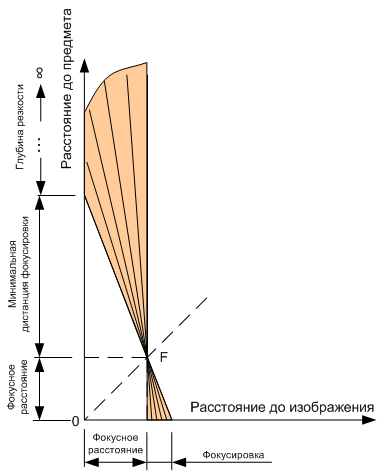
Fig. 3. The relationship between the focus area of the lens and the depth of field in the space of objects.
Zemax design environment features
The Zemax environment functions most commonly used in the design of optical systems are assigned to individual buttons on the main menu. The purpose of these buttons is shown in Fig. four.

Fig. 4. Zemax program interface.
The types of surfaces of elements of optical systems, the radii of surfaces, the distances between elements and other parameters are entered into an editor table, in which each row contains the parameters of one element. The connection of the parameters of the table and elements of the optical scheme is shown on the example five.
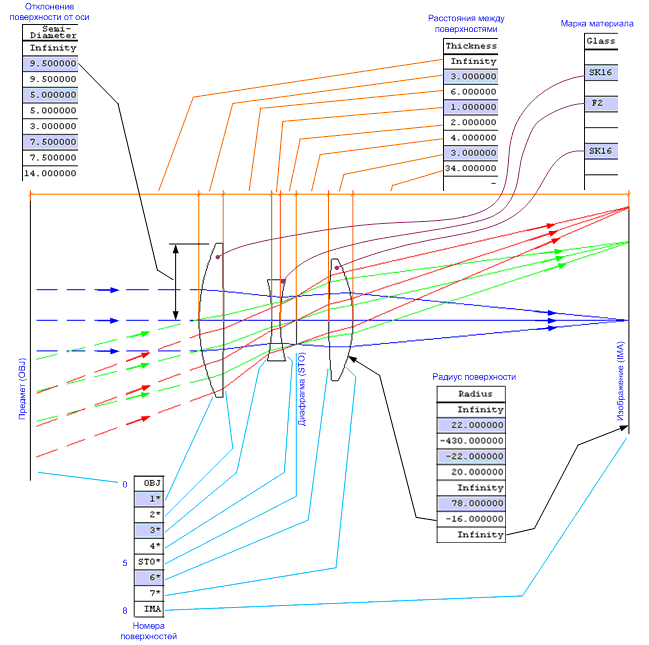
Fig. 5. The connection of the optical scheme with the parameters of the table.
The perfect lens in Zemax
To simulate a paraxial lens in Zemax, you need to set the focal length and, if necessary, enable the calculation of the difference of optical paths passing through the lens (set the OPD mode status to 1 in the corresponding row of the editor table). By default, OPD calculation is not performed (OPD status is zero [2]).
We construct an ideal lens in Zemax, for example, with an entrance pupil diameter of 10 mm and a focal length of 15 mm, which collects the parallel rays of a distant object at one point.
1. Open a new table: menu> button
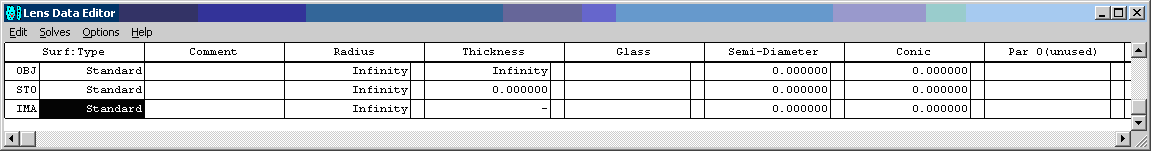
Fig. 6. The initial state of the table of the optical scheme of the Zemax editor. The rows of the table (NN 0; 1 and 2) contain the parameters of the object OBJ, the aperture stop STO and the image IMA.
2. Add a surface between the aperture and the image: select the last line in the IMA line> Lens Data Editor> Edit> Insert Surface menu
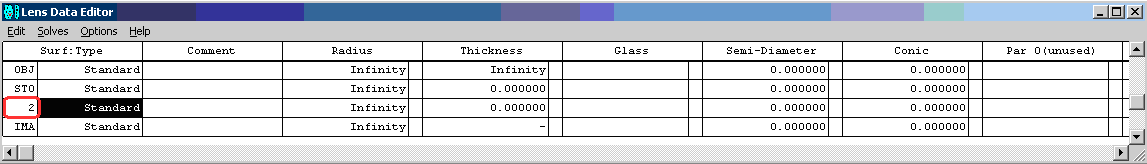
Fig. 7. Added standard surface N2.
3. Choose “Paraxial” surface type: line N2> Surf column: Type> properties window - Properties> Surface Type> Paraxial
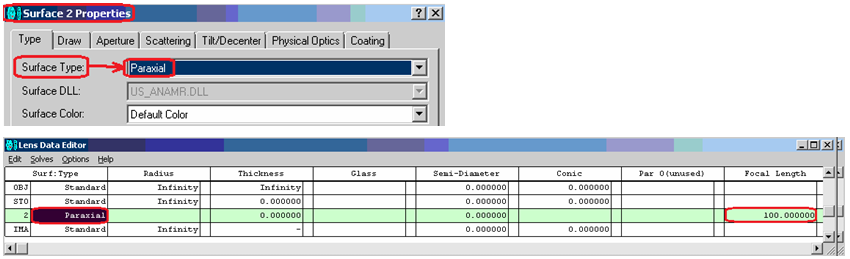
Fig. 8. The N2 surface is changed to an ideal (Paraxial) lens with a focal length of 100 mm. The distance between the lens and the image is zero. The distance between the lens and the STO diaphragm is also zero.
4. Change the focal length from 100 (default) to 15 mm in the column of the Focal Length table
5. Set the distance 15 mm from the lens to the image in the Thickness column.
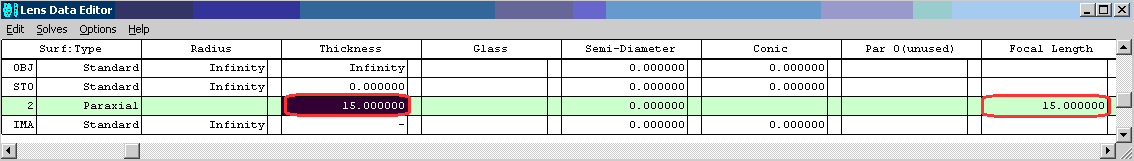
Fig. 9. The focal length of the lens is changed to 15 mm. The distance between the lens and the image is increased to 15 mm.
6. Let's set the diameter of the entrance pupil 10 mm: Main menu> button> tab Aperture> Aperture Value> 10
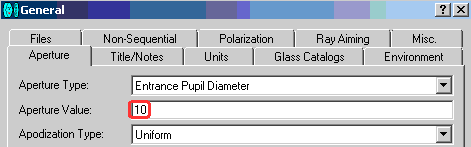
Fig. 10. The diameter of the input aperture of the optical scheme is specified: 10 mm.
7. Let's build the optical scheme: Main menu> button
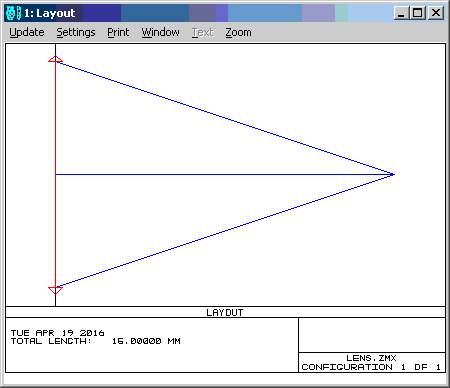
Fig. 11. Optical layout in the Layout window. The coordinates of the aperture and the lens are the same (the distance between them is zero). The coordinates of the mouse on the diagram (in the scale of the optical scheme) are displayed in the picture header.
8. The Layout diagram does not show the rays to the left of the ideal lens (highlighted in red) coming from an object located at an infinite distance, which is designated as Infinity in the Thickness column of the OBJ zero line of the table. To show a part of these rays at the lens entrance, we introduce a surface at a distance, for example, 7 mm in front of the aperture stop STO.
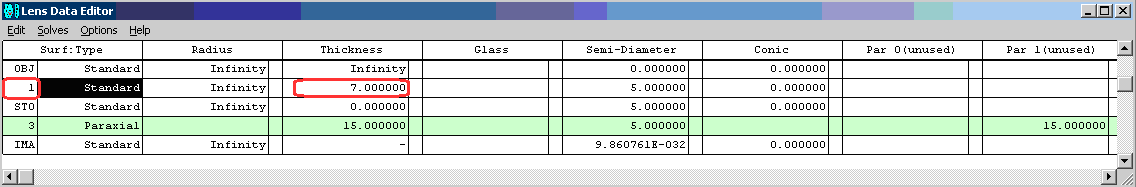
Fig. 12. Added surface in front of the aperture stop STO.
9. Add surface 1 to the displayed part of the optical scheme and increase the number of rays to 7 for clarity: menu of the drawing Layout> Setting> First Surface = 1> Number of Rays = 7.
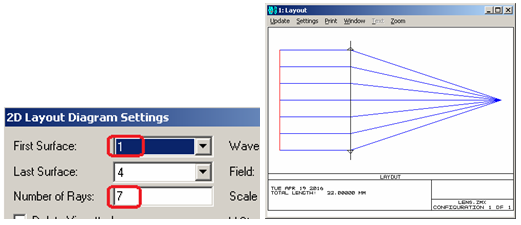
Fig. 13. Shows the rays on a segment of 7 mm to the aperture. Increased the number of rays from 3 to 7.
10. Make the first surface invisible: row N1 of the table> Surf column: Type> properties window - Properties> Draw tab>
11. Update the Layout window of the optical scheme via the main menu button or double-clicking in the area of the scheme window.
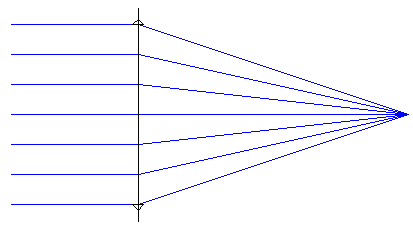
Fig. 14. The first surface of the optical circuit is made invisible.
In the Layout window, you can track changes in the tabular parameters of the optical system and the parameters of the main menu, shown in Fig. 4 and Fig. five.
Smartphone Camera Lens Model
To build an ideal model, take the composite lens of the SUNNY P13N05B camera of the Huawei P7 smartphone (Fig. 15). The lens of the smartphone consists of five plastic elements. An example of a composite lens is shown in Fig. sixteen.
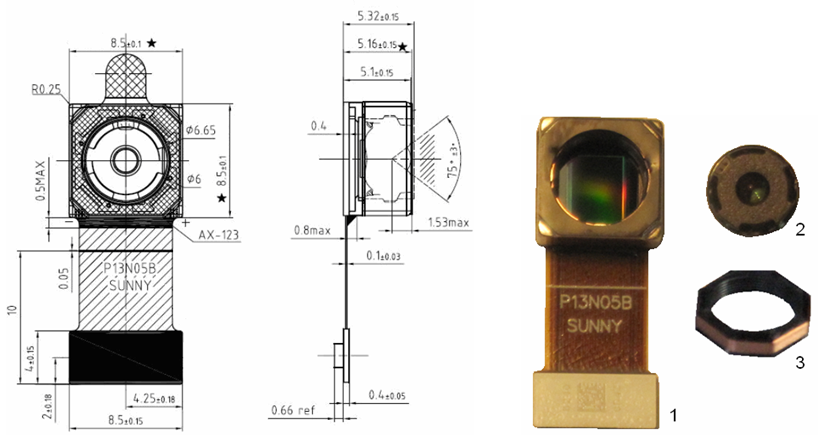
Fig. 15. Dimensions [3] and photographs of the SUNNY P13N05B camera with a SONY IMX214 13 MP photodiode array. 1. - camera module with photodiode array; 2- camera lens; 3 - autofocus drive coil - moving the lens relative to the sensor matrix.
The camera P13N05B has the following characteristics.
• Lens size: 1/3 "
• Size of photodiode array: 6.1 mm (H) × 4.5 mm (V)
• Diagonal core: 5.9 mm
• Composition of the lens: 5 plastic elements (see. Fig. 16)
• Focal length: 3.79 mm
• Aperture number (f / #): 2
• Field of view: 75 ° ± 3 °
• Depth of field: from 7 cm to ∞
• Autofocus Drive Range: ≥ 0.24mm
Fig. 16. An example of a composite lens. IPhone 6 lens.
The parameters of the optical scheme of an ideal camera lens (see Fig. 17) are specified in the Lens Data Editor table and in the windows of the Zemax main menu buttons. The function selected from the list of functions of the selected cell in the Thickness column of the table automatically sets the best distance between the lens and the image. To construct the best image of an object distant to an infinite distance, the plane of the photoreceiver must pass through the point of the main focus at a distance of 3.79 mm from the lens.
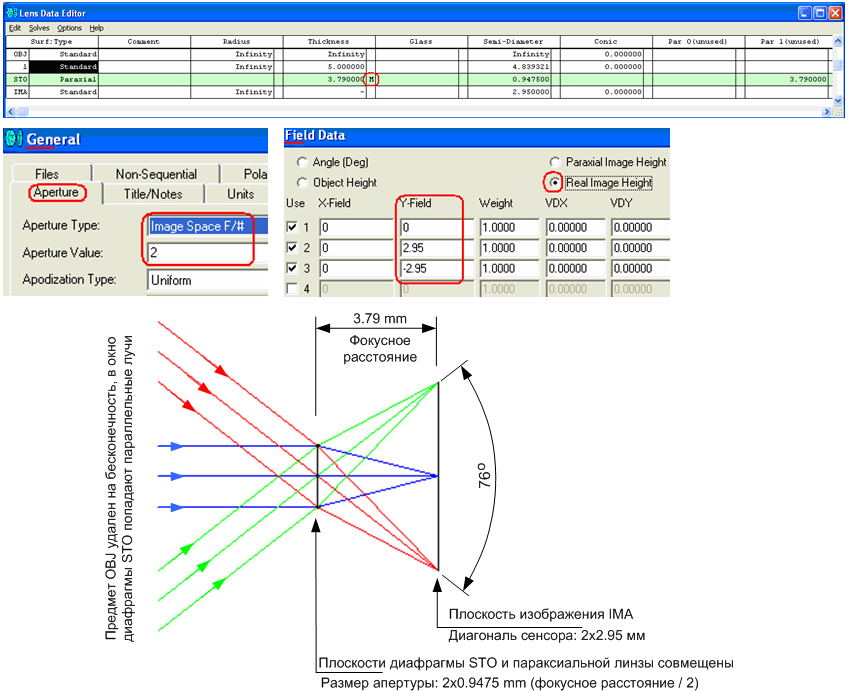
Fig. 17. Optical scheme of a paraxial lens of a photographic lens. Item removed to infinite distance.
Moving the object to the lens by 10 mm while maintaining the viewing angle of 76 ° / 2 in the Field Data window (Fig. 18) increased the distance between the lens and the image to 6.10 mm. Therefore, the change in autofocus when an object approaches from infinity to 10 mm is 2.31 mm (like 6.10 mm - 3.79 mm).
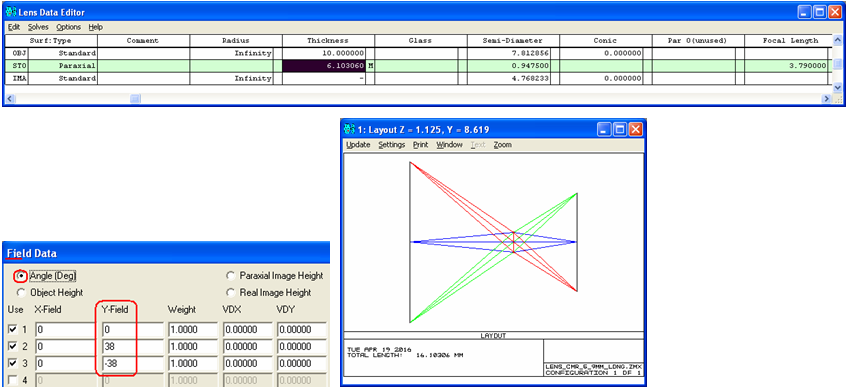
Fig. 18. Building rays from an object located 10 mm from the paraxial lens of the camera and finding the position of the autofocus.
In the specification of the camera P13N05B indicated that the depth of field in the space of objects is in the range from 7 cm to ∞ (infinity). Set the object at a minimum distance of 70 mm from the lens aperture. Zemax sets the distance between the lens and the image plane of 4 mm (see the selected cell of the table in Fig. 19). Thus, to build a high-quality image of an object located in the zone from 7 cm to ∞, it is required to change the distance between the lens and the photosensor from 4 to 3.79 mm. The required 0.21mm change is covered by a 0.24 mm camera autofocus range.
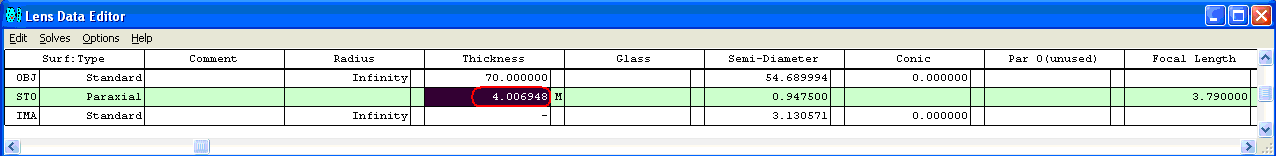
Fig. 19. The distance to the image is 4 mm when the distance to the object is 70 mm. The focal length of the lens is 3.79 mm.
Dependence of the focus range on the focal length of the lens
Focusing area depends not only on the distance to the subject, but also on the main focus of the lens (lens). In Fig. 20 shows the geometry of finding focus areas for lenses with the main focus F1 = 7.5 mm and F2 = 19 mm and the positions of the object in the range AB = 35 ... 52 mm. To adjust the sharpness with the lens F1, you need to change the distance between the main focus of the lens and the image plane in the 0.8 mm range, whereas for the lens with F2, this range increased to 12 mm.
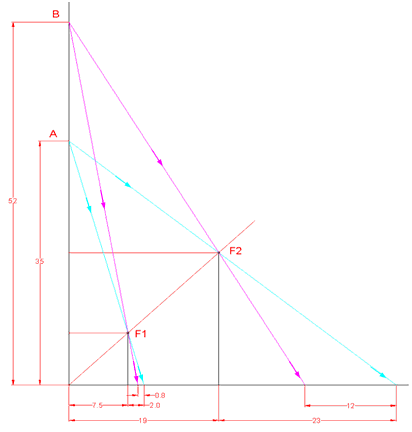
Fig. 20. An example of building focus areas for lenses with different focal lengths F1 and F2.
Ideal telescopes
The comparative dimensions of the Kepler and Galileo telescopes for the same magnification F1 / F2 are shown in Fig. 21. Kepler telescope with collecting lenses gives an inverted image. Galileo's more compact telescope includes a diffusing lens and gives a direct image.
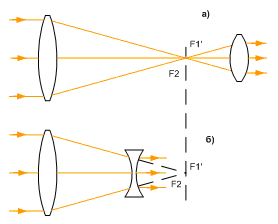
Fig. 21. The scheme of Kepler telescopes (a) and Galileo (b) with the same magnification F2 / F1.
The miniature monocular of MGT 2.5x17.5 USSR, LZOS (Lytkarinsky optical glass factory) is assembled according to the Galilean scheme (Fig. 22). It has the following characteristics.
• Increase: 2.5 times (times)
• Lens diameter: 17.5 mm
• Field of view: 13.5 degrees
• Resolution: 15 ang. sec
• Eyepiece focusing limit: -5 ... + 5 diopters
• Overall dimensions: 22 x 38 mm
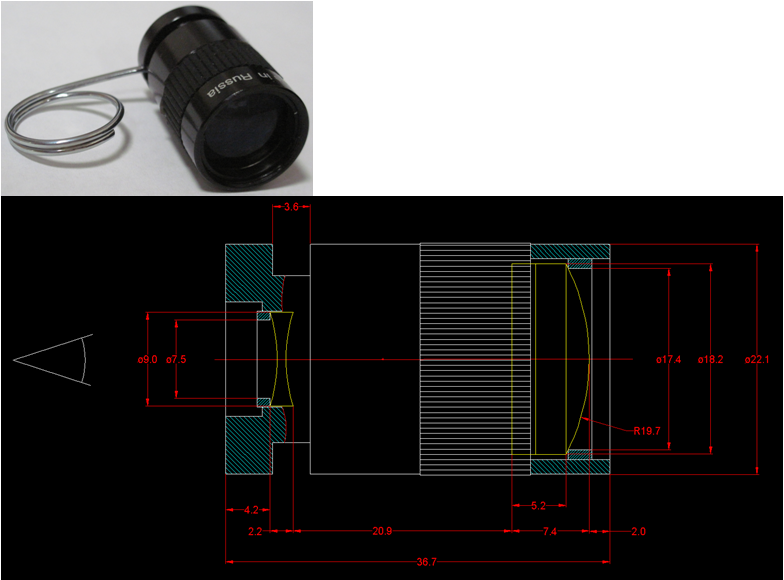
Fig. 22. Type and approximate dimensions of a miniature MGT 2.5x17.5 monocular. Item is on the right.
The equivalent ideal optical scheme of the MGT 2.5x17.5 monocular in ZEMAX is shown in Fig. 23. The scheme consists of a collecting and diffusing lens with the main foci of 37.5 mm and -15 mm, respectively, having a ratio of 2.5 times. Diameter of the collecting lens is 28,75 mm.
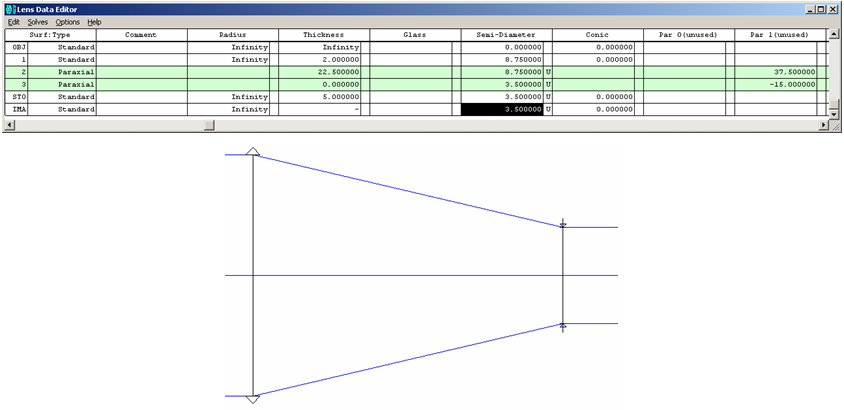
Fig. 23. Tabular data and an ideal optical scheme of a monocular MGT 2.5x17.5. Parallel rays go from the object to an infinite distance.
The option to replace the real paraxial lens
Replace the first paraxial lens (diameter: 17.5 mm; focal length: 37.5 mm) of the monocular with an achromatic lens from the Edmund Optics catalog [4]. To minimize the selection of lenses set the following conditions: category - Achromatic Lenses; diameter - 18 mm; effective focal length EFL 30-39.99 mm; wavelength range - 425 - 675 nm.
Nearest lens required: 18mm Dia. x 35mm FL, VIS 0 ° Coated, Achromatic Lens, Stock No. # 47-706 (part number).
To build an achromatic lens in Zemax, from its specification we take the parameters listed in Table 1. Parameters can also be found in the PDF drawing drawing of the Edmund Optics website [4] or in Fig. 24
Table 1. Edmund Composite Achromatic Lens Parameters # 47-706
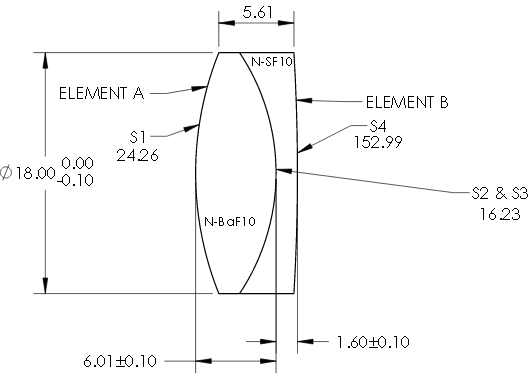
Fig. 24. Drawing of the Achromatic Edmund Lens # 47-706.
Replacing the parameters of the first lens of an ideal telescope (row N2 of the table in Fig. 23) with an Edmund lens # 47-706 gives the option shown in Fig. 25

Fig. 25. Option telescope optics with a real achromatic lens. The distance between the lenses highlighted in the table in red is found by manually shifting the Slider slider.
The distance between the lenses (highlighted in red in the table in Fig. 25) was changed manually by the Slider slider until the rays at the output of the second (ideal lens) were set parallel to the main axis (in this position, the focal lengths of the telescope lenses are at the same point). The action of the slider in real time is displayed by the displacement of the elements of the optical scheme and the change of the ray paths in the optical diagram of the Layout window. The slider can be opened through the main menu Zemax> Tools> Miscellaneous> Slider.
If at the telescope output we put an additional paraxial collecting lens (element N6 in the table and the red plane on the optical scheme of Fig. 26), then we can see the distortion introduced by the real lens (see part of the Zemax diagrams in Fig. 26).

Fig. 26. Optical scheme and diagrams of distortions introduced by a real lens.
Literature
1. Site Optics Realm. Video tutorials on designing in the environment Zemax and the theory of optics. www.opticsrealm.com
2. Zemax Help> Optical Design Program User's Guide .pdf
3. H & L ELECTRICAL MANUFACTORY LIMITED hnl.en.e-cantonfair.com/products/sunny-brand-p13n05b-imx214-sony-sensor-13-0m-pixel-mipi-csi-1080p-sunny-cmos-camera-module- 552104.html
4. Edmund Optics. www.edmundoptics.com/optics/optical-lenses
5. Dr. Bob Davidov. Computer control technologies in the technical systems portalnp.ru/author/bobdavidov .
Introduction
More and more modern automation systems are equipped with optical devices for solving problems of positioning, recognition, observation, etc. Building ideal optical systems using the Zemax calculation program can be useful to non-professionals, for example, to better understand the theory, features of optical devices and perform approximate calculations of optical systems . This paper discusses techniques for building perfect optics in the Zemax environment, gives examples of calculating the autofocus range of a camera, constructing an equivalent circuit for an MGT 2.5x17.5 monocular, a SUNNY P13N05B lens of a Huawei P7 smartphone, and replacing ideal optical elements with a real one.
Perfect optics
The image in perfect optics, in which there are no distortions, is built according to the laws of paraxial optics. The term paraxial means "near the axis." Paraxial optics are well described by linear expressions, which at small angles are replaced by linear equations. In the paraxial region, any real system behaves like an ideal.
Calculations of ideal lenses in the Zemax environment are performed with the assumption that the lenses have paraxial properties not only near the axis, but also on the entire working surface, which acts as an ideal thin lens with a single refractive index of air.
Paraxial optics should be used as a reference with which the aberrations (distortions) of real optics are compared.
Care should be taken to transfer the results of calculations of paraxial optics to real systems, especially when building systems where properties near the optical axis and at a distance differ significantly.
A number of techniques for reducing aberrations and overall dimensions of lenses have been developed: the use of non-spherical surfaces, composite lenses, heterogeneous optical materials, etc. But how would a real lens (Petzval, Gauss, Barlow, ...) not be arranged its characteristics can only approach characteristics of an ideal lens.
')
Build image by collecting lens
Consider the case when rays from each point of the object plane diverge in all directions as from point sources. From the extreme point of object A, as shown in Fig. 1. Only the rays that are focused by the lens will hit the corresponding point B on the image plane. The number of rays of the object falling into the image plane is proportional to the diameter of the lens. The more rays from the subject falls into the image plane, the higher the brightness of the image.
Fig. 1. Conjugate points. The path of the rays from the point of the object to the corresponding point
images on the plane of the photodetector.
To minimize the computation of finding the image, consider the course of only a few rays, for example, as in Fig. 2: beam from the object along the optical axis; the beam passing through the center of the lens and the beam parallel to the optical axis, refracted by the lens and passing through the main focus of the lens (point F on the optical axis).
Fig. 2. Minimum constructions for finding the distance to the image plane, image size and lens magnification. For paraxial optics, the longitudinal increase (due to distances) is equal to the square of the linear increase (perpendicular to the axis), and the angular increase is inversely proportional to the linear.
Communication distance to the subject and image. Depth of field
The construction of the relationship between the focusing zone of the lens and the depth of field in the space of objects [1] is shown in Fig. 3. When the distance to the object is infinite, the plane of the focused image passes through the main focus (the displacement of the image plane relative to the focus is zero). The minimum depth of field in the space of objects is achieved at the maximum distance of the image plane (in the focus area) relative to the main focus.
Fig. 3. The relationship between the focus area of the lens and the depth of field in the space of objects.
Zemax design environment features
The Zemax environment functions most commonly used in the design of optical systems are assigned to individual buttons on the main menu. The purpose of these buttons is shown in Fig. four.
Fig. 4. Zemax program interface.
The types of surfaces of elements of optical systems, the radii of surfaces, the distances between elements and other parameters are entered into an editor table, in which each row contains the parameters of one element. The connection of the parameters of the table and elements of the optical scheme is shown on the example five.
Fig. 5. The connection of the optical scheme with the parameters of the table.
The perfect lens in Zemax
To simulate a paraxial lens in Zemax, you need to set the focal length and, if necessary, enable the calculation of the difference of optical paths passing through the lens (set the OPD mode status to 1 in the corresponding row of the editor table). By default, OPD calculation is not performed (OPD status is zero [2]).
We construct an ideal lens in Zemax, for example, with an entrance pupil diameter of 10 mm and a focal length of 15 mm, which collects the parallel rays of a distant object at one point.
1. Open a new table: menu> button
Fig. 6. The initial state of the table of the optical scheme of the Zemax editor. The rows of the table (NN 0; 1 and 2) contain the parameters of the object OBJ, the aperture stop STO and the image IMA.
2. Add a surface between the aperture and the image: select the last line in the IMA line> Lens Data Editor> Edit> Insert Surface menu
Fig. 7. Added standard surface N2.
3. Choose “Paraxial” surface type: line N2> Surf column: Type> properties window - Properties> Surface Type> Paraxial
Fig. 8. The N2 surface is changed to an ideal (Paraxial) lens with a focal length of 100 mm. The distance between the lens and the image is zero. The distance between the lens and the STO diaphragm is also zero.
4. Change the focal length from 100 (default) to 15 mm in the column of the Focal Length table
5. Set the distance 15 mm from the lens to the image in the Thickness column.
Fig. 9. The focal length of the lens is changed to 15 mm. The distance between the lens and the image is increased to 15 mm.
6. Let's set the diameter of the entrance pupil 10 mm: Main menu> button> tab Aperture> Aperture Value> 10
Fig. 10. The diameter of the input aperture of the optical scheme is specified: 10 mm.
7. Let's build the optical scheme: Main menu> button
Fig. 11. Optical layout in the Layout window. The coordinates of the aperture and the lens are the same (the distance between them is zero). The coordinates of the mouse on the diagram (in the scale of the optical scheme) are displayed in the picture header.
8. The Layout diagram does not show the rays to the left of the ideal lens (highlighted in red) coming from an object located at an infinite distance, which is designated as Infinity in the Thickness column of the OBJ zero line of the table. To show a part of these rays at the lens entrance, we introduce a surface at a distance, for example, 7 mm in front of the aperture stop STO.
Fig. 12. Added surface in front of the aperture stop STO.
9. Add surface 1 to the displayed part of the optical scheme and increase the number of rays to 7 for clarity: menu of the drawing Layout> Setting> First Surface = 1> Number of Rays = 7.
Fig. 13. Shows the rays on a segment of 7 mm to the aperture. Increased the number of rays from 3 to 7.
10. Make the first surface invisible: row N1 of the table> Surf column: Type> properties window - Properties> Draw tab>
11. Update the Layout window of the optical scheme via the main menu button or double-clicking in the area of the scheme window.
Fig. 14. The first surface of the optical circuit is made invisible.
In the Layout window, you can track changes in the tabular parameters of the optical system and the parameters of the main menu, shown in Fig. 4 and Fig. five.
Smartphone Camera Lens Model
To build an ideal model, take the composite lens of the SUNNY P13N05B camera of the Huawei P7 smartphone (Fig. 15). The lens of the smartphone consists of five plastic elements. An example of a composite lens is shown in Fig. sixteen.
Fig. 15. Dimensions [3] and photographs of the SUNNY P13N05B camera with a SONY IMX214 13 MP photodiode array. 1. - camera module with photodiode array; 2- camera lens; 3 - autofocus drive coil - moving the lens relative to the sensor matrix.
The camera P13N05B has the following characteristics.
• Lens size: 1/3 "
• Size of photodiode array: 6.1 mm (H) × 4.5 mm (V)
• Diagonal core: 5.9 mm
• Composition of the lens: 5 plastic elements (see. Fig. 16)
• Focal length: 3.79 mm
• Aperture number (f / #): 2
• Field of view: 75 ° ± 3 °
• Depth of field: from 7 cm to ∞
• Autofocus Drive Range: ≥ 0.24mm
Fig. 16. An example of a composite lens. IPhone 6 lens.
The parameters of the optical scheme of an ideal camera lens (see Fig. 17) are specified in the Lens Data Editor table and in the windows of the Zemax main menu buttons. The function selected from the list of functions of the selected cell in the Thickness column of the table automatically sets the best distance between the lens and the image. To construct the best image of an object distant to an infinite distance, the plane of the photoreceiver must pass through the point of the main focus at a distance of 3.79 mm from the lens.
Fig. 17. Optical scheme of a paraxial lens of a photographic lens. Item removed to infinite distance.
Moving the object to the lens by 10 mm while maintaining the viewing angle of 76 ° / 2 in the Field Data window (Fig. 18) increased the distance between the lens and the image to 6.10 mm. Therefore, the change in autofocus when an object approaches from infinity to 10 mm is 2.31 mm (like 6.10 mm - 3.79 mm).
Fig. 18. Building rays from an object located 10 mm from the paraxial lens of the camera and finding the position of the autofocus.
In the specification of the camera P13N05B indicated that the depth of field in the space of objects is in the range from 7 cm to ∞ (infinity). Set the object at a minimum distance of 70 mm from the lens aperture. Zemax sets the distance between the lens and the image plane of 4 mm (see the selected cell of the table in Fig. 19). Thus, to build a high-quality image of an object located in the zone from 7 cm to ∞, it is required to change the distance between the lens and the photosensor from 4 to 3.79 mm. The required 0.21mm change is covered by a 0.24 mm camera autofocus range.
Fig. 19. The distance to the image is 4 mm when the distance to the object is 70 mm. The focal length of the lens is 3.79 mm.
Dependence of the focus range on the focal length of the lens
Focusing area depends not only on the distance to the subject, but also on the main focus of the lens (lens). In Fig. 20 shows the geometry of finding focus areas for lenses with the main focus F1 = 7.5 mm and F2 = 19 mm and the positions of the object in the range AB = 35 ... 52 mm. To adjust the sharpness with the lens F1, you need to change the distance between the main focus of the lens and the image plane in the 0.8 mm range, whereas for the lens with F2, this range increased to 12 mm.
Fig. 20. An example of building focus areas for lenses with different focal lengths F1 and F2.
Ideal telescopes
The comparative dimensions of the Kepler and Galileo telescopes for the same magnification F1 / F2 are shown in Fig. 21. Kepler telescope with collecting lenses gives an inverted image. Galileo's more compact telescope includes a diffusing lens and gives a direct image.
Fig. 21. The scheme of Kepler telescopes (a) and Galileo (b) with the same magnification F2 / F1.
The miniature monocular of MGT 2.5x17.5 USSR, LZOS (Lytkarinsky optical glass factory) is assembled according to the Galilean scheme (Fig. 22). It has the following characteristics.
• Increase: 2.5 times (times)
• Lens diameter: 17.5 mm
• Field of view: 13.5 degrees
• Resolution: 15 ang. sec
• Eyepiece focusing limit: -5 ... + 5 diopters
• Overall dimensions: 22 x 38 mm
Fig. 22. Type and approximate dimensions of a miniature MGT 2.5x17.5 monocular. Item is on the right.
The equivalent ideal optical scheme of the MGT 2.5x17.5 monocular in ZEMAX is shown in Fig. 23. The scheme consists of a collecting and diffusing lens with the main foci of 37.5 mm and -15 mm, respectively, having a ratio of 2.5 times. Diameter of the collecting lens is 28,75 mm.
Fig. 23. Tabular data and an ideal optical scheme of a monocular MGT 2.5x17.5. Parallel rays go from the object to an infinite distance.
The option to replace the real paraxial lens
Replace the first paraxial lens (diameter: 17.5 mm; focal length: 37.5 mm) of the monocular with an achromatic lens from the Edmund Optics catalog [4]. To minimize the selection of lenses set the following conditions: category - Achromatic Lenses; diameter - 18 mm; effective focal length EFL 30-39.99 mm; wavelength range - 425 - 675 nm.
Nearest lens required: 18mm Dia. x 35mm FL, VIS 0 ° Coated, Achromatic Lens, Stock No. # 47-706 (part number).
To build an achromatic lens in Zemax, from its specification we take the parameters listed in Table 1. Parameters can also be found in the PDF drawing drawing of the Edmund Optics website [4] or in Fig. 24
Table 1. Edmund Composite Achromatic Lens Parameters # 47-706
| Parameter | Value | Note |
|---|---|---|
| Diameter | 18.0 mm | Diameter |
| Clear Aperture CA | 17.0 mm | Diaphragm |
| Effective Focal Length | 35.0 mm | Effective focal length |
| Center Thickness CT 1 | 6.01 mm | The thickness of the 1st element along the axis |
| Center Thickness CT2 | 1.60 mm | The thickness of the 2nd element in the axis |
| Radius R1 (mm) | 24.26 mm | First surface radius |
| Radius R2 (mm) | 16.23 mm | Radius of the second surface |
| Radius R3 (mm) | -152.99 mm | Third surface radius |
| Substrate | N-BAF10 / N-SF10 | Element materials |
Fig. 24. Drawing of the Achromatic Edmund Lens # 47-706.
Replacing the parameters of the first lens of an ideal telescope (row N2 of the table in Fig. 23) with an Edmund lens # 47-706 gives the option shown in Fig. 25
Fig. 25. Option telescope optics with a real achromatic lens. The distance between the lenses highlighted in the table in red is found by manually shifting the Slider slider.
The distance between the lenses (highlighted in red in the table in Fig. 25) was changed manually by the Slider slider until the rays at the output of the second (ideal lens) were set parallel to the main axis (in this position, the focal lengths of the telescope lenses are at the same point). The action of the slider in real time is displayed by the displacement of the elements of the optical scheme and the change of the ray paths in the optical diagram of the Layout window. The slider can be opened through the main menu Zemax> Tools> Miscellaneous> Slider.
If at the telescope output we put an additional paraxial collecting lens (element N6 in the table and the red plane on the optical scheme of Fig. 26), then we can see the distortion introduced by the real lens (see part of the Zemax diagrams in Fig. 26).
Fig. 26. Optical scheme and diagrams of distortions introduced by a real lens.
Literature
1. Site Optics Realm. Video tutorials on designing in the environment Zemax and the theory of optics. www.opticsrealm.com
2. Zemax Help> Optical Design Program User's Guide .pdf
3. H & L ELECTRICAL MANUFACTORY LIMITED hnl.en.e-cantonfair.com/products/sunny-brand-p13n05b-imx214-sony-sensor-13-0m-pixel-mipi-csi-1080p-sunny-cmos-camera-module- 552104.html
4. Edmund Optics. www.edmundoptics.com/optics/optical-lenses
5. Dr. Bob Davidov. Computer control technologies in the technical systems portalnp.ru/author/bobdavidov .
Source: https://habr.com/ru/post/303714/
All Articles