A detailed look at the legacy of Leibniz

Translation of Stephen Wolfram's article " Dropping In on Gottfried Leibniz ".

For many years, I was interested in the personality of Gottfried Leibniz, in particular because he wanted to create something like Mathematica , Wolfram | Alpha and maybe even A New Kind of Science but three centuries earlier. Therefore, when in the recent past I visited Germany, I passionately wanted to visit its archives in Hanover .

Leafing through the yellowed but still strong sheets of his notes I felt some interconnection - I tried to imagine what he was thinking when he wrote them. I also tried to compare the contents of the records with what we know now - three centuries later.
')


Some things in mathematics do not change over time - for example, here Leibniz recorded decomposition in a row of a square root of two (the text itself is written in Latin):



Or here Leibniz tried to calculate the continued fraction - and although there is an arithmetic error here , he nevertheless brought the result to the end (P - in his early notation, he used an equal sign).



And here you can see some points from the mathematical analysis, which can be found practically in this form in modern textbooks.



But what was the essence of his main work? What did Leibniz ponder most of his time?

I have always considered Leibniz an ambiguous person. He did many seemingly unrelated things - in philosophy, mathematics, theology, in lawmaking, physics, history, and other fields. He described all his achievements in the strange manner of the 17th century that we see today.

But over time, I began to better understand Leibniz as a person and I discovered that almost everything that he did was aimed at achieving a single goal which, oddly enough, is very similar to the one to which I aspire today.

Gottfried Leibniz was born in Leipzig in 1646 (now it is a German city). He was born four years after the death of Galileo and the birth of Newton. His father was a professor of philosophy and his mother was from a family of booksellers. Leibniz’s father died when he was 6, but only two years after he was allowed to use his father’s library. Leibniz began to read everything in one gulp. At 15, he entered a local university, studied philosophy and law. At the age of 20 he received diplomas in both directions.

Already as a teenager, Leibniz was interested in systematizing and formalizing knowledge. At that time there were only vague ideas about the systematization of knowledge, for example, in the semi- mystic work Ars Magana (Latin great art) written by Raimund Llull ( Ramon Llull ) in the 1300s, you can try to find a description of a universal system in which all knowledge can be derived from combinations of signs belonging to (as Descartes (Descartes) called it) "the alphabet of human thought." In his graduate work in philosophy, Leibniz adhered to this idea. He used simple principles of combinatorial mathematics to calculate the number of possibilities, talked about the decomposition of ideas into simple components with which you can work using the “logic of invention”. And to evaluate his method, he included in the work a task whose solution was to prove the existence of God.

As Leibniz himself said later, these theses written at the age of 20 were mostly naive. But it seems to me that they have already characterized the mindset of Leibniz with whom he did not part throughout his life. For example, his thesis on the law of “perplexing court cases” was devoted to how these cases can be resolved using logic and combinatorics.

Leibniz was to become a professor, but instead he decided to start working as an advisor to various judicial and political figures. Quite often, he conducted research, examining the dark places of their ancestry and history. He also systematized their libraries, various legal codes, etc. It happened that he had to solve various engineering problems, for example, how best to protect silver mines from water. However, in the beginning most often it was just a consultation on political maneuvering.

Because of one such case in 1672, Leibniz had to leave for Paris for 4 years - during this time he communicated with many leading educators of the time. Prior to this, Leibniz’s knowledge in mathematics was only superficial. But in Paris, he studied all the latest ideas and methods. In particular, he tracked down Christian Huygens (Christiaan Huygens), who agreed to teach him mathematics after he managed to take a test on finding the sum of inverse triangular numbers .

For several years, Leibniz perfected his ideas about the systematization and formalization of knowledge, developing the architecture of what would make knowledge, in modern terms, calculated. He saw the first step in the development of ars characterisitca , a methodology that would allow some things to be associated with symbolic representations, thereby creating a homogeneous “alphabet of thoughts”. Then - as he believed - this alphabet will allow to find “truths in all spheres, using mathematics, be it arithmetic or algebra”.

He came up with many ambitious titles for his ideas: scientia generalis (“common method of knowledge”), lingua philosophica (“language of philosophy”), mathematique universelle (“universal mathematics”), characteristica universalis (“universal system”), and calculus ratiocinator (“mathematics thoughts "). He saw many applications of his ideas in almost all spheres of life - law, medicine, engineering, theology, etc. But he achieved the greatest success in mathematics, and it happened quite quickly.

In my opinion, the most remarkable is the fact that in the history of mathematics the question of notation was extremely rarely raised. This happened at the beginning of the development of modern mathematics at the end of the first decade of the 19th century, thanks to the work of people like Gottlob Frege and Giuseppe Peano. And in the modern world this happened thanks to my work on the creation of the Mathematica system and the Wolfram language. However, a similar event also happened more than three centuries ago thanks to Leibniz. And it seems to me that Leibniz’s successes in mathematics are largely due to how hard he worked with the problem of notation, with what clarity he talked about mathematical structures and related concepts.

If you look at his notes, you will see in an explicit form the process of developing notation. Many things look modern, but they are not devoid of the charm of the 17th century, for example, using alchemy or astronomy symbols to designate algebraic variables.



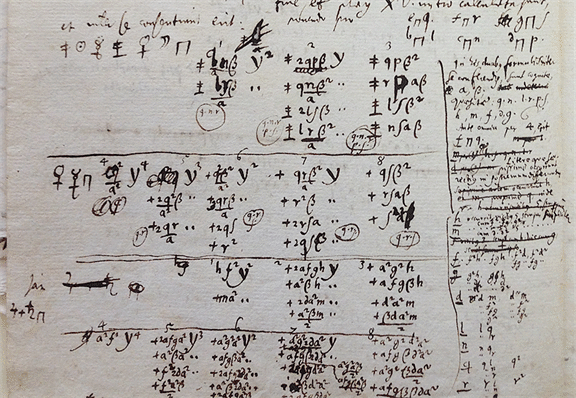
Here, the symbol P is used instead of the equal sign. Leibniz had an interesting idea that when the legs of P are equal - this is equality, when, for example, the left leg is longer, it is equivalent to the “>” sign and vice versa.



Dashes above parts of expressions are used for grouping - I like the idea more than brackets, although it would be harder to type them from the keyboard.



We use such lines for square roots, but Leibniz wanted to use them also in integrals with the “d with a tail” symbol that reminds me of one of the symbols in Mathematica that we created to represent integrals to the system.



In particular, when solving equations, the “±” symbol is quite common and therefore there is often confusion about how to group variables in an expression, say, a ± b ± c. It seems Leibniz also considered this a very inconvenient obstacle and he presented a notation which would be useful to use today.



On account of some notation, I do not have unambiguous thoughts, although many of them, such as tildes on top , look quite nice.



As well as these elements with dots:



Or something like diagrams here ...



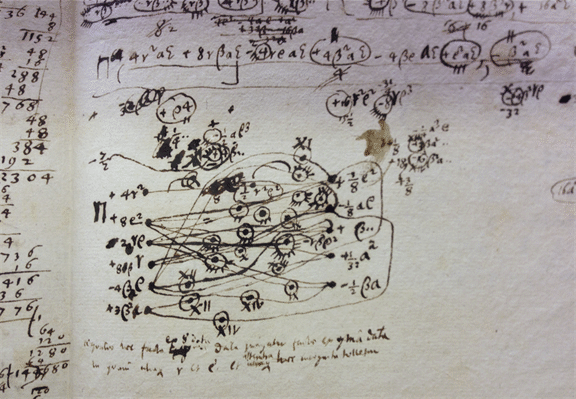
Of course, the most famous elements of the Leibniz notation are the integral sign (long s) and d (in the fields to the right). They are presented together for the first time on November 11, 1675, then 5 in record 1675 was replaced by 3, probably by Leibniz himself.



It seemed to me interesting that all its designations for various operators are very different from logical operators. The operator "or" is just a Latin translation of vel, "and" - et, and so on. And when he came up with quantifiers (universality and existence), he designated them as acronyms in Latin UA and PA



It has always amazed me that the idea of ​​creating a universal language for operating with any data appeared only in the thirties of the twentieth century. Therefore, I wondered if the first sketches of such a system could be hidden in the works of Leibniz - take at least the diagram we just looked at, which we can now call some sort of Turing machine. But over time, having studied more information about Leibniz himself, I realized that all the same this is not the case.

One of the main proofs of my assumption is that Leibniz did not seriously consider discrete systems. He called the results of combinatorial calculations "obvious and not requiring proof" presumably because, he believed, they can be confirmed using simple arithmetic. And he developed the mathematical apparatus only for "geometric" or continuous mathematics. When describing the properties of curves, Leibniz even then proposed an analogue of continuous functions, but it never occurred to him to apply the same principle to discrete mathematics, which could, for example, lead him to the idea of ​​universal elements for constructing functions.

Leibniz understood the full significance of the apparatus developed by him of infinitesimally small values ​​and wanted to develop similar “calculus” for other fields of knowledge. Another idea close to a unified knowledge system was the coding of logical properties. He wanted to assign a prime number to every possible property, and then each item could be characterized by the product of these prime numbers. Such an approach would allow to show the logical difference between objects using arithmetic operations. But he considered only static properties and he never had the idea of ​​a similar Gödel numbering (Gödel), where the numbers also coded operations.

But even despite the fact that Leibniz did not come to the idea of ​​a universal knowledge management system, he still understood that computing is primarily a mechanical process. And rather early in his life, he decided to build a real mechanical calculator for arithmetic operations. This is partly due to the fact that Leibniz himself needed such a device (the best motivation for this kind of things). Despite his mastery of algebra and the like, his works are full of adorable calculations in the field column (sometimes with errors) which are reserved for posterity.



There were separate examples of mechanical calculators created in the time of Leibniz, and when he was in Paris, he undoubtedly saw the folding calculator created by Blaise Pascal in 1642. But Leibniz wanted to make a universal calculator that could perform four basic arithmetic operations. He also wanted the calculator to be easy to use, for example, turning the handle in one direction would mean multiplication, and in the other - division.

In the works of Leibniz, there are many drawings and diagrams of this machine:



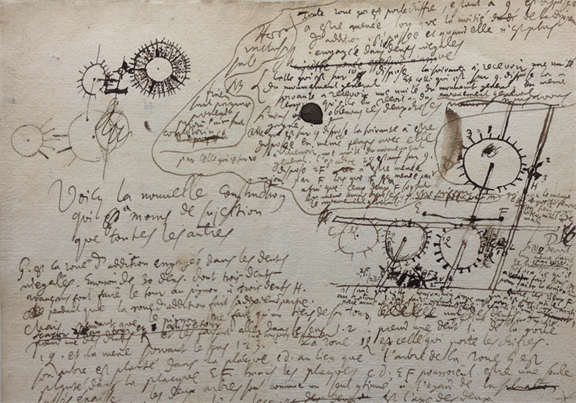
He imagined that his calculator would bring great practical benefits and a considerable part of his fantasies consisted of ideas for successful development of the business associated with the calculator. But unfortunately Leibniz failed to make the calculator reliable - he did not always give the correct result. Like most of the similar mechanisms of that time, the calculator was just an embellished path (odometer). Leibniz faced the same problem as Charles Babbage two hundred years later - it is extremely difficult to make all the disks move at the same time when necessary.

In the beginning, Leibniz created a wooden prototype which he hoped could work with three-digit or four-digit numbers. But in the preliminary screenings in particular, in front of Robert Hook (Robert Hooke) in London in 1673, the mechanism let him down. However, he continued to reflect on its improvement - in 1679, the work "Last improvements to the arithmetic machine" was published:



Notes from 1682 suggest that Leibniz faced new problems:



But he had drawings and he ordered from engineers the production of a copper version of the mechanism for working with a large number of numbers:



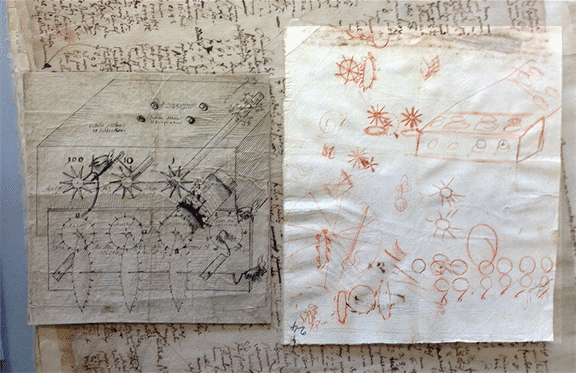
In particular, Leibniz even prepared “marketing material” for his car:



... and user manual ...



Here is the complete machine model with instructions for use:



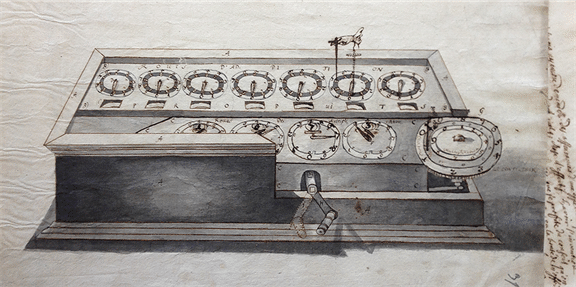
But despite all his efforts, the calculator still did not work. Leibniz worked on a calculator for over forty years and spent a total of over a million dollars on today's exchange rate.

When I visited his archive - I could not help asking curators about what happened to this calculator. “Hm, we can show you to him,” was the answer. The calculator was on one of the many shelves in the basement and looked completely new in its glass case - my own photo in which past and present are strangely interwoven:



Calculator has not lost a single detail. He was in a convenient portable wooden case with a handle for arithmetic operations. If it worked correctly, in just a few minutes of rotation of this pen, we could get an answer to any question from the section of simple arithmetic.



Leibniz considered his calculator a practical project, but nevertheless he also wanted to use it in order to create a “logic” describing mechanical connections. He also reflected on the nature of numbers and arithmetic. He was also struck by binary numbers.

Grounds other than 10 have been used in various entertaining mathematical examples for centuries, but Leibniz focused on base 2 because he believed that it was of particular importance and was probably a bridge between philosophy, theology and mathematics. And when he learned about I Chang from the missionaries from China, this only strengthened his faith. He viewed this work as related to its characteristica universalis .

Leibniz came to the conclusion that it is possible to build a calculator for binary numbers, but at the same time he believed that only numbers with a base of 10 are of practical importance.

Today Leibniz’s binary numbers have mixed feelings. One part of the works looks quite modern while the other is clearly from the 17th century. For example, he argued that the binary system serves as proof that everything can be obtained from nothing, that 1 is God, and 0 is nothing.

After Leibniz for a couple of centuries, the binary number system did not develop until the recent growth associated with the advent of computers. Therefore, the calculations of Leibniz concerning the binary system can be safely called the leading time:



Working with binary numbers, Leibniz was looking for the easiest way to describe the structure of things. And when he introduced the concept of "monad", he no doubt sought the same thing. I must say, I can never understand monads. When it seemed to me that I understood everything there was some nuance that destroyed the whole integrity of the picture.

I have always found it teasing that Leibniz came to the conclusion that the best of all possible worlds is a world in which a huge variety of phenomena follows from the minimum possible number of prerequisites. And indeed, at the time when I was just beginning to work on NKS when I formulated and then studied one-dimensional cellular automata in 1981, I wanted to call them “polymons”, but at the last second I became afraid because I got confused in monads again.

There was always some mystery around Leibniz and his works. Kurt Gödel (possibly in a fit of paranoia) was convinced that Leibniz revealed a great truth which was then hidden from society for centuries. But despite the fact that the works of Leibniz were seized after his death, this was due to the fact that the works on history and genealogy contained information not subject to publicity, whether it be state or personal secrets of those in power.

The works of Leibniz were published a long time ago, but still not fully understood. And the problem is not that there are too many of them. It is about 200,000 pages, which fill no more than a dozen bookshelves. This is only slightly more than my personal archive, which I have been conducting since the 1980s. Difficult causes the heterogeneity of the material - not only different areas of knowledge, but also drawings created one on top of another, notes and letters, the relationship between which is not fully understood.

The Leibniz documents archive contains as very large documents:



and very small (with age, Leibniz’s handwriting became smaller and more dense):



Most of the documents in the archive look very seriously and scientifically. However, despite the fact that the paper at the time of Leibniz was quite expensive - he did not hesitate to spend it on funny drawings (perhaps Spinoza?).



— . 300 , , (Jacob Bernoulli):



? , , . , .



. , . , . , .

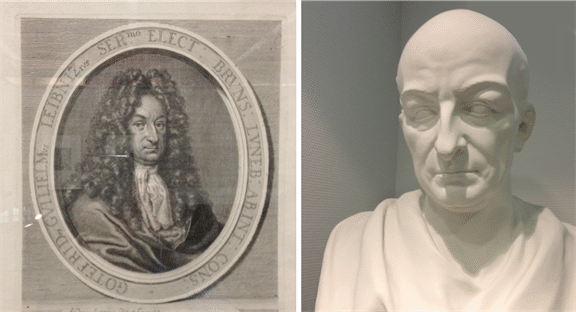
, , . , (George I) , . 30 . , .

— :



— Gottfried von Leibniz von. , . omnibus ex nihilo ducendis ; sufficit unum (« ; 1»). .

2007 60- (Greg Chaitin) . . .

, , :



. 70 , . — « ».



, , «»:



? . — .

, , . . , (, 1 0) . , , . .

. . , . , , . .

, , «» . , , , . .

, . — 300 — .

. , Principia .

— — , . , NKS , , , .

, , , , . — . — , , .

, , . . , . , , .

— , ? , . — . . ( Principle of Computational Equivalence ). , . . , , . .

- . , Wolfram Mathematica Wolfram|Alpha.

14 1716 . 2016 300 . 300 , .