In the world of mathematical paradoxes

Good day, dear habrasoobschestvo.

Today I would like to touch upon such an exciting topic as mathematical paradoxes. On this topic on Habré several remarkable articles ( 1 , 2 , 3 , 4 , 5 ) have already been published, but in math the interesting paradoxes of this sample are far from being exhausted.

Therefore, we will try to consider other entertaining paradoxes (and some “not quite” paradoxes), which have not yet received proper lighting here.

Heap paradox and the “Bald” paradox

These paradoxes have been known since antiquity. To begin with, we will formulate and consider the heap paradox associated with the uncertainty of the heap concept:

“If one is added to a grain by grain, then at what point does the heap form?”
or reverse wording:
“Removing one grain from a heap of 1 million grains, from what moment does it cease to be a bunch?”
')
The paradox formulation is based on an obvious premise, according to which one grain does not form a heap, and an inductive premise, according to which the addition of one grain to an aggregate that is not a heap is not essential for the formation of a heap. From these presuppositions it follows that no aggregate of an arbitrarily large number of grains will form heaps, which contradicts the idea of ​​the existence of a heap of grains. Obviously, these arguments lead to wrong conclusions.

However, until very recently, it was not clear what arguments to use here. Only with the advent of the Lofty Zadeh theory of fuzzy sets and fuzzy logic, it became clear that fuzzy reasoning is appropriate here, since there is a classic fuzzy logic object - the vague notion of “being a bunch”. These objects in fuzzy logic are interpreted as having an inaccurate value, characterized by some fuzzy set.

According to such reasoning, the conclusion at each step remains the same, but the belonging of its correctness decreases with each step. When this affiliation falls below 50%, the opposite conclusion becomes more correct.

Similar reasoning can be applied to the “Bald” paradox:
“If the hair from the head falls out one by one, when does a person become bald?”

Paradox liar

If the statement in the picture is true, then, based on its content, it is true that it is false; but if it is false, then what it claims is wrong; it means that it is not true that the statement in the picture is false, and it means that this statement is true.

The liar paradox demonstrates the divergence of colloquial speech with formal logic, introducing a statement that is both true and false. Within the framework of formal logic, this statement is not provable and irrefutable, therefore the solution of this paradox does not exist, but there are various options for its elimination.

For this you can apply the reasoning used in the previous section, for this we suppose that the statement is true by 0.5, then it is false by 0.5, that is, not every phrase can be called entirely false or entirely true - “in some way the statement in the picture is lying, but in something - telling the truth "

The same conclusion can be reached with the help of triple logic. There are three degrees of truth in it: "truth", "lie" and "indefinitely." By "indefinitely" is meant an intermediate meaning between truth and falsehood. To this degree of truth and the paradox of the liar.

As already mentioned, this is not a solution to the liar paradox, but merely an explanation of why this paradox arises in the classical two-digit logic of statements. They testify that the strict division of all statements into true and false in this case is not applicable, since it leads to a paradox.

At present, many people are of the view that this statement is not a logical statement at all, and it is senseless to apply classical methods of formal logic to it.

Theseus Paradox

This paradox can be formulated as follows:

“If all the component parts of the original object were replaced, does the object remain the same object?”

It was proposed several solutions to this paradox. According to the philosophical school of Aristotle, there are several reasons for describing an object: the form, material and essence of a thing (which, according to Aristotle, is the most important characteristic). Based on this, the ship remained the same, since its essence has not changed, only the worn material has changed.

The next solution proposed to give the argument the “same” quantitative and qualitative characteristic. In this case, after changing the boards, the Theseus ship will be quantitatively the same, and, qualitatively, by another ship.

Recently, in order to solve the Theseus paradox, they suggested using a 4-dimensional interpretation, in which a 3-dimensional ship also has a length in 4 dimensions-time. The resulting 4-dimensional ship over the time series is quantitatively identical with itself. But individual “time slices” may differ qualitatively from each other.

Abilene's Paradox

This paradox is that a group of people can make a decision that contradicts the possible choice of any member of the group due to the fact that each individual believes that his goals are contrary to the goals of the group, and therefore does not object.

The paradox was described by Jerry Harvey in the article The Abilene Paradox and other Meditations on Management. The name of the paradox is based on the following anecdote described in this article:

In one hot Texas evening, a certain family played dominoes on the porch until the father-in-law offered to go to Abilene for dinner. My wife said, "It sounds good." The husband, despite the fact that the trip promised to be long and hot, thought that it would be necessary to adjust to others, and said: “In my opinion, not bad; I hope that your mother will not refuse. " The mother-in-law replied: “Of course, let's go! I have not been to Abilene for a long time. ”
The road was hot, dusty and long. When they finally arrived at the cafeteria, the food was tasteless. Four hours later, they, exhausted, returned home.

One of them said insincerely: “True, it was a good trip?” My mother-in-law said that, in fact, she would have better stayed at home, but she went, since the other three were full of enthusiasm. The husband said: "I would be glad not to go anywhere, I went only to give pleasure to the others." The wife said: “And I went, counting on the joy of the others. You had to be crazy to go voluntarily on this trip. ” The father-in-law replied that he offered this only because it seemed to him that the others were bored.

And they sat, stunned by the fact that they had gone on a trip that none of them wanted. Each of them would prefer to quietly enjoy that day.

This paradox is easily explained by various sociological sciences, confirming that a person rarely commits acts contrary to the actions of his group. I think many people have come across this paradox more than once in their lives.

The Simpson Paradox and the Will Rogers Phenomenon

I note that these paradoxes are “seeming”, that is, they can arise at an intuitive level, but if you carry out calculations, then it is easy to make sure that no paradox arises.

To illustrate Simpson's paradox, consider the example described by the well-known popularizer of mathematics, Martin Gardner .

Suppose we have four sets of stones. The probability of pulling out the black stone of set No. 1 is higher than that of set No. 2. In turn, the probability of pulling out the black stone from set No. 3 is greater than that of set No. 4. Combine set No. 1 with set No. 3 (we get set I), and set number 2 - with a set number 4 (set II). Intuitively, one can expect that the probability of pulling out a black stone from set I will be higher than from set II. However, in general, this statement is false.

An example in which the simpson paradox is performed:

	Black balls	White balls	Chance to pull a black stone
Set №1	6	7	6/13 ≈ 0.4615
Set number 2	four	five	4/9 ≈ 0.4444
Set number 3	6	3	6/9 ≈ 0.6667
Set №4	9	five	9/14 ≈ 0.6429

Now let's mix sets No. 1 and No. 3 - of which black stones can be pulled out with greater probability and sets No. 2 and No. 4 - of which black stones can be pulled out with less probability.

	Black balls	White balls	Chance to pull a black stone
Set I	12	ten	12/22 ≈ 0.5454
Set II	13	ten	13/23 ≈ 0.5652

As we see from the table after mixing, the probability of pulling out a black stone from set II was higher than from set I.

Mathematically, there is no paradox here, since the total probability of a set depends on the ratio of the number of black stones and both colors, in this case there were 9 black stones in the 4 set, and 7 white stones in the first set that most affected the final alignment.

Close to the Simpson Paradox and the Will Rogers phenomenon. In fact, they describe the same phenomenon, but in other terms.
I think many times faced with phrases like this:
"When the Oceans left Oklahoma and moved to California, they increased the average intelligence of both states"

This phrase is attributed to Will Rogers, in honor of which the phenomenon got its name.

From the point of view of mathematics, there is no paradox either. To verify this, it is enough to consider two sets: the first is {1, 2}, and the second is {90,100}, if the number 90 from the second set is transferred to the first, then the arithmetic average of the elements of both the first set and the second will increase.

Cell disappearance

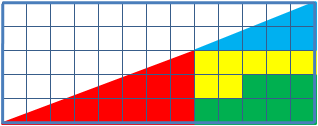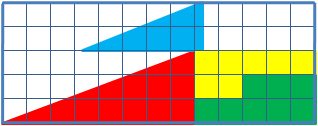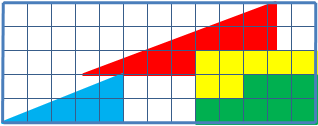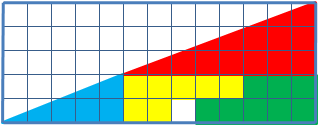
A wide class of tasks for the permutation of figures with signs of sophistry: initially a masked error was introduced in their condition. To some extent, these tasks are closer to optical illusions than to mathematics.

For example, let's consider one similar problem: a right-angled triangle of 13 × 5 cells is given, made up of 4 parts. After the parts are rearranged, when the original proportions are visually preserved, an additional cell, not occupied by any part, appears.

Mathematically, the paradoxes and the mysterious disappearance of the square are not here. Visually observed triangles, in fact, they are not, the hypotenuse in both pseudo-triangles are in fact broken lines (in the first triangle it has a break inwards, and in the second - outwards). If we impose a triangle on each other, then between their "hypotenuse" parallelogram is formed, which contains the "missing" area.

Instead of conclusion

To my great regret, it is impossible to consider all interesting mathematical paradoxes (and “not quite” paradoxes) in the framework of one article. But I hope that this article did not leave you indifferent, and I will be very happy if you decide that you have not wasted your time reading.