Spiral Ulam, the area of ​​the prohibition of primes

Each natural number has very many known and, apparently, even more unknown properties. Even - odd, simple - composite, finite - infinite and other properties contribute to the introduction of the classification of numbers of some order in their set. The traditional approach assumes that without having the number itself (its value), it is impossible to determine its properties. But it is not so. A number of useful properties for some numbers can be determined without knowing their values, but having information about their position in the natural number of numbers (NPS). Primes, except 2, can only be odd with a flexion of ≠ 5, and their position of the NPS is determined by an odd position. These positions themselves are not all equivalent. About some large odd N (x1, x2) numbers (of course in odd positions in the NPS) you can, without using the traditional (probabilistic) and deterministic (very laborious) algorithms, definitely, they cannot be simple.

The device NRS, the position of the numbers in the NRC and their properties

We will consider the relationship of the properties of natural numbers and their position in the NPS, considering such a relationship to exist.
For example, an odd number equal to an even square (> 2) without one, always composite: x² -1 = (x +1) (x -1). Odd square is a composite number. Information about this in a convenient form is provided by the NPS model - the Ulam spiral, where the position of all squares is uniquely determined. Consequently, the described diagonal rays pressed to the diagonals of squares cannot contain primes. In addition to the lines mentioned below, we point out others that have the same property. This property of the model was not previously mentioned by Ulam himself or by other authors who worked with the model or mentioned it in the publications, was not noted. Big riddle in this. We identify the point (cell) with the coordinates (x1, x2) of the helix plane and the number N (x1, x2) in this position.
The fact of the existence of a forbidden area for primes in the NPS is established from observations of a spiral, and then confirmed (proved) by the author by mathematical means. The usefulness of this property is not obvious. But for odd numbers, the position of the cells (x1, x2) of which in the spiral belongs to the area of ​​the prohibition of prime numbers, not only their simplicity is established, but they are also factorized without problems.
On the basis of this fact about some odd numbers N (x1, x2) of even a very large capacity, it is possible to state with certainty that they are composite and then factor them easily.
')
The coordinate system of the model . When using the concept of the contour structure of the NPS in the previous work “The Model of the Natural Number Series (NPS). Spiral Ulam ”the author proposed a coordinate system on the plane of the spiral. The center of the spiral is taken as the coordinate origin. The system is rather closer to polar than to Cartesian. The first coordinate x1 of the number N (x1, x2) is assigned to the contour number (x1 = k) . The second coordinate x2 <L (k), where L (k) is the length of the contour, determines the distance of the cell with the number N (x1, x2) from the beginning of the contour. For the convenience of the reader, the author has saved the drawings of the previous work in this.


Figure 1 - Model NPS and an enlarged fragment of the central part of the helix
In Figure 1, from the NPG fragment (399x399 cells), the central part with the number of cells 19x19 = 361 is cut out and the enlarged fragment is shown on the right with the cells (x1, x2) filled with numbers. In the figure (with numbers), even in a limited volume, the vertical and horizontal single and double lanes (highways) that do not contain shaded cells, i.e., are clearly visible. prime numbers. Below in Figure 2, a careful examination of these highways can also be seen.

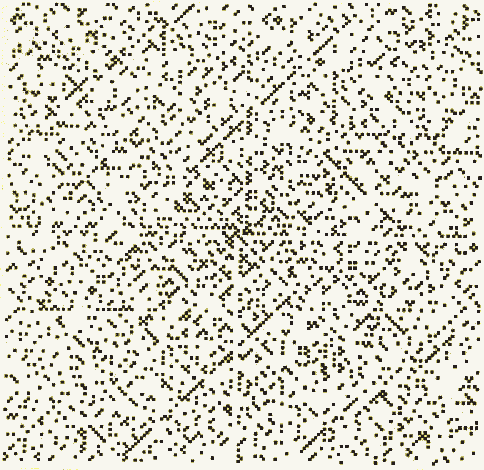
Figure 2 - Representation by the model of a helix of a fragment (200x200 cells) of the natural number with filled cells for prime numbers.
When manipulating with primes it is useful to know and have information about some of their properties and dependencies. For example, the fact that squares of prime numbers, for example p and q numbers, except 2, when comparing modulo 8, always have one residue 1, ² 1 (mod8) , and modulo 30 such residues can be only two 1 and 19: ² 1 (mod30) or q² ≡ 19 (mod30). Let us give more detailed information about the number 30, which clarify something about such mysteriousness in the behavior of prime numbers, their full squares. This number 30 = 2 ∙ 3 ​​∙ 5 plays a significant role in the study of simple natural numbers.
The number 30 is the largest number for which all are mutually simple with it and its smaller numbers are prime. Number 30 is preceded by the same number 24, which also plays a significant role in number theory.

The connection of two models of the natural series of numbers of the flat Ulam spiral and linear analytical

It’s more common for us to perceive natural numbers as elements of a linear model. This is convenient, since on the numerical axis all points have one single coordinate, moreover, the value of this coordinate coincides with the number itself. When manipulating data, you don’t need to think about what is being processed - the coordinate or the number itself. Not so on the flat. Its point has three characteristics: two coordinates (x1, x2) and the value of the number N (x1, x2) at a point, which in our model is a function of coordinates. Therefore, we establish some connections between the two models of a linear and flat helix. The concept of contour is introduced in both models in the same way - the beginning of the contour is an odd square. The length of the contour is a multiple of 8. The numbers forming the contour are a segment of the natural series.

Natural numbers can be represented by such an analytical model:
30k, 30k ± 1, 30k ± 2, 30k ± 3, ..., 30k ± 15, k = 1 (1) ∞, of which primes are only represented as 30k ± 1, 30k ± 7, 30k ± 11 , 30k ± 13 . Of these, 8 prime numbers with k = 0.1 are 7,11,13,17,19,23,29 and 31 ≡ 1 (mod30) form a multiplicative group of residues modulo 30. The order of such a group is equal to the value of the Euler function 30 (30 ) = (2) ((3) (5) = 8. Such groups are called Euler groups.
Now about the number 24. Let us show that for primes p, q> 3, the difference of the squares of two prime p² - q² is always divisible by 24. Consider two triples of adjacent numbers (p-1) p (p + 1) and three (q-1 ) q (q + 1), where p and q are simple. In each triple one of the numbers is a multiple of three, and this is not p or q. Consequently, both the products of (p –1) (p + 1) and (q –1) (q + 1) are multiples of three, which are formed by pairs of consecutive even numbers. But for such numbers it is known that one of them is a multiple of two, and the other is four. It follows that both works are divided by 8. But they are divided into three. Then each of the products is divided by 24. The difference between the products of brackets (p -1) (p + 1) - (q -1) (q + 1) must also be divided by 24, and this is the difference of squares p² - q². Knowing this fact makes it possible to test another with one known square. This does not exclude the multiplicity of the difference of squares of ordinary odd numbers to 24, for example, 225 - 81 = 144 = 6 ∙ 24, but does not guarantee its 1225 - 441 = 784 ≠ 24k , for the difference of squares of ordinary odd and prime numbers , 225 - 49 = 176 ≠ 24k . For squares of prime numbers, this is guaranteed.

And about the values ​​of consecutive n-th and (n + 1) -th prime numbers. So, Euclid proved the validity of the relation for the primes p (n + 1) <p (1) ∙ p (2) ∙ p (3) ∙ .. ∙ p (n), where the values ​​in brackets denote the ordinal numbers of prime numbers. This is a rather rough estimate. Later it was shown that for n> 4, the relation takes the form p (n + 1) ² <p (1) p (2) p (3) ... p (n), i.e. already for
p (5) ² = 11² = 121 <p (1) ∙ p (2) ∙ p (3) ∙ p (4) = 2 3 5 7 = 210;
p (5) = 11 <√ (p (1) ∙ p (2) ∙ p (3) p (4)) = √ (2 ∙ 3 ​​∙ 5 ∙ 7) = √210 . This is a significantly improved score.
P.L. Chebyshev proved an even stronger result, p (n + 1) <2p (n).

Areas of a flat model, excluding the appearance of primes in them

Now let's take a closer look at the rays coming out of the central part of the helix and others, which were mentioned earlier and also do not contain cells with prime numbers.
We will call the vertical and horizontal lines of the spiral "highways", and the primes in their cells "traffic lights", limiting the speed of movement along the lines. Then on the spiral, you can specify the specific "high-speed" highway, going in the direction from the center in four directions: "North - South" and "West - East", not containing traffic lights at all. The cells of these highways are filled only with composite numbers, i.e. they do not contain prime numbers. The fact itself is quite remarkable and even surprising, possibly containing a “hint” on the nature and distribution of primes in the NPS. Even more surprising is the fact that the South and East highways contain two adjacent lanes, and the North and West each one. Within the k-th circuit, we denote the cells belonging to the highways with the symbols of the cardinal points: (k) - the northern highway; (k) - the western highway; J1 (k) - the southern first highway and J2 (k) - the southern second highway; B1 (k) is the eastern first highway and B2 (k) is the eastern second highway.

Theoretically, the highways of the three lanes are unlikely, since the strip perpendicular to the highways of the direction (like all the others) in the three adjacent cells contains adjacent natural numbers. Among these numbers, one of the three is always a multiple of three, that is, composite, and two of them are either even or odd. Let be a multiple of three in a cell on the side of a two-lane highway. Then the fourth number in the circuit through two cells will also be a multiple of three. Consequently, after two cells from the number, the cell on the other side also contains a composite number, which in some position may be simple. Even and odd numbers in cells of a two-lane backbone are placed in a checkerboard pattern and, apparently, there is a law governing the filling of odd cells of highways, precluding the appearance of prime numbers in them.
Pairs of odd numbers in adjacent cells of different highways turn out to be multiple consecutively increasing 3,5,7,9, ... odd numbers:
(27.51): 3; (85,125): 5; (175,231): 7; (297, 369): 9 ...... (east direction); 51 = 27 + 24; 125 = 85 +40;
231 = 175 + 56; 369 = 297 +72 increment of values ​​is a multiple of 8 with multiplicity-divisor of numbers.
(21.45): 3; (75.115): 5; (161,217): 7; (279.351): 9; ... (south direction).
The description of the entire infinite set of cells of the highways is simpler than for cells outside the highways. One coordinate - the number of the contour k of the spiral is enough to determine the number in the main line cell belonging to the specified contour number k. The following dependencies are used (see table).

Table. The calculated characteristics of the cells of the main lines of the Ulam spiral


The formulas given in the table guarantee, when defining the contour number k, unlimited continuation of the strips while preserving the marked properties of the strips and the numbers in them. A particularly important property is the property of divisibility of odd numbers in the cells forming the stripes. In each circuit, for a given k number, the values ​​in six cells at the intersection of highways with a contour are determined by the formulas in the table. All these numbers, let's call them the reference points , are composite and their divisors are known. The formulas in the table already contain descriptions for each divider.
Other numbers of the k-th contour ( reference frame ± t ∙ divider of the reference frame ) can also be subjected to the factorization procedure under certain conditions.

These conditions include the following. The test number differs from the rapper by a multiple of the smaller or greater divisor. For such numbers, factorization is performed without problems. The property of divisibility of odd numbers in the cells forming the stripes is important. Consequently, in each contour there are more than 8 cells containing odd numbers, which cannot be simple, since in the expression ( reference ± t divider of the reference ) the common divider is put out for the bracket.

In addition to the considered lines, there are lines (diagonal) of odd numbers that also do not contain prime numbers. First, such an odd diagonal is the diagonal of odd squares. Even diagonals, which obviously do not contain primes, are pressed to it before and after it. In each contour at the same time there are three adjacent cells without prime numbers. Secondly, three more adjacent cells without prime numbers in each contour appear adjacent to the diagonal of even squares. This diagonal is preceded by an odd diagonal, in which, as we now show, all cells are occupied by composite numbers. The values ​​in the cells of this diagonal are equal to the even square (2k) ² - 1 without one. But such a relation is always decomposed into a product of two brackets — a sum with a unit and a difference with a unit of the first degree of an even square of the contour, (2k + 1) (2k - 1).
An example . Let the number N = 4294967297 = F5 be given. It is required to determine its position in the model, i.e. (x1, x2) coordinates of the cell with this number, as well as the values ​​and positions of the six numbers belonging to this contour, on the highways: C = ?; B1 = ?; B2 = ?; J1 =? J2 =?; H =?
Decision. We extract the square root of the number N and round it to a smaller odd number. The value of the extracted square root is 65536.0000076. The smallest nearest integer is 65536 = 2k, (hence x1 = k = 32768 ) an even number, an odd number less than 65536 - 1 = 65535. This number is odd and the cell containing its square is Ch (k) = 4294836225, is the starting cell of the contour containing the number
N = F5 = 4294967297. The second coordinate x2 = 4294967297 - 4294836225 = 131072. So, for the number F5, a pair of coordinates (x1, x2) = (32768.131072) is obtained. The first coordinate of all frames is the same - this is the contour number x1 = 32768. The second coordinates x2 are different for all frames.
Contour cells belonging to highways are guaranteed a multiplicative representation.
C = 4294934528 = 32768 131072; W = 4295000064 = 32768 131073; H1 = 4295065599 = 32769 131071;
R2 = 4295065600 = 32768 131075; B1 = 429 486 8991 = 32767 131073; B2 = 4294868992 = 32768 131069.
Highway cells receive coordinates: the beginning of the contour NC (x1, x2) = (32768, 0);
B1 (x1, x2) = (32768, 32766); B2 (x1, x2) = (32768, 32767); C (x1, x2) = (32768, 98303);
H (x1, x2) = (32768, 63839); U1 (x1, x2) = (32768, 229374); U2 (x1, x2) = (32768, 229375);
The number in the contour before the even square (2k) ² - 1 = (2k +1) (2k - 1) = 6553565537 = 4294967295; guaranteed composite; the number behind the even square also turned out to be a compound (2k) ² + 1 = 641x6700417.