Effective mental arithmetic or brain warm-up
This article is inspired by the topic “How and how fast do you feel in your mind at the elementary level?” And is intended to spread the techniques of S.. Rachinsky for an oral account.
Raczynski was a wonderful teacher who taught in rural schools in the 19th century and showed from his own experience that it was possible to develop the skill of a quick oral account. For his students, it was not a particular problem to find a similar example in mind:
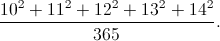
One of the most common oral account techniques is that any number can be represented as a sum or difference of numbers, one or more of which is “round”:
')

Since by 10 , 100 , 1000, and others. multiply round numbers faster, in your mind you need to reduce everything to such simple operations as 18 x 100 or 36 x 10 . Accordingly, it is easier to fold, “splitting off” a round number, and then adding a “tail”: 1800 + 200 + 190 .
Another example:
With an oral account, it is more convenient to operate with a divisible and divisor rather than an integer (for example, 5 should be represented as 10: 2 , and 50 as 100: 2 ):
Similarly, multiplication or division by 25 , because 25 = 100: 4 . For example,
Now it does not seem impossible to multiply in mind 625 by 53 :
It turns out that to simply build any two-digit number into a square, it suffices to remember the squares of all numbers from 1 to 25 . Fortunately, the squares to 10, we already know from the multiplication table. The remaining squares can be viewed in the table below:

Reception Rachinsky is as follows. In order to find the square of any two-digit number, multiply the difference between this number and 25 by 100 and add to the resulting product the square of the complement of this number to 50 or the square of its excess over the 50th . For example,
In general, ( M is a two-digit number):
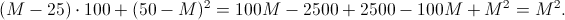
Let's try to apply this trick when squaring a three-digit number, breaking it up previously into smaller components:
Hmm, I would not say that it is much easier than erection, but perhaps you can adapt with time.
And, of course, one should start training with squaring two-digit numbers, and there one can even walk to disassemble in one's mind.
This interesting technique was invented by a 12-year-old student of Rachinsky and is one of the options for adding up to a round number.
Let two two-digit numbers be given, in which the sum of the units is 10:
Compiling their work, we get:
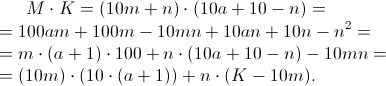
For example, calculate 77 x 13 . The sum of these numbers is 10 , because 7 + 3 = 10 . First, put a smaller number before a large one: 77 x 13 = 13 x 77 .
To get round numbers, we take three units from 13 and add them to 77 . Now we multiply the new numbers 80 x 10 , and add the product of the selected 3 units to the result obtained by the difference of the old number 77 and the new number 10 :
This technique has a special case: everything is greatly simplified when the two factors have the same number of tens. In this case, the number of tens is multiplied by the next number and the product of the units of these numbers is attributed to the result. Let's see how elegant this technique is by example.
48 x 42 . The number of tens of 4 , the following number: 5 ; 4 x 5 = 20 . The product of the units: 8 x 2 = 16 . So
99 x 91 . Number of tens: 9 ; subsequent number: 10 ; 9 x 10 = 90 . The product of the units: 9 x 1 = 09 . So
Aha, that is, to multiply 95 x 95 , it is enough to count 9 x 10 = 90 and 5 x 5 = 25 and the answer is ready:
Then the previous example can be calculated a little easier:
It would seem, why be able to count in your mind in the 21st century, when you can just give a voice command to a smartphone? But if you think about what will happen to humanity, if it charges not only physical work, but any mental work, too? Does it degrade? Even if you do not consider the oral account as an end in itself, it is well suited for hardening the mind.
References :
“1001 tasks for mental accounts in school S.A. Raczynski .
Raczynski was a wonderful teacher who taught in rural schools in the 19th century and showed from his own experience that it was possible to develop the skill of a quick oral account. For his students, it was not a particular problem to find a similar example in mind:
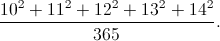
Use round numbers
One of the most common oral account techniques is that any number can be represented as a sum or difference of numbers, one or more of which is “round”:
')

Since by 10 , 100 , 1000, and others. multiply round numbers faster, in your mind you need to reduce everything to such simple operations as 18 x 100 or 36 x 10 . Accordingly, it is easier to fold, “splitting off” a round number, and then adding a “tail”: 1800 + 200 + 190 .
Another example:
31 x 29 = (30 + 1) x (30 - 1) = 30 x 30 - 1 x 1 = 900 - 1 = 899. Simplify division multiplication
With an oral account, it is more convenient to operate with a divisible and divisor rather than an integer (for example, 5 should be represented as 10: 2 , and 50 as 100: 2 ):
68 x 50 = (68 x 100) : 2 = 6800 : 2 = 3400; 3400 : 50 = (3400 x 2) : 100 = 6800 : 100 = 68. Similarly, multiplication or division by 25 , because 25 = 100: 4 . For example,
600 : 25 = (600 : 100) x 4 = 6 x 4 = 24; 24 x 25 = (24 x 100) : 4 = 2400 : 4 = 600. Now it does not seem impossible to multiply in mind 625 by 53 :
625 x 53 = 625 x 50 + 625 x 3 = (625 x 100) : 2 + 600 x 3 + 25 x 3 = (625 x 100) : 2 + 1800 + (20 + 5) x 3 = = (60000 + 2500) : 2 + 1800 + 60 + 15 = 30000 + 1250 + 1800 + 50 + 25 = 33000 + 50 + 50 + 25 = 33125. Squaring a two-digit number
It turns out that to simply build any two-digit number into a square, it suffices to remember the squares of all numbers from 1 to 25 . Fortunately, the squares to 10, we already know from the multiplication table. The remaining squares can be viewed in the table below:

Reception Rachinsky is as follows. In order to find the square of any two-digit number, multiply the difference between this number and 25 by 100 and add to the resulting product the square of the complement of this number to 50 or the square of its excess over the 50th . For example,
37^2 = 12 x 100 + 13^2 = 1200 + 169 = 1369; 84^2 = 59 x 100 + 34^2 = 5900 + 9 x 100 + 16^2 = 6800 + 256 = 7056; In general, ( M is a two-digit number):
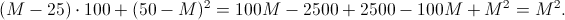
Let's try to apply this trick when squaring a three-digit number, breaking it up previously into smaller components:
195^2 = (100 + 95)^2 = 10000 + 2 x 100 x 95 + 95^2 = 10000 + 9500 x 2 + 70 x 100 + 45^2 = 10000 + (90+5) x 2 x 100 + + 7000 + 20 x 100 + 5^2 = 17000 + 19000 + 2000 + 25 = 38025. Hmm, I would not say that it is much easier than erection, but perhaps you can adapt with time.
And, of course, one should start training with squaring two-digit numbers, and there one can even walk to disassemble in one's mind.
Multiplication of two-digit numbers
This interesting technique was invented by a 12-year-old student of Rachinsky and is one of the options for adding up to a round number.
Let two two-digit numbers be given, in which the sum of the units is 10:
M = 10m + n, K = 10a + 10 - n. Compiling their work, we get:
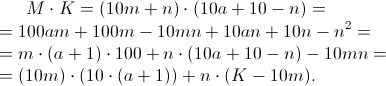
For example, calculate 77 x 13 . The sum of these numbers is 10 , because 7 + 3 = 10 . First, put a smaller number before a large one: 77 x 13 = 13 x 77 .
To get round numbers, we take three units from 13 and add them to 77 . Now we multiply the new numbers 80 x 10 , and add the product of the selected 3 units to the result obtained by the difference of the old number 77 and the new number 10 :
13 x 77 = 10 x 80 + 3 x (77 - 10) = 800 + 3 x 67 = 800 + 3 x (60 + 7) = 800 + 3 x 60 + 3 x 7 = 800 + 180 + 21 = 800 + 201 = 1001. This technique has a special case: everything is greatly simplified when the two factors have the same number of tens. In this case, the number of tens is multiplied by the next number and the product of the units of these numbers is attributed to the result. Let's see how elegant this technique is by example.
48 x 42 . The number of tens of 4 , the following number: 5 ; 4 x 5 = 20 . The product of the units: 8 x 2 = 16 . So
48 x 42 = 2016. 99 x 91 . Number of tens: 9 ; subsequent number: 10 ; 9 x 10 = 90 . The product of the units: 9 x 1 = 09 . So
99 x 91 = 9009. Aha, that is, to multiply 95 x 95 , it is enough to count 9 x 10 = 90 and 5 x 5 = 25 and the answer is ready:
95 x 95 = 9025. Then the previous example can be calculated a little easier:
195^2 = (100 + 95)^2 = 10000 + 2 x 100 x 95 + 95^2 = 10000 + 9500 x 2 + 9025 = 10000 + (90+5) x 2 x 100 + 9000 + 25 = = 10000 + 19000 + 1000 + 8000 + 25 = 38025. Instead of conclusion
It would seem, why be able to count in your mind in the 21st century, when you can just give a voice command to a smartphone? But if you think about what will happen to humanity, if it charges not only physical work, but any mental work, too? Does it degrade? Even if you do not consider the oral account as an end in itself, it is well suited for hardening the mind.
References :
“1001 tasks for mental accounts in school S.A. Raczynski .
Source: https://habr.com/ru/post/207034/
All Articles