Cutting into two equal parts, part three
First part
The first part of the second part
The second part of the second part
Well, gentlemen, it's time to finish. In the last article of the cycle (the name of which breaks my template that hasn't really woken up yet) we will put a fat point in the history of this task. Despite the fact that in the comments to the second part, a more convenient and universal way was proposed to do this, I still use the tools developed by me personally before writing the first article. First, do not disappear as good, and secondly, I think everyone understands that the task - it's just a reason to draw beautiful drawings in GeoGebra and post them to Habr. Well, as they say, rushed.

')
The sliding symmetry is determined by the following parameters: the axis of symmetry and the shear vector parallel to it. The axis of symmetry, in turn, is determined by the direction and specific position on the plane. Now I will outline a number of facts which follow each other, sufficiently obvious not to call them even Lemmas, and certainly not to prove them.
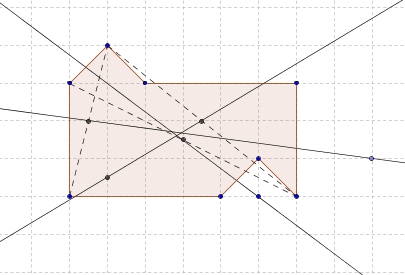
Armed with these facts, I drew such a picture:

It shows that for most of the directions, either the points A and E, or the points B and F will appear on the borders, and therefore, the axis will pass through the “center” of the figure (the intersection of the diagonals of the rectangle ABEF). The second most common is the case with points C and F, the honorable third is with points C and A. In these cases, obviously, the axis of symmetry will pass through the midpoints of the segments CF and CA, respectively.
Now let's see in which directions the axis will divide the figure into two equal parts. Without going into tedious details, I’ll say simply: but with such.
Now we have three specific axes and, moreover, three concrete shift vectors are attached to them - in fact, the borders in all cases consist of one point. It is easy to show that for all these three sliding symmetries there are points of a figure for which there is neither an image nor a pre-image - which, according to Lemma 3, means that these are bad, unfit sliding symmetries that cannot correspond to splitting the figure into two equal parts. Search for specific points I leave the reader.
Figure A 0 cannot be cut into two equal parts. Now, with a clear conscience, I will go to revise "And the dawns here are quiet." Happy Victory Day, comrades habrovchane.
The first part of the second part
The second part of the second part
Well, gentlemen, it's time to finish. In the last article of the cycle (the name of which breaks my template that hasn't really woken up yet) we will put a fat point in the history of this task. Despite the fact that in the comments to the second part, a more convenient and universal way was proposed to do this, I still use the tools developed by me personally before writing the first article. First, do not disappear as good, and secondly, I think everyone understands that the task - it's just a reason to draw beautiful drawings in GeoGebra and post them to Habr. Well, as they say, rushed.

')
Case 3: sliding symmetry
The sliding symmetry is determined by the following parameters: the axis of symmetry and the shear vector parallel to it. The axis of symmetry, in turn, is determined by the direction and specific position on the plane. Now I will outline a number of facts which follow each other, sufficiently obvious not to call them even Lemmas, and certainly not to prove them.
- The axis of sliding symmetry is equidistant from the boundaries (we take the concept of the boundary smoothly from the case of parallel transport).
- If we take a segment with ends at different boundaries, then the axis of symmetry will pass through its middle.
- By specifying the direction of the axis of sliding symmetry, we automatically recognize its specific position. If both borders consist of a single point, then we also know the shift vector.
- The parts into which the figure is divided by the axis have an equal area (this fact does not follow from the previous ones)
Armed with these facts, I drew such a picture:

It shows that for most of the directions, either the points A and E, or the points B and F will appear on the borders, and therefore, the axis will pass through the “center” of the figure (the intersection of the diagonals of the rectangle ABEF). The second most common is the case with points C and F, the honorable third is with points C and A. In these cases, obviously, the axis of symmetry will pass through the midpoints of the segments CF and CA, respectively.
Now let's see in which directions the axis will divide the figure into two equal parts. Without going into tedious details, I’ll say simply: but with such.

Now we have three specific axes and, moreover, three concrete shift vectors are attached to them - in fact, the borders in all cases consist of one point. It is easy to show that for all these three sliding symmetries there are points of a figure for which there is neither an image nor a pre-image - which, according to Lemma 3, means that these are bad, unfit sliding symmetries that cannot correspond to splitting the figure into two equal parts. Search for specific points I leave the reader.
Conclusion
Figure A 0 cannot be cut into two equal parts. Now, with a clear conscience, I will go to revise "And the dawns here are quiet." Happy Victory Day, comrades habrovchane.
Source: https://habr.com/ru/post/179173/
All Articles