Cutting into two equal parts, the second part of the second part
First part
The first part of the second part
The May holidays continue, the number of uses of the word “part” per line of text is overwhelming, and we, dear readers, finally finish off the case of turning with the center inside the figure.
')
To begin with, we use the property of the far boundary section. If this sentence is a meaningless set of letters for you, you should familiarize yourself with the previous two parts. If not, then here is a beautiful color picture, which is, in fact, a continuation of a similar picture from the previous part. Let me remind you: each of the colored areas is a locus of points for which, if they were a center of rotation, the boundary section would consist of a single vertex colored in the corresponding color. Since a section cannot consist of a single point (see Lemma 1), the center of rotation can be located only on the boundary between the regions. Thus, the set of possible positions of the center of rotation is narrowed to a set of segments. Next, we will try to narrow it down to an empty set.

Due to the absence of an internal border (and the associated criterion), we cannot deal with this case as famously as with the case of the center outside the figure. However, something we still can. Let's start with the following reasoning: take a secant slightly smaller radius than the outer border. Then the section (at least in the case of this particular shape) will consist of two or more small-small arcs. Since their angular measure will be less than the angle of rotation, when mapping from B to C, they have nothing left to do but go into each other. Accordingly, if we have exactly two small arcs, then these arcs must have an equal angular measure. We show that in the case of our figure, this criterion is systematically violated.
Take an arbitrary point O on the segment i and assign it to the center. Conduct a secant slightly smaller radius than the outer boundary. Then the section will consist of two rather small arcs: LP and QS. At the same time, QS = QR + RS. However, LP = QR due to symmetry. Therefore, LP QS, which means that the point O can in no way be the center of rotation.

We can rotate a similar trick with segments j, k, m, p. For the sake of at least some intelligibility, I have removed all unnecessary from the drawing, but still I don’t understand a damn thing, because, as mentioned above, the arcs are very, very small. The dotted arcs are obviously part of the secant; bold highlighted, in fact, sections. Dotted cuts are those tricky cuts that, similarly to the case of segment i, separate a piece from one of the section arcs equal to the other arc, thus showing that the entire first arc will be larger than the second.

There remains a segment n, for which it is not possible so conveniently to show the inequality of small arcs. In view of this, we will need to slightly expand the existing mathematical apparatus by adding the following lemma to it:
Lemma 3. For each point of A, its image or prototype also belongs to A.
What does this lemma give us? And the fact that it is enough for us to find a point that belongs to our figure, but when rotated to the corresponding angle, clockwise and counterclockwise, ceases to belong to it. Such a find would mean that this turn is not good.
For these dirty purposes, we take the point K. If we move the center of rotation O along the segment n, then the images of the point K, when turning plus or minus the angle POQ, will move along the corresponding bold arcs of the corresponding dotted circles (it is useful here to clarify that points P and Q the essence of the boundary section for the center lying on the segment n, and, accordingly, the angle POQ - this is exactly the angle of rotation). It is easy to see that these arcs never intersect with the figure A 0 . Therefore, the center of rotation can not be located on the segment n.
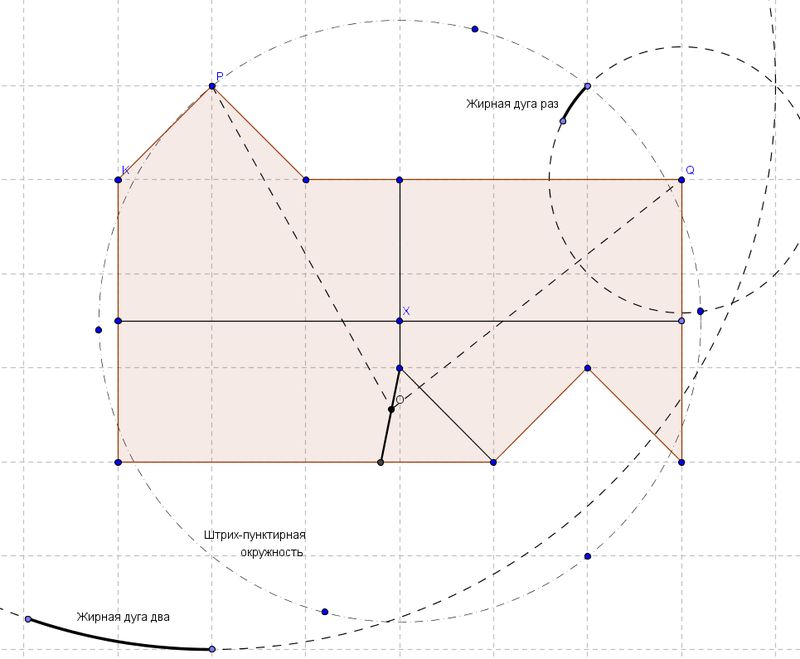
Pay attention also to the dot-dotted circle on which the point P is located and five more nameless points are marked (the end of the “fat arc times” does not count, it got there by chance). The point is that the point X, which is in the center of the figure, is special. For her, the boundary section will consist of not two, but as many as four points - respectively, the proof based on “small arcs” does not work for her. However, the proof based on Lemma 3 works remarkably. The five unnamed points on the dash-dotted circle are the images of the point P for all possible turns corresponding to the boundary section corresponding to the point X (I apologize for the abuse of the word "corresponding"). None of these images falls in the figure A 0 - therefore, point X is also excluded.
There is one more special point with a special boundary section. I give attentive readers the right to find it and prove that it also cannot be the center of the turn. It is not difficult.
If a figure A 0 and can be cut into two equal figures B and C, then B is not translated into C either by a parallel translation or by a turn. Only sliding symmetry remains. The last article of the cycle, in which I will deal with this case, will be published on May 9, which symbolizes the final victory over the task, which at one time caused so much rustle.
The first part of the second part
The May holidays continue, the number of uses of the word “part” per line of text is overwhelming, and we, dear readers, finally finish off the case of turning with the center inside the figure.

')
Case 2.2: the center of rotation inside the figure
To begin with, we use the property of the far boundary section. If this sentence is a meaningless set of letters for you, you should familiarize yourself with the previous two parts. If not, then here is a beautiful color picture, which is, in fact, a continuation of a similar picture from the previous part. Let me remind you: each of the colored areas is a locus of points for which, if they were a center of rotation, the boundary section would consist of a single vertex colored in the corresponding color. Since a section cannot consist of a single point (see Lemma 1), the center of rotation can be located only on the boundary between the regions. Thus, the set of possible positions of the center of rotation is narrowed to a set of segments. Next, we will try to narrow it down to an empty set.

Due to the absence of an internal border (and the associated criterion), we cannot deal with this case as famously as with the case of the center outside the figure. However, something we still can. Let's start with the following reasoning: take a secant slightly smaller radius than the outer border. Then the section (at least in the case of this particular shape) will consist of two or more small-small arcs. Since their angular measure will be less than the angle of rotation, when mapping from B to C, they have nothing left to do but go into each other. Accordingly, if we have exactly two small arcs, then these arcs must have an equal angular measure. We show that in the case of our figure, this criterion is systematically violated.
Take an arbitrary point O on the segment i and assign it to the center. Conduct a secant slightly smaller radius than the outer boundary. Then the section will consist of two rather small arcs: LP and QS. At the same time, QS = QR + RS. However, LP = QR due to symmetry. Therefore, LP QS, which means that the point O can in no way be the center of rotation.

We can rotate a similar trick with segments j, k, m, p. For the sake of at least some intelligibility, I have removed all unnecessary from the drawing, but still I don’t understand a damn thing, because, as mentioned above, the arcs are very, very small. The dotted arcs are obviously part of the secant; bold highlighted, in fact, sections. Dotted cuts are those tricky cuts that, similarly to the case of segment i, separate a piece from one of the section arcs equal to the other arc, thus showing that the entire first arc will be larger than the second.

There remains a segment n, for which it is not possible so conveniently to show the inequality of small arcs. In view of this, we will need to slightly expand the existing mathematical apparatus by adding the following lemma to it:
Lemma 3. For each point of A, its image or prototype also belongs to A.
Proof: obvious. Let the point belong to the figure B, then its image belongs to the figure C and, therefore, to the figure A. Similarly, if the point belongs to the figure C.
What does this lemma give us? And the fact that it is enough for us to find a point that belongs to our figure, but when rotated to the corresponding angle, clockwise and counterclockwise, ceases to belong to it. Such a find would mean that this turn is not good.
For these dirty purposes, we take the point K. If we move the center of rotation O along the segment n, then the images of the point K, when turning plus or minus the angle POQ, will move along the corresponding bold arcs of the corresponding dotted circles (it is useful here to clarify that points P and Q the essence of the boundary section for the center lying on the segment n, and, accordingly, the angle POQ - this is exactly the angle of rotation). It is easy to see that these arcs never intersect with the figure A 0 . Therefore, the center of rotation can not be located on the segment n.

Pay attention also to the dot-dotted circle on which the point P is located and five more nameless points are marked (the end of the “fat arc times” does not count, it got there by chance). The point is that the point X, which is in the center of the figure, is special. For her, the boundary section will consist of not two, but as many as four points - respectively, the proof based on “small arcs” does not work for her. However, the proof based on Lemma 3 works remarkably. The five unnamed points on the dash-dotted circle are the images of the point P for all possible turns corresponding to the boundary section corresponding to the point X (I apologize for the abuse of the word "corresponding"). None of these images falls in the figure A 0 - therefore, point X is also excluded.
There is one more special point with a special boundary section. I give attentive readers the right to find it and prove that it also cannot be the center of the turn. It is not difficult.
Conclusion
If a figure A 0 and can be cut into two equal figures B and C, then B is not translated into C either by a parallel translation or by a turn. Only sliding symmetry remains. The last article of the cycle, in which I will deal with this case, will be published on May 9, which symbolizes the final victory over the task, which at one time caused so much rustle.
Source: https://habr.com/ru/post/178703/
All Articles