Quantum mechanics for all, for nothing, and let no one leave offended: part one
Hello! I would like to bring to your attention a great introduction to quantum mechanics, written by Eliezer Yudkovsky; perhaps, he is known to you from his website lesswrong.com, dedicated to rationalism, prejudice, cognitive paradoxes, and many more interesting things.
read the second part →
I warn you at once: this cycle of articles differs markedly from the traditional introduction to quantum mechanics.
First , I will not quote Richard Feynman, who once said that "it is normal not to understand quantum mechanics, because no one understands it." Once it was, but times change.
')
I will not say: "Quantum mechanics can not be understood, just need to get used to it." (This quote is attributed to John von Neumann; he lived in those dark times when no one really understood quantum mechanics.)
You can not end the explanation with the words "If something is not clear, it should be so." No, it should not be . Maybe the problem is you. Maybe - in your teacher. In any case, it must be solved , and not sit back and reassure themselves that everyone else does not understand anything either.
I will not say that quantum mechanics is something strange , confusing, or inaccessible to human understanding . Yes, it is counterintuitive - but this is a disaster solely by our intuition. Quantum mechanics originated long before the Sun, the planet Earth, or human civilization. She's not going to change for you. In general, there are no discouraging facts , only theories that are discouraged by facts ; and if the theory does not coincide with the practice, it does not do her honor.
You should always consider reality as a completely mundane thing. Since the beginning of time, nothing unusual has happened in the universe.
Our goal is to learn how to feel at home in this quantum world. Because we are already at home.
Throughout this cycle, I will speak of quantum mechanics as the most common theory; and where the intuitive view of the world does not coincide with it, I will make fun of intuition for the inconsistency of reality.
Secondly , I am not going to follow the traditional order of studying quantum mechanics, which copies the order in which it was discovered.
Usually, it all starts with the story that matter sometimes behaves like a bunch of small billiard balls colliding with each other, and sometimes like waves on the surface of a pool. This is accompanied by several examples that illustrate both views of matter.
Earlier, when all this was only born and no one had a clue about the mathematical foundations of physics, scientists seriously believed that everything consists of atoms that behave approximately like billiard balls. And then they began to believe that everything consists of waves. And then they again returned to the billiard balls. All this led to the fact that scientists finally got confused, and only a few decades later - by the end of the nineteenth century - did they manage to put everything in its place.
If we apply this historical authentic approach to teaching modern students (as they are doing now), the same thing that happened to the early scientists will naturally happen to them, namely, they will fall into complete and absolute confusion . Telling students of physics about wave-particle duality is the same as starting a course in chemistry with a lecture on four elements.
The electron is not like a billiard ball, nor the crest of an ocean wave. An electron is a completely different object from a mathematical point of view, and it remains so under any circumstances . And if you persist in your aspiration to consider him and that, and how you are more comfortable , I warn you: if you chase two hares, you will not catch one.
This is not the only reason why historical order is not the best choice. Let's follow the hypothetical process from the very beginning : people notice that they are surrounded by other animals - inside the animals, it turns out, there are organs - and organs, if you look closely, are made up of tissues - under a microscope you can see that tissues consist of cells - cells consist of proteins, and other chemical compounds - chemical compounds are composed of atoms - atoms are made of protons, neutrons and electrons - but the latter is much easier and more intuitive animals, with which it all began, but were discovered by tens of thousands of years poses e.
Physics do not begin to pass from biology. Then why should it begin with a discussion of laboratory experiments and their results, which, even in the case of the simplest experiments, are the result of many complex and intricate processes?
On the one hand, I can understand why the experiment is put at the forefront. We are talking about physics , after all.
On the other hand, to give students a complex mathematical apparatus in their hands only so that they can analyze simple experience — this is too much . Programmers, for example, are first taught to add two variables, and only then to write multi-threaded applications; and spit on the fact that the latter are “closer to real life”.
Classical mechanics does not follow explicitly from quantum mechanics. Moreover, classical mechanics is at a much higher level. Compare atoms and molecules with quarks: millions of chemicals known to science, hundreds of chemical elements, and a total of six quarks. First, it is better to understand the simple, and only then proceed to the complex.
Finally , I will consider quantum mechanics from a strictly realistic position — our world is quantum, our equations describe a territory, not its map, and the familiar world implicitly exists in the quantum world. If there are anti-realists among my readers, please keep your comments. Quantum mechanics are much harder to understand and imagine if you doubt its fairness. I will talk about this in more detail in one of the following articles.
I think that the point of view that I will present in this introduction is shared by most theoretical physicists. But you still need to know that this is not the only possible point of view, and a large proportion of scientists doubt the correctness of the realistic position. Although I am not going to pay attention to any other theories right now , I feel obliged to mention that they are .
To summarize , my goal is to teach you to think like a native of the quantum world , and not as a tourist necessarily .
Stronger hold on to reality. We begin.
Look at the pic. 1. At point A there is a semi-silvered mirror, and at points B and C - two photon detectors.

This simple experiment at one time made scientists smash their heads. The fact is that in half of the cases the photon released in the direction of the mirror was registered by the first detector, and in half by the second. And the scientists — attention, get ready to laugh — assumed that the mirror was either letting the photon through, or reflecting it.
Ha-ha-ha, imagine a mirror that can choose for itself, let it pass a photon or not miss it! If you can imagine it, then still do not do it - and then you will get confused just like those scientists. The mirror behaves in exactly the same way in both cases.
If we tried to write a computer program simulating this experiment (and not just predicting the result), it would look something like this ...
At the beginning of the program, we declare a variable that stores in itself a specific mathematical object — a configuration . It represents a certain description of the state of the world - in this case, “one photon flies to point A”.
In fact, the configuration is described by a complex number (recall that complex numbers have the form (a + b i ), where a and b are real numbers, and i is an imaginary unit, that is, a number such that i ² = -1) . Our configuration "photon flies to point A " also corresponds to some number. Let it be (-1 + 0 i ). In the following, we will call the number corresponding to the configuration its amplitude .
We introduce two more configurations: “the photon flies from A to point B ” and “the photon flies from A to point C ”. We do not yet know the amplitudes of these configurations; they will be assigned values during program execution.

The amplitudes can be calculated by applying the rule that the mirror operates to the initial configuration. Without going into details, we can assume that the rule looks like this: “multiply by 1 when the photon flies by; multiply by i when the photon is reflected. ” Apply the rule: the amplitude of the configuration “photon flies to B ” equals (-1 + 0 i ) × i = (0 + - i ), and the amplitude of the configuration “photon flies to C ” equals (-1 + 0 i ) × 1 = ( -1 + 0 i ). Other configurations in fig. 1 no, so we're done.
In principle, we can consider “the first detector registers a photon” and “the second detector registers a photon” as separate configurations, but this does not change anything; their amplitudes will be equal to the amplitudes of the two previous configurations, respectively. (In fact , they still need to be multiplied by a factor equal to the distance from A to the detectors, but we simply assume that all distances in our experiment are unit multipliers.)
So, here is the final state of the program:
And, perhaps:
Of course, no matter how many times we run the program, the final state will remain the same.
Now, for rather complex reasons, which I won't go into yet, there is no easy way to measure the amplitude of the configuration. The state of the program is hidden from us.
What to do?
Although we cannot measure amplitude directly, we have something - namely, a magic measuring thing that can tell us the square of the magnitude module of the configuration. In other words, for amplitude (a + b i ), the thing will answer with a number (a² + b²).
It would be more accurate to say that the magic thing finds only the ratio of the squares of the modules to each other. But even this information is enough to understand what is happening inside the program and according to what laws it works.
With the help of the gizmo, we can easily find out that the squares of the modules of the configurations “triggered the first detector” and “triggered the second detector” are equal. And having conducted some more complex experiments, we can also find out the ratio of the amplitudes themselves - i to 1.
By the way, what is this magic measuring thing like that?
Well, when such experiments are carried out in real life, the fact that the experiment is carried out a couple of thousand times serves as a magic thing and they simply count how many times the photon is in the first detector and how many in the second. The ratio of these values will be the ratio of the squares of the amplitude modules. Why it will be so - the question is different, much more complicated. In the meantime, you can use the thing and without understanding how yes why it works. Everything has its time.
You may ask: “But why do we need quantum theory at all, if its predictions coincide with the predictions of the billiard theory?” There are two reasons. First, the reality , whatever you think, is still subject to the quantum laws - amplitudes, complex numbers, and so on. And secondly, the "billiard" theory does not work for any more or less complex experiment. Want an example? You are welcome.
In fig. 2 you can see two mirrors at points B and C , and two half-mirrors at points A and D. Later I will explain why the segment DE is drawn by a dotted line; on the calculations it does not affect.
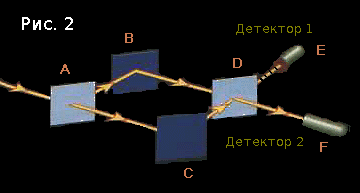
Let's apply the rules we already know.
At the beginning, we have the configuration “photon flies to A ”, its amplitude is (-1 + 0 i ).
We consider the amplitudes of the configurations "photon flies from A to B " and "photon flies from A to C ":
It is intuitively clear that an ordinary mirror behaves like a half-mirror: it always reflects a photon, it always multiplies the amplitude by i . So:
It is important to understand that “from B to D ” and “from C to D ” are two different configurations. You can't just write “photon flies to D ”, because what happens next will depend on the angle at which this photon comes in D.
We consider further:
Total:
The ratio of the squares of the amplitude modules is 0 to 4; it follows from the calculations that the first detector will not work at all ! Therefore, the segment DE was drawn by a dotted line in Fig. 2

If the half-mirrors had reflected or passed the photon randomly, both detectors would react with approximately the same frequency. But this does not coincide with the results of the experiments. That's all.
You could argue: “And that's not all! Suppose, for example, that when a mirror reflects a photon, something happens to it that the second time it does not reflect? Conversely, when the mirror misses the photon, the next time it will have to reflect. "
First, Occam's razor. There is no need to invent a complex explanation, if there is already a simple (if, of course, we consider quantum mechanics simple ...) And secondly, I can come up with another experience that will refute this alternative theory.
Place a small opaque object between B and D so that the amplitude of the “photon flies from B to D ” configuration is always zero.
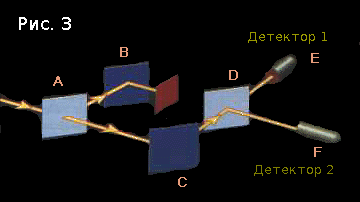
Now the amplitude of the “photon flies from D to F ” configuration is (1 + 0 i ), and the amplitude of the “photon flies from D to E ” configuration is (0 + - i ). The squares of the modules are equal to 1. This means that in half of the cases the first detector will be triggered, and in half the second.
It is impossible to explain, if we assume that the photon is a small billiard ball, which is reflected from the mirrors.
The fact is that amplitude cannot be thought of as probability. In probability theory, if an event X can occur or not occur, then the probability of an event Z is equal to P ( Z | X ) P ( X ) + P ( Z | ¬ X ) P (¬ X ), where all the probabilities are positive. If you know that the probability of Z , provided that X has happened, is 0.5, and the probability of X is 0.3, then the total probability of Z is at least 0.15, regardless of what happens if X does not happen. There are no negative probabilities. Possible and impossible events cannot cancel each other. And the amplitudes - can.

Here is an example of wrong thinking: “A photon flies to B or C , but it could fly differently, and this affects the likelihood that it will fly to E ...”
Events that did not happen have no influence on the world. The only thing that can affect the world is our imagination. "Oh my God, this car almost hit me," you think, and decide to go to the monastery, never to meet with dangerous cars. But the real thing is still not the event itself , but only your imagination contained in your brain - which can be pulled out of you, felt and put back in order to make sure that it is quite real.
Really everything that affects the world. (If you think this is not the case, try defining the word “real.”) Configurations and amplitudes directly affect the world, so they are also real. To say that a configuration is “what could have happened” is as strange as saying that a chair is “what could have happened.”
What is this configuration then?
To be continued.
In fact, everything is a little more complicated than you might have thought after reading this article.
Each configuration describes all the particles in the universe. Amplitude is a continuous distribution over the entire configuration space, and not a discrete one, as we have considered today. Indeed, photons do not teleport from one place to another instantly , and each different state of the world is described by a new configuration. In the end we will get to that.
If you do not understand anything of this paragraph, do not worry, I will explain everything. Then.
read the second part →
Author: Eliezer Yudkowsky. Free and abbreviated (quite a bit) translation: me. Links to originals:
read the second part →
Introduction to Introduction
I warn you at once: this cycle of articles differs markedly from the traditional introduction to quantum mechanics.
First , I will not quote Richard Feynman, who once said that "it is normal not to understand quantum mechanics, because no one understands it." Once it was, but times change.
')
I will not say: "Quantum mechanics can not be understood, just need to get used to it." (This quote is attributed to John von Neumann; he lived in those dark times when no one really understood quantum mechanics.)
You can not end the explanation with the words "If something is not clear, it should be so." No, it should not be . Maybe the problem is you. Maybe - in your teacher. In any case, it must be solved , and not sit back and reassure themselves that everyone else does not understand anything either.
I will not say that quantum mechanics is something strange , confusing, or inaccessible to human understanding . Yes, it is counterintuitive - but this is a disaster solely by our intuition. Quantum mechanics originated long before the Sun, the planet Earth, or human civilization. She's not going to change for you. In general, there are no discouraging facts , only theories that are discouraged by facts ; and if the theory does not coincide with the practice, it does not do her honor.
You should always consider reality as a completely mundane thing. Since the beginning of time, nothing unusual has happened in the universe.
Our goal is to learn how to feel at home in this quantum world. Because we are already at home.
Throughout this cycle, I will speak of quantum mechanics as the most common theory; and where the intuitive view of the world does not coincide with it, I will make fun of intuition for the inconsistency of reality.
Secondly , I am not going to follow the traditional order of studying quantum mechanics, which copies the order in which it was discovered.
Usually, it all starts with the story that matter sometimes behaves like a bunch of small billiard balls colliding with each other, and sometimes like waves on the surface of a pool. This is accompanied by several examples that illustrate both views of matter.
Earlier, when all this was only born and no one had a clue about the mathematical foundations of physics, scientists seriously believed that everything consists of atoms that behave approximately like billiard balls. And then they began to believe that everything consists of waves. And then they again returned to the billiard balls. All this led to the fact that scientists finally got confused, and only a few decades later - by the end of the nineteenth century - did they manage to put everything in its place.
If we apply this historical authentic approach to teaching modern students (as they are doing now), the same thing that happened to the early scientists will naturally happen to them, namely, they will fall into complete and absolute confusion . Telling students of physics about wave-particle duality is the same as starting a course in chemistry with a lecture on four elements.
The electron is not like a billiard ball, nor the crest of an ocean wave. An electron is a completely different object from a mathematical point of view, and it remains so under any circumstances . And if you persist in your aspiration to consider him and that, and how you are more comfortable , I warn you: if you chase two hares, you will not catch one.
This is not the only reason why historical order is not the best choice. Let's follow the hypothetical process from the very beginning : people notice that they are surrounded by other animals - inside the animals, it turns out, there are organs - and organs, if you look closely, are made up of tissues - under a microscope you can see that tissues consist of cells - cells consist of proteins, and other chemical compounds - chemical compounds are composed of atoms - atoms are made of protons, neutrons and electrons - but the latter is much easier and more intuitive animals, with which it all began, but were discovered by tens of thousands of years poses e.
Physics do not begin to pass from biology. Then why should it begin with a discussion of laboratory experiments and their results, which, even in the case of the simplest experiments, are the result of many complex and intricate processes?
On the one hand, I can understand why the experiment is put at the forefront. We are talking about physics , after all.
On the other hand, to give students a complex mathematical apparatus in their hands only so that they can analyze simple experience — this is too much . Programmers, for example, are first taught to add two variables, and only then to write multi-threaded applications; and spit on the fact that the latter are “closer to real life”.
Classical mechanics does not follow explicitly from quantum mechanics. Moreover, classical mechanics is at a much higher level. Compare atoms and molecules with quarks: millions of chemicals known to science, hundreds of chemical elements, and a total of six quarks. First, it is better to understand the simple, and only then proceed to the complex.
Finally , I will consider quantum mechanics from a strictly realistic position — our world is quantum, our equations describe a territory, not its map, and the familiar world implicitly exists in the quantum world. If there are anti-realists among my readers, please keep your comments. Quantum mechanics are much harder to understand and imagine if you doubt its fairness. I will talk about this in more detail in one of the following articles.
I think that the point of view that I will present in this introduction is shared by most theoretical physicists. But you still need to know that this is not the only possible point of view, and a large proportion of scientists doubt the correctness of the realistic position. Although I am not going to pay attention to any other theories right now , I feel obliged to mention that they are .
To summarize , my goal is to teach you to think like a native of the quantum world , and not as a tourist necessarily .
Stronger hold on to reality. We begin.
Configurations and amplitudes
Look at the pic. 1. At point A there is a semi-silvered mirror, and at points B and C - two photon detectors.

This simple experiment at one time made scientists smash their heads. The fact is that in half of the cases the photon released in the direction of the mirror was registered by the first detector, and in half by the second. And the scientists — attention, get ready to laugh — assumed that the mirror was either letting the photon through, or reflecting it.
Ha-ha-ha, imagine a mirror that can choose for itself, let it pass a photon or not miss it! If you can imagine it, then still do not do it - and then you will get confused just like those scientists. The mirror behaves in exactly the same way in both cases.
If we tried to write a computer program simulating this experiment (and not just predicting the result), it would look something like this ...
At the beginning of the program, we declare a variable that stores in itself a specific mathematical object — a configuration . It represents a certain description of the state of the world - in this case, “one photon flies to point A”.
In fact, the configuration is described by a complex number (recall that complex numbers have the form (a + b i ), where a and b are real numbers, and i is an imaginary unit, that is, a number such that i ² = -1) . Our configuration "photon flies to point A " also corresponds to some number. Let it be (-1 + 0 i ). In the following, we will call the number corresponding to the configuration its amplitude .
We introduce two more configurations: “the photon flies from A to point B ” and “the photon flies from A to point C ”. We do not yet know the amplitudes of these configurations; they will be assigned values during program execution.

The amplitudes can be calculated by applying the rule that the mirror operates to the initial configuration. Without going into details, we can assume that the rule looks like this: “multiply by 1 when the photon flies by; multiply by i when the photon is reflected. ” Apply the rule: the amplitude of the configuration “photon flies to B ” equals (-1 + 0 i ) × i = (0 + - i ), and the amplitude of the configuration “photon flies to C ” equals (-1 + 0 i ) × 1 = ( -1 + 0 i ). Other configurations in fig. 1 no, so we're done.
In principle, we can consider “the first detector registers a photon” and “the second detector registers a photon” as separate configurations, but this does not change anything; their amplitudes will be equal to the amplitudes of the two previous configurations, respectively. (In fact , they still need to be multiplied by a factor equal to the distance from A to the detectors, but we simply assume that all distances in our experiment are unit multipliers.)
So, here is the final state of the program:
- "Photon flies to A ": (-1 + 0 i )
- "Photon flies from A to B ": (0 + - i )
- "Photon flies from A to C ": (-1 + 0 i )
And, perhaps:
- "The first detector worked": (0 + - i )
- "The second detector worked": (-1 + 0 i )
Of course, no matter how many times we run the program, the final state will remain the same.
Now, for rather complex reasons, which I won't go into yet, there is no easy way to measure the amplitude of the configuration. The state of the program is hidden from us.
What to do?
Although we cannot measure amplitude directly, we have something - namely, a magic measuring thing that can tell us the square of the magnitude module of the configuration. In other words, for amplitude (a + b i ), the thing will answer with a number (a² + b²).
It would be more accurate to say that the magic thing finds only the ratio of the squares of the modules to each other. But even this information is enough to understand what is happening inside the program and according to what laws it works.
With the help of the gizmo, we can easily find out that the squares of the modules of the configurations “triggered the first detector” and “triggered the second detector” are equal. And having conducted some more complex experiments, we can also find out the ratio of the amplitudes themselves - i to 1.
By the way, what is this magic measuring thing like that?
Well, when such experiments are carried out in real life, the fact that the experiment is carried out a couple of thousand times serves as a magic thing and they simply count how many times the photon is in the first detector and how many in the second. The ratio of these values will be the ratio of the squares of the amplitude modules. Why it will be so - the question is different, much more complicated. In the meantime, you can use the thing and without understanding how yes why it works. Everything has its time.
You may ask: “But why do we need quantum theory at all, if its predictions coincide with the predictions of the billiard theory?” There are two reasons. First, the reality , whatever you think, is still subject to the quantum laws - amplitudes, complex numbers, and so on. And secondly, the "billiard" theory does not work for any more or less complex experiment. Want an example? You are welcome.
In fig. 2 you can see two mirrors at points B and C , and two half-mirrors at points A and D. Later I will explain why the segment DE is drawn by a dotted line; on the calculations it does not affect.

Let's apply the rules we already know.
At the beginning, we have the configuration “photon flies to A ”, its amplitude is (-1 + 0 i ).
We consider the amplitudes of the configurations "photon flies from A to B " and "photon flies from A to C ":
- "Photon flies from A to B " = i × "photon flies to A " = (0 + - i )
- "Photon flies from A to C " = 1 × "photon flies to A " = (-1 + 0 i )
It is intuitively clear that an ordinary mirror behaves like a half-mirror: it always reflects a photon, it always multiplies the amplitude by i . So:
- "Photon flies from B to D " = i × "photon flies from A to B " = (1 + 0 i )
- "Photon flies from C to D " = i × "photon flies from A to C " = (0 + - i )
It is important to understand that “from B to D ” and “from C to D ” are two different configurations. You can't just write “photon flies to D ”, because what happens next will depend on the angle at which this photon comes in D.
We consider further:
- the amplitude of the configuration “photon flies from B to D ”, equal to (1 + 0 i ):
- multiplied by i , and the result (0 + i ) is counted in favor of the configuration “photon flies from D to E ”
- multiplied by 1, and the result (1 + 0 i ) is counted in favor of the configuration “photon flies from D to F ”
- the amplitude of the configuration “photon flies from C to D ”, equal to (0 + - i ):
- multiplied by i , and the result (1 + 0 i ) counts in favor of the configuration “photon flies from D to F ”
- multiplied by 1, and the result (0 + - i ) is counted in favor of the configuration "photon flies from D to E "
Total:
- "Photon flies from D to E " = (0 + i ) + (0 + - i ) = (0 + 0 i ) = 0
- "Photon flies from D to F " = (1 + 0 i ) + (1 + 0 i ) = (2 + 0 i )
The ratio of the squares of the amplitude modules is 0 to 4; it follows from the calculations that the first detector will not work at all ! Therefore, the segment DE was drawn by a dotted line in Fig. 2

If the half-mirrors had reflected or passed the photon randomly, both detectors would react with approximately the same frequency. But this does not coincide with the results of the experiments. That's all.
You could argue: “And that's not all! Suppose, for example, that when a mirror reflects a photon, something happens to it that the second time it does not reflect? Conversely, when the mirror misses the photon, the next time it will have to reflect. "
First, Occam's razor. There is no need to invent a complex explanation, if there is already a simple (if, of course, we consider quantum mechanics simple ...) And secondly, I can come up with another experience that will refute this alternative theory.
Place a small opaque object between B and D so that the amplitude of the “photon flies from B to D ” configuration is always zero.

Now the amplitude of the “photon flies from D to F ” configuration is (1 + 0 i ), and the amplitude of the “photon flies from D to E ” configuration is (0 + - i ). The squares of the modules are equal to 1. This means that in half of the cases the first detector will be triggered, and in half the second.
It is impossible to explain, if we assume that the photon is a small billiard ball, which is reflected from the mirrors.
The fact is that amplitude cannot be thought of as probability. In probability theory, if an event X can occur or not occur, then the probability of an event Z is equal to P ( Z | X ) P ( X ) + P ( Z | ¬ X ) P (¬ X ), where all the probabilities are positive. If you know that the probability of Z , provided that X has happened, is 0.5, and the probability of X is 0.3, then the total probability of Z is at least 0.15, regardless of what happens if X does not happen. There are no negative probabilities. Possible and impossible events cannot cancel each other. And the amplitudes - can.

Here is an example of wrong thinking: “A photon flies to B or C , but it could fly differently, and this affects the likelihood that it will fly to E ...”
Events that did not happen have no influence on the world. The only thing that can affect the world is our imagination. "Oh my God, this car almost hit me," you think, and decide to go to the monastery, never to meet with dangerous cars. But the real thing is still not the event itself , but only your imagination contained in your brain - which can be pulled out of you, felt and put back in order to make sure that it is quite real.
Really everything that affects the world. (If you think this is not the case, try defining the word “real.”) Configurations and amplitudes directly affect the world, so they are also real. To say that a configuration is “what could have happened” is as strange as saying that a chair is “what could have happened.”
What is this configuration then?
To be continued.
In fact, everything is a little more complicated than you might have thought after reading this article.
Each configuration describes all the particles in the universe. Amplitude is a continuous distribution over the entire configuration space, and not a discrete one, as we have considered today. Indeed, photons do not teleport from one place to another instantly , and each different state of the world is described by a new configuration. In the end we will get to that.
If you do not understand anything of this paragraph, do not worry, I will explain everything. Then.
read the second part →
Author: Eliezer Yudkowsky. Free and abbreviated (quite a bit) translation: me. Links to originals:
lesswrong.com/lw/pc/quantum_explanations lesswrong.com/lw/pc/quantum_explanations , lesswrong.com/lw/pd/configurations_and_amplitude lesswrong.com/lw/pd/configurations_and_amplitude .Source: https://habr.com/ru/post/171489/
All Articles