Finger topology
Topology - quite a beautiful, sonorous word, very popular in some non-mathematical circles, interested me in the 9th grade. Of course, I did not have an exact idea, however, I suspected that everything was tied to geometry.
What is topology ? I must say at once that there are at least two terms “Topology” - one of them simply denotes a certain mathematical structure, the second one carries the whole science behind it. This science consists in the study of the properties of an object that will not change upon its deformation.
')

We see that the circle is transformed into a donut by continuous deformations (in common, the “two-dimensional torus”). It was observed that the topology studies what remains unchanged under such deformations. In this case, the number of “holes” in the object remains unchanged - it is one. For now, let's leave it as it is, a little later we'll figure it out for sure)

Continuous deformations of a person (see picture) can unravel fingers - a fact. Not immediately obvious, but you can guess. And if our topological person prudently put the watch on one hand, then our task will become impossible.
So, I hope a couple of examples brought some clarity to what is happening.
Let's try to formalize it all childishly.
We assume that we are working with clay figures, and plasticine can stretch, compress, while gluing different points and gaps is prohibited . Figures that are translated into each other by continuous deformations described earlier are called homeomorphic.

A very useful case is a sphere with handles. A sphere can have 0 handles - then it’s just a sphere, maybe one - then it’s a bagel (in common “a two-dimensional torus”), etc.
So why is a sphere with handles separated from other figures? Everything is very simple - any shape is homeomorphic to a sphere with some pens. That is, in fact, we do not have anything else. O_o Be it a cup, a spoon, a fork (spoon = fork!), A computer mouse, a man.
This is such a substantive theorem proved. Not us and not now. More precisely, it is proven for a much more general situation. Let me explain: we were limited to the consideration of figures molded from clay and without cavities. This causes the following troubles:
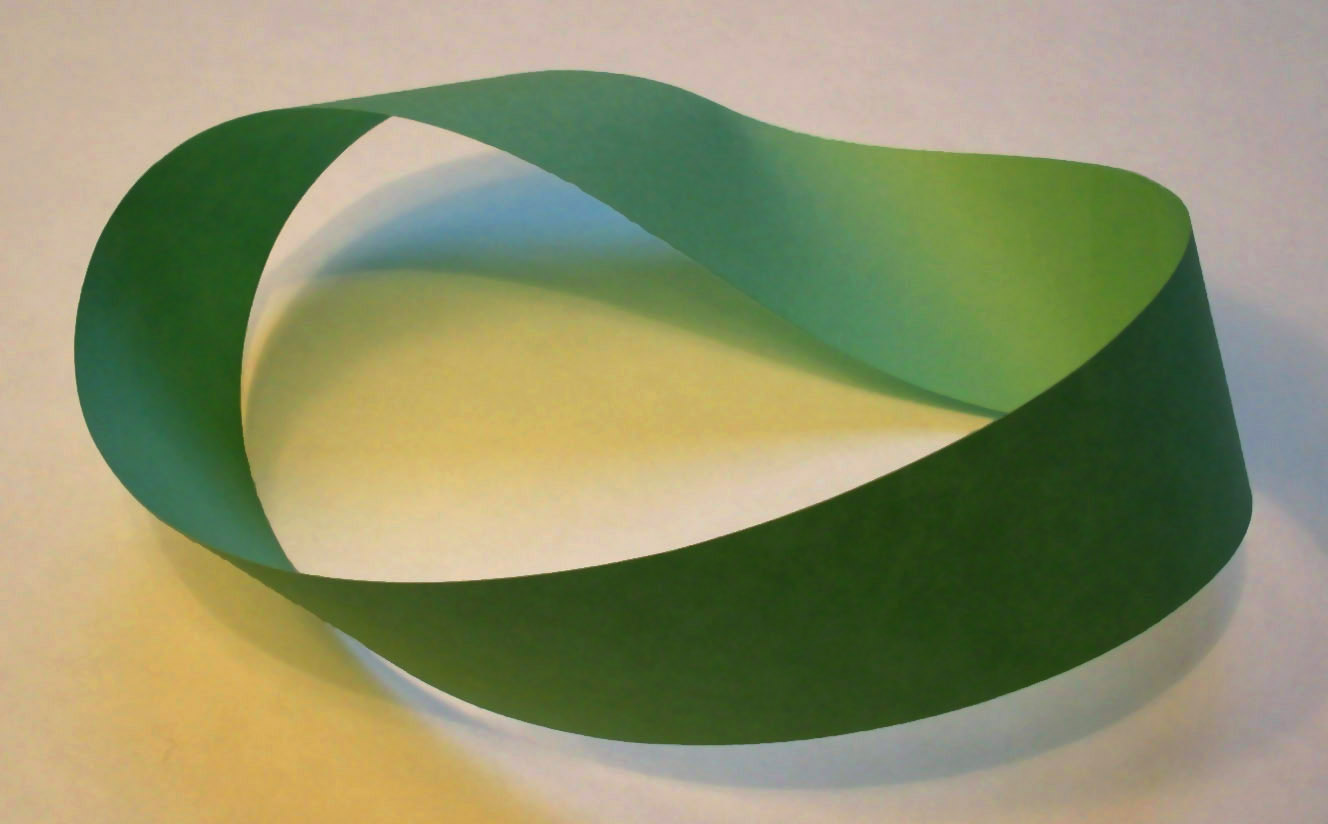
1) we can’t get a non-orientable surface (Klein bottle, Mobius band, projective plane),
2) we confine ourselves to two-dimensional surfaces (n / a: a sphere is a two-dimensional surface),
3) we can not get the surface, the figures stretching to infinity (you can of course imagine this, but there is not enough clay).

Comment: depicts a self-intersection that we forbade initially. In fact, if we consider embedding in four-dimensional space, then we can get rid of self-intersections, but “More Than Three-Dimensional” spaces are unattractive.
Non-orientable surfaces are remarkable in that they do not divide space into two parts, for example, because sphere.
But it is not all that bad. More precisely, everything is as good as in the orientable case - any non-orientable two-dimensional surface (correctly added: compact, connected, without an edge) is homeomorphic to a sphere with a certain number of “Mobius films” and handles. By the way, the Klein bottle is a sphere with two Möbius films.
We familiarized ourselves with the simplest statement of the essence of continuous mappings (deformations), implicitly formulated a classification theorem for two-dimensional compact connected surfaces without an edge, familiarized ourselves with the concept of orientability.
Next, we will talk about the connection of topology with other branches of mathematics / mechanics, I will try to find some highlights.
Words and text were selected in such a way that everything was “intuitively clear.” As a result - the complete absence of mathematical literacy.
What is topology ? I must say at once that there are at least two terms “Topology” - one of them simply denotes a certain mathematical structure, the second one carries the whole science behind it. This science consists in the study of the properties of an object that will not change upon its deformation.
')
Illustrative example 1. Cup bagel.

We see that the circle is transformed into a donut by continuous deformations (in common, the “two-dimensional torus”). It was observed that the topology studies what remains unchanged under such deformations. In this case, the number of “holes” in the object remains unchanged - it is one. For now, let's leave it as it is, a little later we'll figure it out for sure)
A good example 2. Topological people.

Continuous deformations of a person (see picture) can unravel fingers - a fact. Not immediately obvious, but you can guess. And if our topological person prudently put the watch on one hand, then our task will become impossible.
Let's be clear
So, I hope a couple of examples brought some clarity to what is happening.
Let's try to formalize it all childishly.
We assume that we are working with clay figures, and plasticine can stretch, compress, while gluing different points and gaps is prohibited . Figures that are translated into each other by continuous deformations described earlier are called homeomorphic.

A very useful case is a sphere with handles. A sphere can have 0 handles - then it’s just a sphere, maybe one - then it’s a bagel (in common “a two-dimensional torus”), etc.
So why is a sphere with handles separated from other figures? Everything is very simple - any shape is homeomorphic to a sphere with some pens. That is, in fact, we do not have anything else. O_o Be it a cup, a spoon, a fork (spoon = fork!), A computer mouse, a man.
This is such a substantive theorem proved. Not us and not now. More precisely, it is proven for a much more general situation. Let me explain: we were limited to the consideration of figures molded from clay and without cavities. This causes the following troubles:
1) we can’t get a non-orientable surface (Klein bottle, Mobius band, projective plane),
2) we confine ourselves to two-dimensional surfaces (n / a: a sphere is a two-dimensional surface),
3) we can not get the surface, the figures stretching to infinity (you can of course imagine this, but there is not enough clay).
The Mobius strip

Bottle of klein

Comment: depicts a self-intersection that we forbade initially. In fact, if we consider embedding in four-dimensional space, then we can get rid of self-intersections, but “More Than Three-Dimensional” spaces are unattractive.
Non-orientable surfaces are remarkable in that they do not divide space into two parts, for example, because sphere.
But it is not all that bad. More precisely, everything is as good as in the orientable case - any non-orientable two-dimensional surface (correctly added: compact, connected, without an edge) is homeomorphic to a sphere with a certain number of “Mobius films” and handles. By the way, the Klein bottle is a sphere with two Möbius films.
Subtotal
We familiarized ourselves with the simplest statement of the essence of continuous mappings (deformations), implicitly formulated a classification theorem for two-dimensional compact connected surfaces without an edge, familiarized ourselves with the concept of orientability.
Next, we will talk about the connection of topology with other branches of mathematics / mechanics, I will try to find some highlights.
Source: https://habr.com/ru/post/168133/
All Articles