Black-Scholes model: a formula that changed the stock market
It is surprising how much human existence depends on mathematics, if one simple formula can create a global financial crisis.
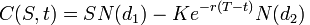
The BBC has published an article about a formula that changed the stock market and was to blame for the current financial crisis. This is the Black-Scholes model , which is used to evaluate derivatives and equity capital of financial companies.
')
The mathematical model of Black-Scholes, introduced in the 70s, gave birth to a new financial system based on options, futures and derivatives trading. In this new system there was nothing from the old classic stock markets. The phenomenal success and widespread formula led to the fact that Myron Scholes received the Nobel Prize in Economics in 1997 "for a new method for determining the value of derivative securities."
Generally speaking, the first futures began to be used in trading in the 17th century, on the Japanese rice exchange. Traders then began to conclude futures transactions, that is, to set the price of the goods, the delivery of which will take place in the future.
By the 20th century, not only futures, but also options were in use on American commodity exchanges - the same price agreement for the future, but without the obligation to make a purchase. Options bought for "insurance" from a sharp increase in prices. Over time, traders have a desire to resell these options, which was difficult, because no one could answer the question: how much are these securities?
For example, how much does an annual option to buy rice cost $ 100 at the beginning of the year, when rice costs $ 90, or one month before the expiration of the option, when rice costs $ 90? Unknown. This is where the revolutionary Black-Scholes model came into being, which takes into account market volatility.
Call option price:
Where
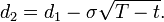
The price of a put option:
- the current value of the call option at time t before the expiration of the option;
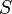- The current price of the underlying stock;
- the probability that the deviation will be less in the conditions of the standard normal distribution;
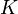- option exercise price;
- risk-free interest rate;
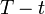- time before the expiration of the option (the period of the option);
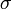- volatility (square root of the variance) of the underlying stock.
Stanford University finance professor Myron Scholes has been passionate about finances since childhood. While still small, he persuaded mom to open an account so that he could trade in the stock market. At 27, he got a position at MIT and, together with his colleague Fisher Black (Fischer Black), seriously took up the puzzle of pricing options. As already mentioned, the key to the solution was to take into account, directly in the formula, the limit of market volatility.
Mayron Shoulzm says that after a year and a half of working on the formula, they saw elements of options in all the objects of the surrounding world.
To the surprise of the authors themselves, the Black-Scholes model began to be used everywhere: in 2007 the volume of trade in derivatives in the world exceeded 1 square trillion dollars, which is ten times the value of goods produced in the entire history of human civilization. What it all ended is well known.
Unforeseen changes in market volatility led to unpleasant consequences for financial markets. Now, some experts call this mathematical model a “dangerous invention,” which unnecessarily simplified such a complex thing as asset valuation. The 1998 crisis showed that a strong change in volatility happens more often than expected, and therefore all assets will have to be overestimated with new coefficients. Roughly speaking, the bloated bubble of the global economy needs to be shriveled back, even if it even means a long recession for developed countries. And all because of an overly simplified mathematical model.
By the way, the hedge fund of Knowles Long-Term Capital Management collapsed back in September 1998, less than a year after he received the Nobel Prize. The fund lost four billion dollars in Russia by joining the GKO financial pyramid that the Russian government built.
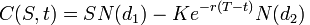
The BBC has published an article about a formula that changed the stock market and was to blame for the current financial crisis. This is the Black-Scholes model , which is used to evaluate derivatives and equity capital of financial companies.
')
The mathematical model of Black-Scholes, introduced in the 70s, gave birth to a new financial system based on options, futures and derivatives trading. In this new system there was nothing from the old classic stock markets. The phenomenal success and widespread formula led to the fact that Myron Scholes received the Nobel Prize in Economics in 1997 "for a new method for determining the value of derivative securities."
Generally speaking, the first futures began to be used in trading in the 17th century, on the Japanese rice exchange. Traders then began to conclude futures transactions, that is, to set the price of the goods, the delivery of which will take place in the future.
By the 20th century, not only futures, but also options were in use on American commodity exchanges - the same price agreement for the future, but without the obligation to make a purchase. Options bought for "insurance" from a sharp increase in prices. Over time, traders have a desire to resell these options, which was difficult, because no one could answer the question: how much are these securities?
For example, how much does an annual option to buy rice cost $ 100 at the beginning of the year, when rice costs $ 90, or one month before the expiration of the option, when rice costs $ 90? Unknown. This is where the revolutionary Black-Scholes model came into being, which takes into account market volatility.
Call option price:
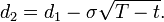
WhereThe price of a put option:
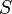- the current value of the call option at time t before the expiration of the option; - The current price of the underlying stock; - the probability that the deviation will be less in the conditions of the standard normal distribution;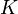 - option exercise price; - risk-free interest rate;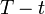 - time before the expiration of the option (the period of the option); 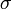- volatility (square root of the variance) of the underlying stock.Stanford University finance professor Myron Scholes has been passionate about finances since childhood. While still small, he persuaded mom to open an account so that he could trade in the stock market. At 27, he got a position at MIT and, together with his colleague Fisher Black (Fischer Black), seriously took up the puzzle of pricing options. As already mentioned, the key to the solution was to take into account, directly in the formula, the limit of market volatility.
Mayron Shoulzm says that after a year and a half of working on the formula, they saw elements of options in all the objects of the surrounding world.
To the surprise of the authors themselves, the Black-Scholes model began to be used everywhere: in 2007 the volume of trade in derivatives in the world exceeded 1 square trillion dollars, which is ten times the value of goods produced in the entire history of human civilization. What it all ended is well known.
Unforeseen changes in market volatility led to unpleasant consequences for financial markets. Now, some experts call this mathematical model a “dangerous invention,” which unnecessarily simplified such a complex thing as asset valuation. The 1998 crisis showed that a strong change in volatility happens more often than expected, and therefore all assets will have to be overestimated with new coefficients. Roughly speaking, the bloated bubble of the global economy needs to be shriveled back, even if it even means a long recession for developed countries. And all because of an overly simplified mathematical model.
By the way, the hedge fund of Knowles Long-Term Capital Management collapsed back in September 1998, less than a year after he received the Nobel Prize. The fund lost four billion dollars in Russia by joining the GKO financial pyramid that the Russian government built.
Source: https://habr.com/ru/post/143059/
All Articles