Workshop on using Schuhart control charts
Recently, I published here my slidecast with a story about 6-sigma, Shewhart control charts and snowflake people , where in a fairly simple language, sometimes abusing foul language, under the 20-minute laughter of students listened to how to separate system variations from variations caused by special reasons .
Now I want to analyze in detail the example of building a Shewhart control map based on real data. As real data, I took historical information about completed personal tasks. I have this information thanks to the adaptation of David Allen’s personal effectiveness model Getting Things (I also have an old slidecast in three parts: Part 1 , Part 2 , Part 3 + Excel-tablet with macros for analyzing tasks from Outlook ).
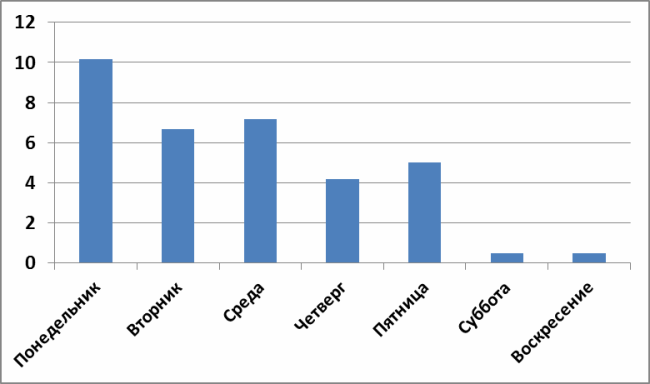
Task setting looks like this. I have a distribution of the average number of completed tasks depending on the day of the week (below on the chart) and I need to answer the question: “is there anything special on Mondays or is it just an error of the system?”
')
We will answer this question with the help of the Shewhart control card - the main tool of statistical process control.
So, the Shewhart criterion for the presence of a particular cause of variation is quite simple: if a point goes beyond the control limits calculated in a special way, then it indicates a particular cause. If the point lies within these limits, then the deviation is due to the general properties of the system itself. Roughly speaking, is the measurement error.
The formula for calculating the control limits is as follows:

Where
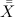 - the average value of the average values for the subgroup,
- the average value of the average values for the subgroup,
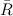 - medium span,
- medium span,
 - some engineering coefficient depending on the size of the subgroup.
- some engineering coefficient depending on the size of the subgroup.
All formulas and tabular coefficients can be found, for example, in GOST 50779.42-99 , where the approach to statistical management is briefly and clearly set out (honestly, I did not expect that there is such a GOST. The topic of statistical management and its place in business optimization is described in more detail in book D. Wheeler ).
In our case, we group the number of completed tasks by day of the week — this will be the subgroups of our sample. I took the data on the number of completed tasks for 5 weeks of work, that is, the size of the subgroup is equal to 5. Using table 2 of the GOST, we find the value of the engineering coefficient:
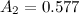
Calculating the average value and scope (the difference between the minimum and maximum values) for the subgroup (in our case for the day of the week) is quite simple, in my case the results are as follows:
The central line of the control card will be the average of group averages, that is:
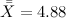
We also calculate the average span:
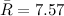
Now we know that the lower control limit for the number of completed tasks will be equal to:
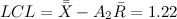
That is, those days in which I complete a smaller number of tasks on average are very special from the point of view of the system.
Similarly, we obtain the upper control limit:
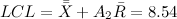
Now plot the center line (red), the upper control limit (green) and the lower control limit (purple):
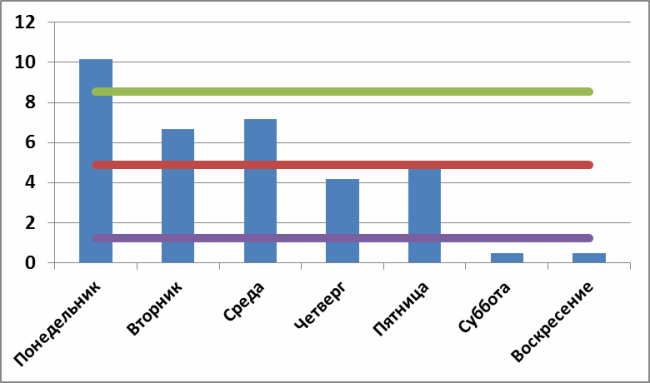
And, oh, a miracle! We see three clearly distinct groups that go beyond the control limits, in which there are clearly not systemic reasons for the variations!
I do not work on Saturdays and Sundays. Fact. And Monday turned out to be a really special day. And now you can think and look for what is so really special on Mondays.
However, if the average number of tasks performed on Monday were within the control limits and even stood out strongly against the rest of the points, then from the point of view of Shewhart and Deming, it would be senseless to look for some particular features on Mondays, since such behavior is due solely to common reasons . For example, I built a control card for another 5 weeks at the end of last year:
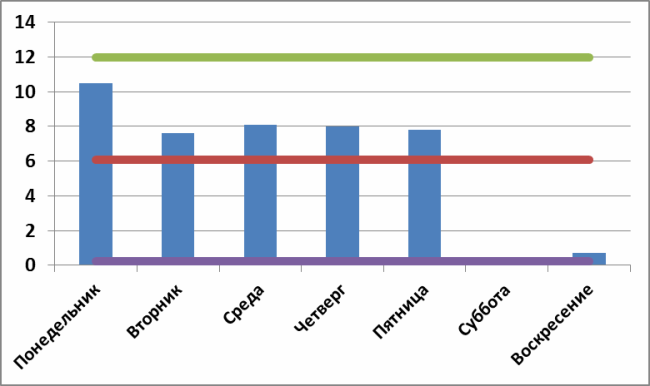
And it seems like there is some feeling that Monday somehow stands out, but according to Shewhart's criterion, this is only a fluctuation or an error in the system itself. According to Schuhart, in this case, it is possible to investigate the special reasons of Mondays for an arbitrarily long time - they simply do not exist. From the point of view of the statistical office, on this data, Monday is no different from any other working day (even Sundays).
Now I want to analyze in detail the example of building a Shewhart control map based on real data. As real data, I took historical information about completed personal tasks. I have this information thanks to the adaptation of David Allen’s personal effectiveness model Getting Things (I also have an old slidecast in three parts: Part 1 , Part 2 , Part 3 + Excel-tablet with macros for analyzing tasks from Outlook ).
Task setting looks like this. I have a distribution of the average number of completed tasks depending on the day of the week (below on the chart) and I need to answer the question: “is there anything special on Mondays or is it just an error of the system?”
')

We will answer this question with the help of the Shewhart control card - the main tool of statistical process control.
So, the Shewhart criterion for the presence of a particular cause of variation is quite simple: if a point goes beyond the control limits calculated in a special way, then it indicates a particular cause. If the point lies within these limits, then the deviation is due to the general properties of the system itself. Roughly speaking, is the measurement error.
The formula for calculating the control limits is as follows:

Where
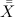 - the average value of the average values for the subgroup,
- the average value of the average values for the subgroup,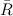 - medium span,
- medium span, - some engineering coefficient depending on the size of the subgroup.
- some engineering coefficient depending on the size of the subgroup.All formulas and tabular coefficients can be found, for example, in GOST 50779.42-99 , where the approach to statistical management is briefly and clearly set out (honestly, I did not expect that there is such a GOST. The topic of statistical management and its place in business optimization is described in more detail in book D. Wheeler ).
In our case, we group the number of completed tasks by day of the week — this will be the subgroups of our sample. I took the data on the number of completed tasks for 5 weeks of work, that is, the size of the subgroup is equal to 5. Using table 2 of the GOST, we find the value of the engineering coefficient:
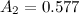
Calculating the average value and scope (the difference between the minimum and maximum values) for the subgroup (in our case for the day of the week) is quite simple, in my case the results are as follows:
| Day of the week | Group average | Swipe |
|---|---|---|
| Monday | 10.2 | eight |
| Tuesday | 6.7 | ten |
| Wednesday | 7.2 | eleven |
| Thursday | 4.2 | 9 |
| Friday | 5.0 | ten |
| Saturday | 0.5 | 2 |
| Sunday | 0.5 | 3 |
The central line of the control card will be the average of group averages, that is:
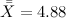
We also calculate the average span:
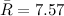
Now we know that the lower control limit for the number of completed tasks will be equal to:
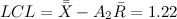
That is, those days in which I complete a smaller number of tasks on average are very special from the point of view of the system.
Similarly, we obtain the upper control limit:
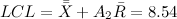
Now plot the center line (red), the upper control limit (green) and the lower control limit (purple):

And, oh, a miracle! We see three clearly distinct groups that go beyond the control limits, in which there are clearly not systemic reasons for the variations!
I do not work on Saturdays and Sundays. Fact. And Monday turned out to be a really special day. And now you can think and look for what is so really special on Mondays.
However, if the average number of tasks performed on Monday were within the control limits and even stood out strongly against the rest of the points, then from the point of view of Shewhart and Deming, it would be senseless to look for some particular features on Mondays, since such behavior is due solely to common reasons . For example, I built a control card for another 5 weeks at the end of last year:

And it seems like there is some feeling that Monday somehow stands out, but according to Shewhart's criterion, this is only a fluctuation or an error in the system itself. According to Schuhart, in this case, it is possible to investigate the special reasons of Mondays for an arbitrarily long time - they simply do not exist. From the point of view of the statistical office, on this data, Monday is no different from any other working day (even Sundays).
Source: https://habr.com/ru/post/139596/
All Articles