Doppler effect in networks: GSM, 3G, 4G
After reading this article, I almost flew off the chair and did not eat my radio engineering diploma. To clarify the situation and calm down the fermenters, I decided to write about the Doppler effect in more detail.
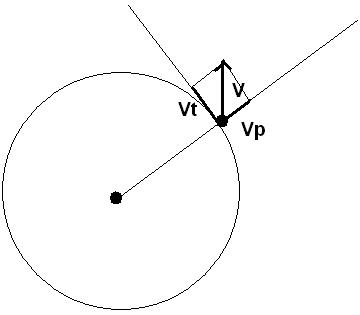
Let's start with the basics. The Doppler effect is observed only in the presence of a parallel component of the velocity of motion, with a tangential motion of the Doppler frequency shift will not be (at least at up to relativistic speeds).
In the above formula:
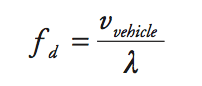
Vp speed will be featured. As it is easy to see, if the body moves in a circle, then the Doppler effect will not be generally observed. On this occasion there are very good illustrations on wikipedia.

Since most people are more accustomed to working with frequency, I will allow myself to perform some obvious transformations on the Doppler effect formula. We translate the wavelength into frequency, and also take into account the direction angle to the base station:

But let's continue to consider the case when our body moves away (or approaching) from the BS in a straight line. In this case, the calculations from the first article will be correct:
Oh! What is it? The speeds are equal, and the frequencies are different. In the heat of distrust of the author recalculate. The result is 34 * 2.5E9 / 3E8 = 283.3 (3) Hz. It is this value that will change the frequency of the signal: increase if the body comes closer or decreases if the body is removed. Also note that when the direction of movement changes, this frequency will change, but never will be more than 283.3 (3) Hz, since cos (x) is never greater than 1. With the Doppler effect, everything seems to be all. We received all the data we needed.
Now let's turn to such a thing as a radio.
GSM and 3G networks use phase shift keying with robust coding. What is important for us is that this phase shift keying (further PSK). Any PSK signal, in addition to the standard set of parameters, is characterized by such a thing as phase inflorescence. Here I will give an inflorescence for the most common type of PSK, namely QPSK:

More detailed information on what phase manipulation is available on Wikipedia.
To receive such signals, synchronous or digital detectors are used, which require the exact value of the reference frequency for their work (for more information on this topic, see the books on receiving and processing signals or in the specification for developing dial-up modems). Since there is nothing ideal in our world, such types of receivers basically cannot work without the AFC system (auto frequency control). Characteristics of such a system can be seen in the passport to the receiver in columns: capture band. Let's turn to the standard. According to the standard, the BS frequency instability in the GSM system is about 10E – 6 ... 10E – 7, which means that the capture bandwidth of the AFC should be twice (at least) wider than this value and be 500..5000 Hz. In addition, there is such a thing as a stock, and the value of this parameter is clearly greater. We see that this value is clearly greater than 283.3 (3) Hz, which indicates the ability of the AFC system to fully compensate for the Doppler effect at such speeds and even large ones.
For those who are not gullible, as an argument, I suggest reading the article in a very respected IEEE magazine.
Let's start with the basics. The Doppler effect is observed only in the presence of a parallel component of the velocity of motion, with a tangential motion of the Doppler frequency shift will not be (at least at up to relativistic speeds).

In the above formula:
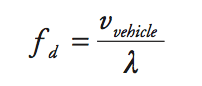
Vp speed will be featured. As it is easy to see, if the body moves in a circle, then the Doppler effect will not be generally observed. On this occasion there are very good illustrations on wikipedia.


Since most people are more accustomed to working with frequency, I will allow myself to perform some obvious transformations on the Doppler effect formula. We translate the wavelength into frequency, and also take into account the direction angle to the base station:

But let's continue to consider the case when our body moves away (or approaching) from the BS in a straight line. In this case, the calculations from the first article will be correct:
Here c is the speed of light, which for simplicity we take equal to 3x10 ^ 8 and f is the frequency of the signal: 2.5 GHz
Thus, the wavelength is 3x10 ^ 8 / 2.5x10 ^ 9 = 0.12 (m)
For a vehicle moving at a speed of 122 km / h (34 m / s), the Doppler frequency change will be 34 / 0.12 = 283 (Hz)
')
That is, when the subscriber moves relative to the base station at a speed of 122 km / h, the frequency of the signal for him will change to 272 Hz.
Oh! What is it? The speeds are equal, and the frequencies are different. In the heat of distrust of the author recalculate. The result is 34 * 2.5E9 / 3E8 = 283.3 (3) Hz. It is this value that will change the frequency of the signal: increase if the body comes closer or decreases if the body is removed. Also note that when the direction of movement changes, this frequency will change, but never will be more than 283.3 (3) Hz, since cos (x) is never greater than 1. With the Doppler effect, everything seems to be all. We received all the data we needed.
Now let's turn to such a thing as a radio.
GSM and 3G networks use phase shift keying with robust coding. What is important for us is that this phase shift keying (further PSK). Any PSK signal, in addition to the standard set of parameters, is characterized by such a thing as phase inflorescence. Here I will give an inflorescence for the most common type of PSK, namely QPSK:

More detailed information on what phase manipulation is available on Wikipedia.
To receive such signals, synchronous or digital detectors are used, which require the exact value of the reference frequency for their work (for more information on this topic, see the books on receiving and processing signals or in the specification for developing dial-up modems). Since there is nothing ideal in our world, such types of receivers basically cannot work without the AFC system (auto frequency control). Characteristics of such a system can be seen in the passport to the receiver in columns: capture band. Let's turn to the standard. According to the standard, the BS frequency instability in the GSM system is about 10E – 6 ... 10E – 7, which means that the capture bandwidth of the AFC should be twice (at least) wider than this value and be 500..5000 Hz. In addition, there is such a thing as a stock, and the value of this parameter is clearly greater. We see that this value is clearly greater than 283.3 (3) Hz, which indicates the ability of the AFC system to fully compensate for the Doppler effect at such speeds and even large ones.
For those who are not gullible, as an argument, I suggest reading the article in a very respected IEEE magazine.
Source: https://habr.com/ru/post/131248/
All Articles